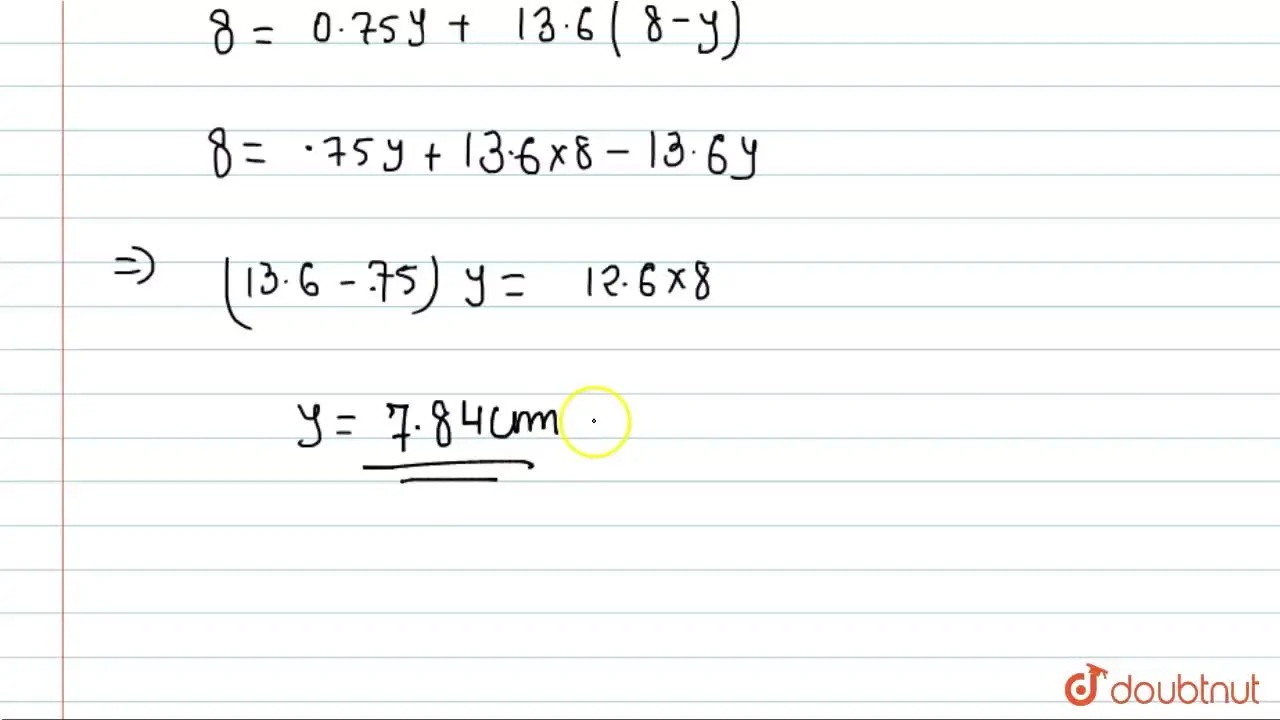The arms of a vertical U tube of uniform inner cross section contains mercury. In one of the arms of the tube, water column of length 8 cm is introduced. In the other arm, oil of density 0.75 g/cm3 is poured till the upper surfaces of water and oil are at same horizontal level. Calculate the length of oilcolumn.

As A and B are at same levels
PA=PB
(8−h)×13.6×g+h×0.75×g=8×1×g
8×13.6−13.6×h+h×0.75=8
100.8=−h×0.75+13.6h
=h(0.75+13.6)
h=7.84 cm
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Competition File (A. MCQ (Objectie Type Questions))20videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Competition File (B. MCQ (AIPMT/NEET & Other State Boards For Medical Entrance))6videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Revision Exercise (Long Answer Questions)7videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MATHEMATICAL TOOLSEXERCISE - PRACTICE PROBLEMS (10)12videos
MATHEMATICAL TOOLS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
Similar Questions
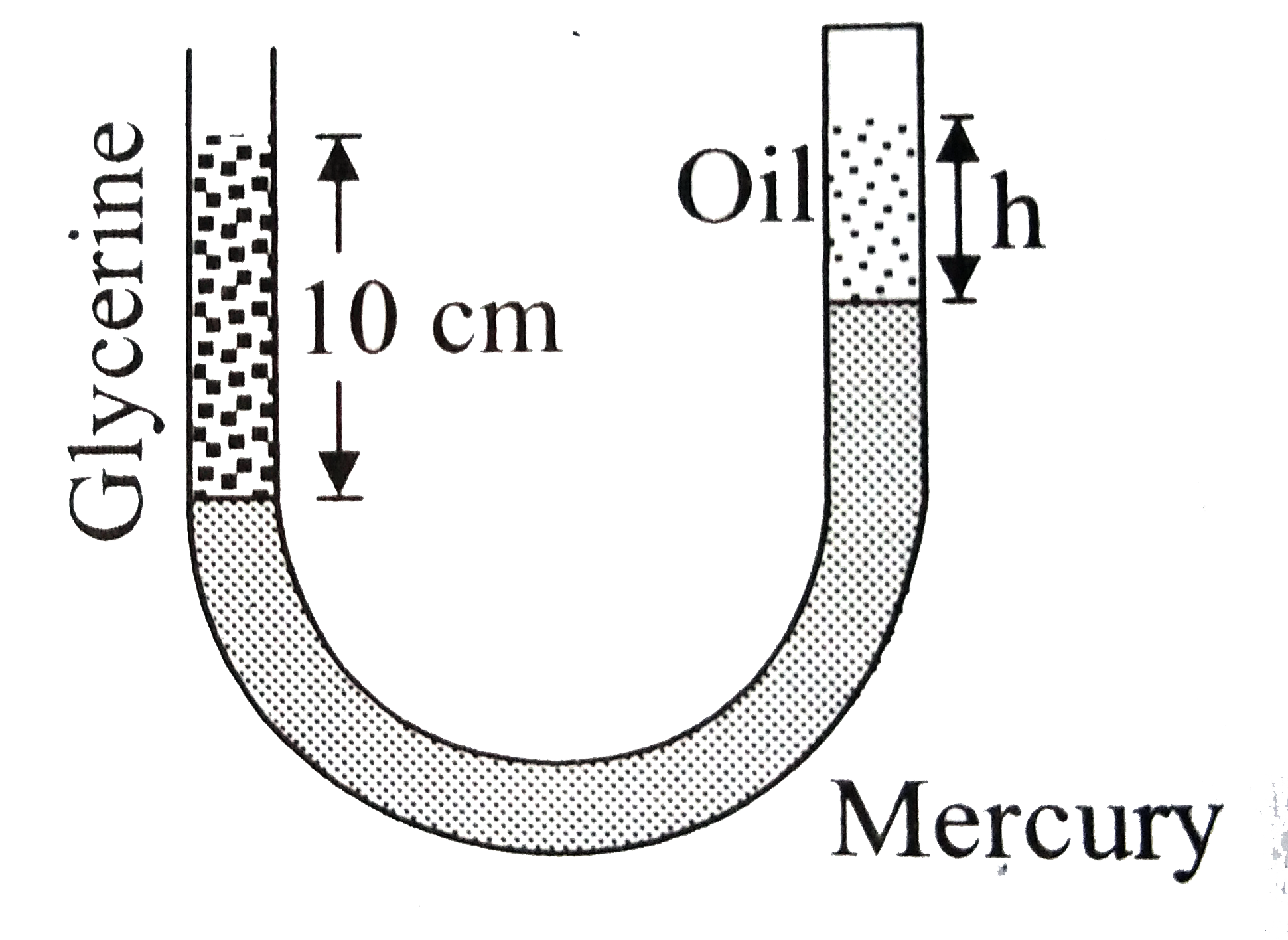
A vertical U tube of uniform cross section contains mercury in both of its arms. A glycerine (d=1.3g/cm3) column of length 10cm is introduced into one of the arms. Oil of density 0.8g/cm3 is poured in the other arm until the upper surfaces of the oil and glycerine are in the same horizontal level. Find the length of oil column. Densit of mercury is 13.6g/cm3.
 View Solution
View SolutionA vertical U-tube of uniform inner cross-section contains mercury in both of its arms. A glycerine (density1.3gcm−3) column of length 10cmis introduced into one of the arms Oil of density 0.8gcm−3 is poured in the other arm until the upper surface of the oil and glycerine are in the same horizontal level. Find the length of the oil column.
View Solution_S01_015_Q01.png)
A vertical U-tube of uniform cross-section contains mercury in both arms A glycerine (relative density =1.3) column of length 10 cm is introduced into one of the arms. Oil of density 800 gm m−3 is poured into the other arm until the upper surface of the oil and hlycerine are at the same horizontal level, find the length of the column. Density of mercury is 13.6×103kgm−3View SolutionA vertical U-tube of uniform inner cross section contains mercury in both sides of its arms. A glycerin (density =1.3g/cm3) column of length 10 cm is introduced into one of its arms. Oil of density 0.8 gm/cm3 is poured into the other arm until the upper surfaces of the oil and glycerin are in the same horizontal level. Find the length of the same horizontal level. Find the length of the oil column, Density of mercury =13.6g/cm3
 View Solution
View SolutionAn open U-tube contains mercury. When 11.2 cm of water is poured into one of the arms of the tube, how high does the mercury rise in the other arm from its initial level ?
View SolutionA vertical U - tube of uniform cross - section contains water in both the arms. A 10 cm glycerine column (R.D. = 1.2) is added to one of the limbs. What is the level difference between the two free surface in the two limbs?
View SolutionA vertical U-tube of uniform cross-section contains water jn both the arms. A 10 cm glycerine column (R.D. = 1.2) is added to one of the limbs. The level difference between the two free surfaces in the two limbs wiil be
View SolutionA vertical U-tube of uniform cross-section contains water upto a height of 30 cm. show that if the water in one limb is depressed and then released, its up and down motion in the two limbs of the tube is simple harmonic and calculate its time-period.
View SolutionA vertical U-tube of uniform cross-section contains water upto a height of 30 cm. show that if the water in one limb is depressed and then released, its up and down motion in the two limbs of the tube is simple harmonic and calculate its time-period.
View SolutionA vertical U-tube of uniform cross-section contains water upto a height of 2.45 cm. show that if the water on one side is decpressed and then released, its up and down motion in the tube is SHM and calculate its time period. Given g=980cms−2.
View Solution