Two small spherical metal balls, having equal masses, are made from materials of densities and have radii 1 mm and 2mm respectively, they are made to fall vertically (from rest) in a viscous medium whose coefficient of viscosity equal and whose density is 0.1 . The ratio of their terminal velocities would be
The correct Answer is:A
Step by step video, text & image solution for Two small spherical metal balls, having equal masses, are made from materials of densities rho_(1) and rho_(2) (rho_(1)=8rho_(2)) and have radii 1 mm and 2mm respectively, they are made to fall vertically (from rest) in a viscous medium whose coefficient of viscosity equal eta and whose density is 0.1 rho_(2). The ratio of their terminal velocities would be by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Competition File (JEE (Main) & Other State Boards For Engineering Entrance)23videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Competition File (JEE (Advanced) For IIT Entrance)14videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Competition File (A. MCQ (Objectie Type Questions))20videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MATHEMATICAL TOOLSEXERCISE - PRACTICE PROBLEMS (10)12videos
MATHEMATICAL TOOLS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
Similar Questions
Two circular discs A and B have equal masses and uniform thickness but have densities ρ1 and ρ2 such that ρ1>ρ2 . Their moments of inertia is
View SolutionThe escape velocities of the two planets, of densities ρ1 and ρ2 and having same radius, are v1 and v2 respectively. Then
View SolutionTwo wires of equal diameters, of resistivities ρ1 and ρ2 and length l1 and l2, respectively of the combination is
View SolutionEqual masses of two substance of densities ρ1andρ2 are mixed together. What is the density of the mixture?
View SolutionTwo discs have same mass and thickness. Their materials are of densities ρ1 and ρ2 . The ratio of their moment of inertia about central axis will be
View SolutionTwo disc has same mass rotates about the same axis with their densities ρ1 and ρ2 respectively such that (ρ1>ρ2), then the relation between I1 and I2 will be
View SolutionThe light cone is in equilibrium under the action of hydrostatic forces of two liquids of densities ρ1 and ρ2. Find ρ1/ρ2. Find ρ1 and ρ2.
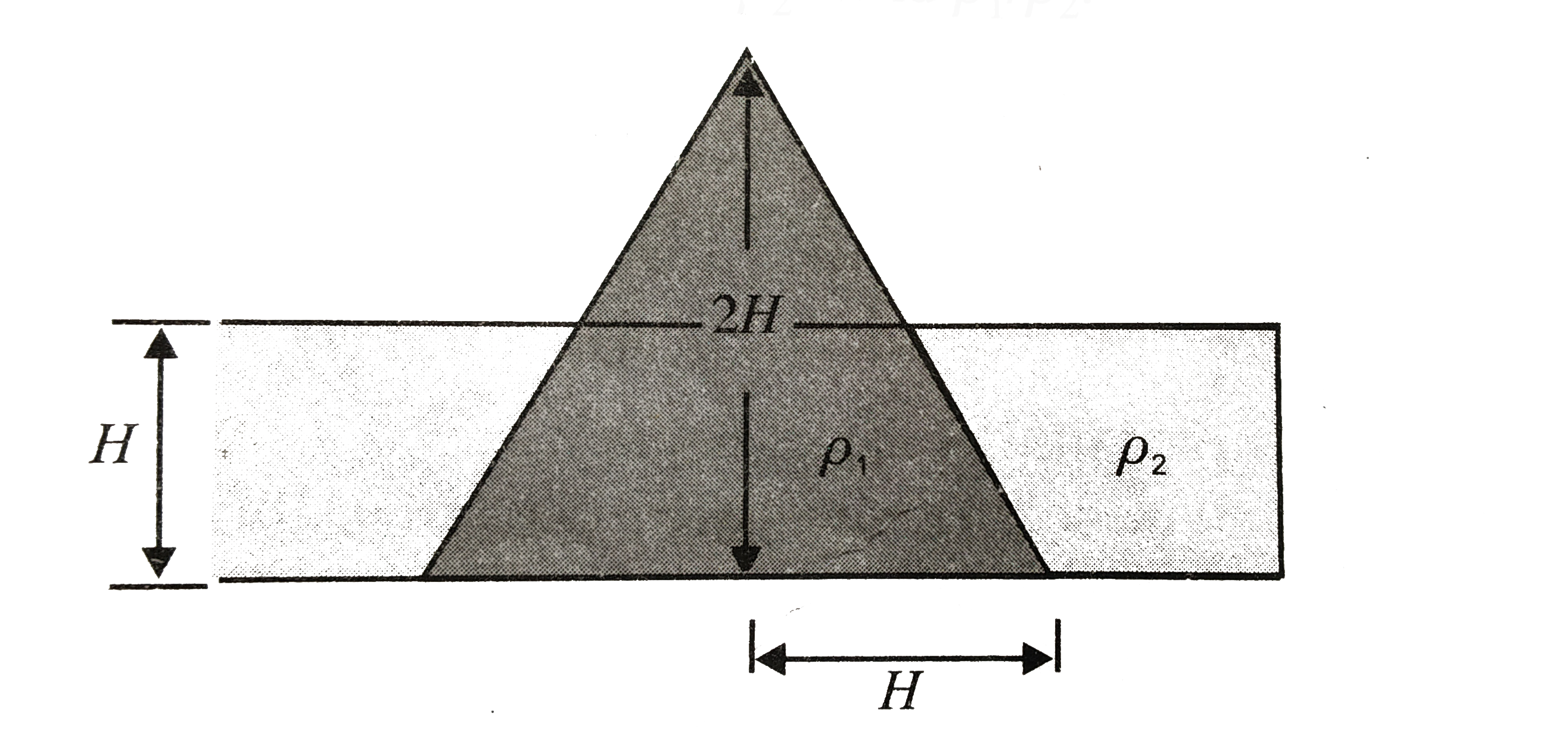 View Solution
View SolutionTwo wires of equal diameters of resistivies ρ1 and ρ2 and lengths l and l respectively are joined in series. The equivalent resistivity of the combination is
View SolutionTwo circular disc of same mass and thickness are made from metals having densities ρ1 and ρ2 respectively. The ratio of their moment of inertia about an axis passing through its centre is,
View SolutionTwo solid spheres are made up of the same material of density ρ. The ratio of their radii is 1:2. The ratio of their moments of inertia about their respective diameters is
View Solution


