A circle is drawn in a sectore of a larger circle of radius r, as shown in fugure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the smaller circle is
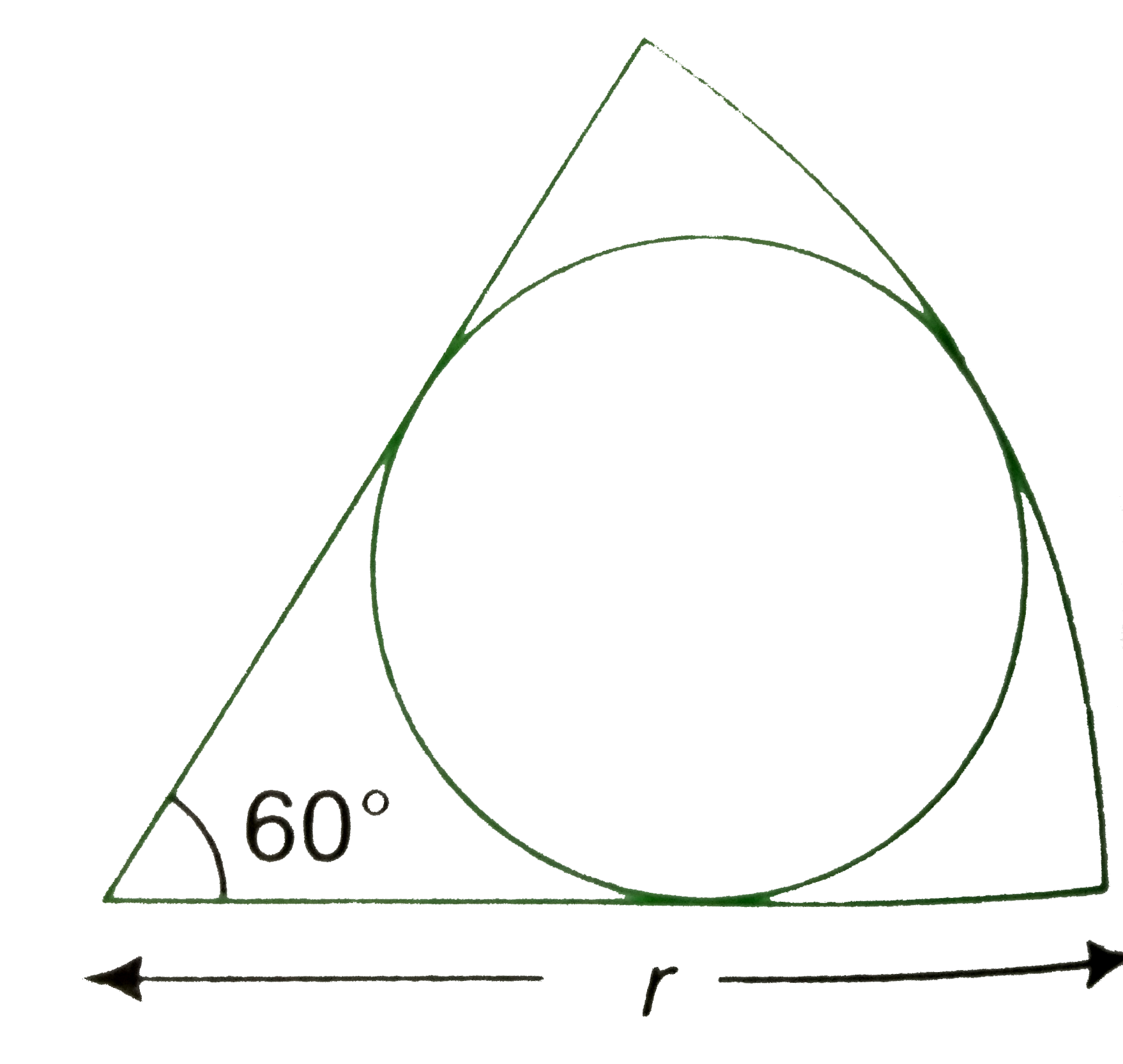
r2
r3
2√3r5
r√2
The correct Answer is:A
Let the radius of smaller circle be x. Here,
OP=xcosec30∘
andOQ=r=xcosec30∘
⇒=x=r3

Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Multiple)
17 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Comprehension)
11 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.6
9 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Videos
Similar Questions
A circle with radius 2 is placed against a right angle. A smaller circle is also placed as shown in the adjoining figure. The radius of the smaller circle is:
View SolutionO is the centre of the smaller circle, O' is the centre of bigger circle.CD is tangent to the smaller circle,Find the radius of the smaller circle.
View Solution
Knowledge Check
A circle is drawn in a sector of a larger circle of radius r, as shown in the adjacent figure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the small circle is-
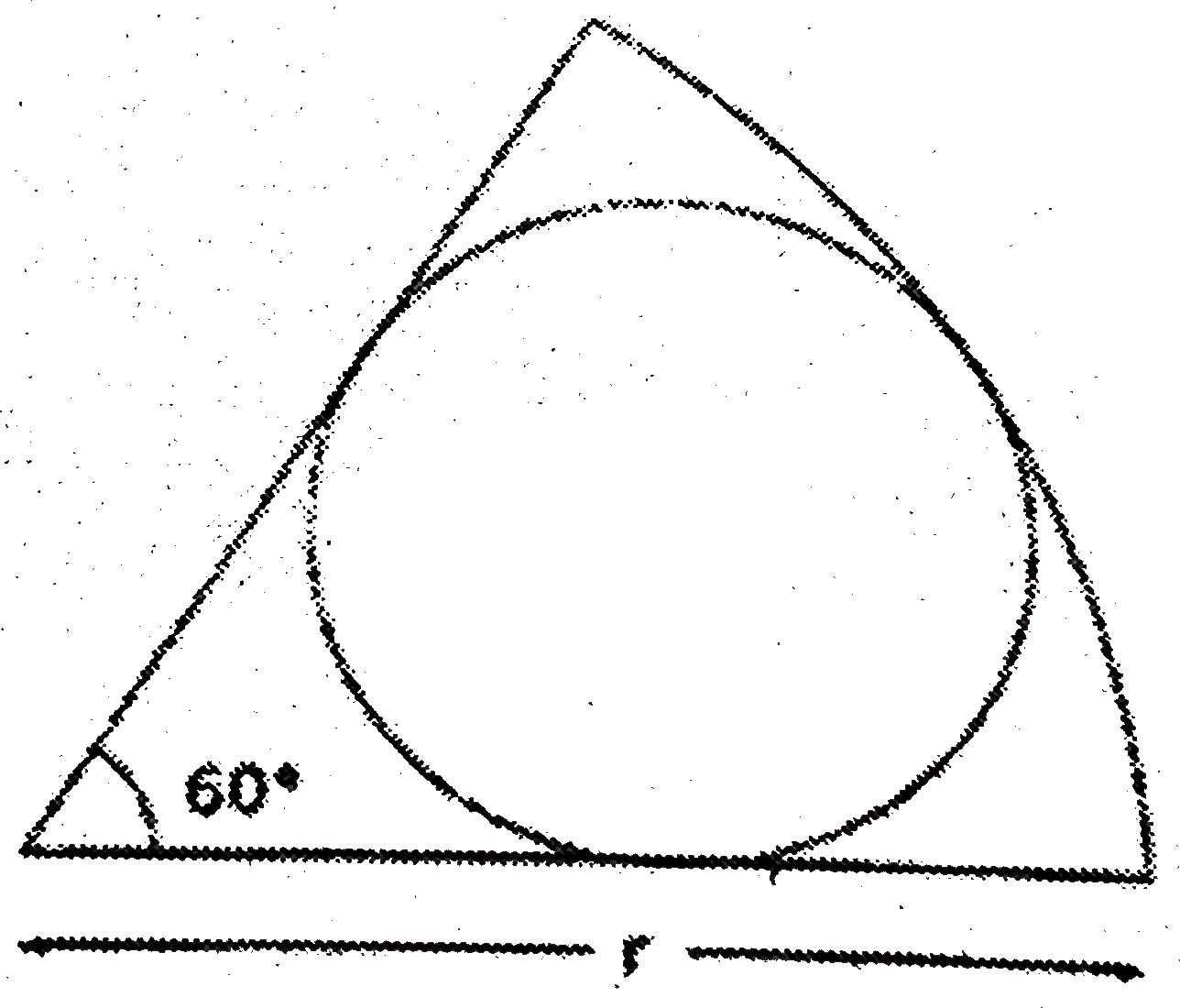 Ar2Br3C2√3r5Dr√2
Ar2Br3C2√3r5Dr√2If θ is the angle (in degrees) of a sector of a circle of radius r, then area of sector is
Aθ360×πr2Bθ180×πr2Cθ360×2πrDθ180×2πrTwo circles touch each other externally. The distance between their centres is 8 cm. If the radius of one circle is 3 cm, then the radius of the other circle is:
दो वृत्त एक दूसरे को बाह्य रूप से स्पर्श करते हैं। उनके केंद्रों के बीच की दूरी 8 सेमी है। यदि एक वृत्त की त्रिज्या 3 सेमी है, तो दूसरे वृत्त की त्रिज्या है:A7.5 cmB9 cmC8 cmD10 cm
The adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.
View SolutionA smaller circle touches internally to a larger circle at A and passes through the centre of the larger circle. O is the centre of the larger circle and BA, OA are of the diameters of the larger and smaller circles respectively. Chord AC intersects the smaller circle at a point D. If AC=12cm, then AD is :
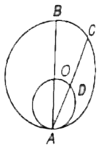
View SolutionIn two concentric circles, a chord of length 8cm of the larger circle touches the smaller circle. If the radius of the larger circle is 5cm then find the radius of the smaller circle.
View SolutionThe area of a sector of a circle is 77 sq cm and the angle of the sector is 45∘. Find the radius of the circle.
View SolutionCircumference of a sector of angle p∘ of a circle with radius R is
View Solution
