The adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.
Here, radius of larger circle, R=10 cm
Radius of smaller circle, r=4 cm
(a) ∴ Area of larger circle, A=πR2=3.14∗10∗10=314cm2
(b)Area of smaller circle a=πr2=3.14∗4∗4=50.24cm2
(c) Area of shaded region =A−a=314−50.24=263.76cm2
Topper's Solved these Questions
- PERIMETER AND AREABOOK - NCERTCHAPTER - PERIMETER AND AREAEXERCISE - EXERCISE 11.2
8 Videos
- PERIMETER AND AREABOOK - NCERTCHAPTER - PERIMETER AND AREAEXERCISE - EXERCISE 11.4
8 Videos
- LINES AND ANGLESBOOK - NCERTCHAPTER - LINES AND ANGLESEXERCISE - SOLVED EXAMPLES
1 Video
- RATIONAL NUMBERSBOOK - NCERTCHAPTER - RATIONAL NUMBERSEXERCISE - SOLVED EXAMPLES
6 Videos
Similar Questions
The adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.
View SolutionIn two concentric circles, a chord of length 8cm of the larger circle touches the smaller circle. If the radius of the larger circle is 5cm then find the radius of the smaller circle.
View Solution
Knowledge Check
In the following figure, three circles are given. The two smaller circles are equal. The radius of the larger circle is 8 cm. O is the centre or the larger circle.
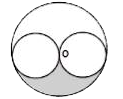
Find area of shaded portion.A16πB20πC4πD8πThe difference between the radii of the smaller circle and the bigger circle is 7 cm and the difference between the areas of the two circles is 1078 sq cm. What is the radius of the smaller circle in cm?
A28B21C17.5D35From a point Q, the length of the tangent to a circle is 12 cm and the distance of Q from the centre is 13 cm. The radius of the circle is 13 cm. The radius of the circle is :
A12 cmB13 cmC5 cmDNone of these
The sum of circumferences of two circles is 132 cm. If the radius of one circles is 14 cm, find the radius of the other circle.
View SolutionIn the given figure,diameter of larger semi-circle is 14 cm and radius of smaller semi-circle is 3.5cm. find :
View SolutionTwo circles touch each other at point X. Two common tangents of the circles meet at point P and none of the tangents passes through X. These tangents touch the larger circle at points B and C. If the radius of the larger circle is 15 cm and CP = 20 cm, then what is the radius (in cm) of the smaller circle?
View SolutionThe figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given ∠APB=a∘, Calculate in terms of a∘, the value of
View SolutionTwo circles have an external tangent with length 36 cm. The shortest distance between these circles is 14 cm. If the radius of the longer circle is 4 times the radius of the smaller circle then the radius of the larger circle in cms is
View Solution
