A conducting ring of radius 2 metre is placed in an uniform magnetic field B of 0.01 Tesla oscillating with frequency 200 Hz with its plane at right angles to B . What will be the induced electric field ?
4 volts/m
6 volts /m
10 volts/m
8 volts/m
The correct Answer is:D
To solve the problem of finding the induced electric field in a conducting ring placed in a uniform magnetic field, we can follow these steps:
Step 1: Understand the Problem
We have a conducting ring with a radius R=2 meters placed in a uniform magnetic field B=0.01 Tesla. The magnetic field is oscillating with a frequency f=200 Hz, and the plane of the ring is perpendicular to the magnetic field.
Step 2: Calculate the Area of the Ring
The area A of the ring can be calculated using the formula for the area of a circle:
A=πR2
Substituting the value of R:
A=π(2)2=4πm2
Step 3: Calculate the Time Period of Oscillation
The time period T of the oscillation can be calculated using the frequency:
T=1f=1200s=0.005s
Step 4: Calculate the Change in Magnetic Flux
The magnetic flux Φ through the ring is given by:
Φ=B⋅A
Since the magnetic field is oscillating, we need to consider the change in flux over time. The maximum change in flux occurs when the angle θ changes from 0 to 90 degrees.
At t=0:
Φ0=B⋅A=0.01⋅4π=0.04πWb
At t=T4 (where θ=90∘):
Φ=0Wb
Thus, the change in flux ΔΦ is:
ΔΦ=Φ−Φ0=0−0.04π=−0.04πWb
Step 5: Calculate the Induced EMF
According to Faraday's law of electromagnetic induction, the induced EMF E is given by:
E=−dΦdt
The average rate of change of flux over the time period T/4 is:
E=−ΔΦT/4=−−0.04π0.005/4=0.04π⋅40.005=0.16π0.005=32πV
Step 6: Relate Induced EMF to Electric Field
The induced electric field E around the ring can be found using the relationship between EMF and electric field:
E=E⋅(2πR)
Substituting for R=2:
32π=E⋅(2π⋅2)
Simplifying gives:
32π=E⋅4π
Thus,
E=32π4π=8V/m
Final Answer
The induced electric field is:
8V/m
We have a conducting ring with a radius R=2 meters placed in a uniform magnetic field B=0.01 Tesla. The magnetic field is oscillating with a frequency f=200 Hz, and the plane of the ring is perpendicular to the magnetic field.
The area A of the ring can be calculated using the formula for the area of a circle:
A=πR2
Substituting the value of R:
A=π(2)2=4πm2
The time period T of the oscillation can be calculated using the frequency:
T=1f=1200s=0.005s
The magnetic flux Φ through the ring is given by:
Φ=B⋅A
Since the magnetic field is oscillating, we need to consider the change in flux over time. The maximum change in flux occurs when the angle θ changes from 0 to 90 degrees.
Φ0=B⋅A=0.01⋅4π=0.04πWb
At t=T4 (where θ=90∘):
Φ=0Wb
Thus, the change in flux ΔΦ is:
ΔΦ=Φ−Φ0=0−0.04π=−0.04πWb
According to Faraday's law of electromagnetic induction, the induced EMF E is given by:
E=−dΦdt
The average rate of change of flux over the time period T/4 is:
E=−ΔΦT/4=−−0.04π0.005/4=0.04π⋅40.005=0.16π0.005=32πV
The induced electric field E around the ring can be found using the relationship between EMF and electric field:
E=E⋅(2πR)
Substituting for R=2:
32π=E⋅(2π⋅2)
Simplifying gives:
32π=E⋅4π
Thus,
E=32π4π=8V/m
The induced electric field is:
8V/m
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:NARAYNAChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE-2 (C.W)
Explore 22 Videos
- ELECTROMAGNETIC INDUCTIONBook:NARAYNAChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE-2 (H.W)
Explore 16 Videos
- ELECTROMAGNETIC INDUCTIONBook:NARAYNAChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE-1 (C.W)
Explore 58 Videos
- ELECTRO MAGNETIC WAVESBook:NARAYNAChapter:ELECTRO MAGNETIC WAVESExercise:LEVEL-II(H.W)
Explore 14 Videos
- ELECTROMAGNETIC WAVES Book:NARAYNAChapter:ELECTROMAGNETIC WAVES Exercise:EXERCISE -4
Explore 15 Videos
Similar Questions
A conducting ring of radius l m kept in a uniform magnetic field B of 0.01 T, rotates uniformly with an angular velocity 100 rad s–1 with its axis of rotation perpendicular to B. The maximum induced emf in it is
View SolutionA conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
View Solution
Knowledge Check
A Conducting ring of radius 1 meter is placed in an uniform magnetic field B of 0.01 tesla oscillating with frequency 100Hz with its plane at right angles to B. What will be the induced electric field?
Aπ volt /mB2 volt/mC10 volt/mD62 volt/mA conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
AIt is rotated about it axisBit is translatedCit is rotated about a diameterDit is deformedA circular loop of radius R is placed in a uniform magnetic field B=2sinp Tesla as shown. Which of the following is incorrect?
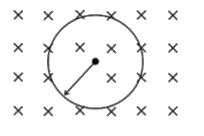 AForce on the loop is zero at t=12 secBForce on the loop is zero at t=32 secCForce on the every element of the loop is radially inward at t=54 secDForce on the every element of the loop is radially inwart at t=74 sec
AForce on the loop is zero at t=12 secBForce on the loop is zero at t=32 secCForce on the every element of the loop is radially inward at t=54 secDForce on the every element of the loop is radially inwart at t=74 sec
A conducting circular loop of radius r carries a constant current i. It is placed in a uniform magnetic field B such that B is perpendicular to the plane of loop. What is the magnetic force acting on the loop?
View SolutionA conducting ring of radius r is placed in a varying magnetic field perpendicular to the plane of the ring. If the rate at which the magnetic field varies is x, the electric field intensity at any point of the ring is
View SolutionMetal ring of radius R is placed perpendicular to uniform magnetic field B. Magnetic field starts changing at a rate α
View SolutionA circular coil of radius r is placed in a uniform magnetic field B. The magnetic field is normal to the plane of the coil is rotated at an angular speed of ω, about its own axis , then the induced emf in the coil is __
View SolutionA conducting circular loop of radius r carries a constant i. It is placed in a uniform magnetic field B such that B is perpendiclar to the plane of thre loop is .What is the magnetic force acting on the loop?
View Solution
