In prove that
To prove that in triangle ABC, where angle A = 60°, the equation BC2=AB2+AC2−AB⋅AC holds true, we can follow these steps:
1. Draw Triangle ABC:
- Begin by sketching triangle ABC with angle A = 60°. Label the sides as follows: let AB=c, AC=b, and BC=a.
2. Use the Cosine Rule:
- According to the cosine rule, for any triangle, the relationship between the sides and angles is given by:
a2=b2+c2−2bc⋅cos(A)
- In our case, since A=60∘, we know that cos(60∘)=12.
3. Substitute the Values:
- Substitute cos(60∘) into the cosine rule:
a2=b2+c2−2bc⋅12
4. Simplify the Equation:
- Simplifying the equation gives:
a2=b2+c2−bc
5. Rearranging the Terms:
- We can rewrite the equation as:
a2=AB2+AC2−AB⋅AC
- Here, a=BC, b=AC, and c=AB.
6. Conclusion:
- Therefore, we have proved that:
BC2=AB2+AC2−AB⋅AC
- Begin by sketching triangle ABC with angle A = 60°. Label the sides as follows: let AB=c, AC=b, and BC=a.
- According to the cosine rule, for any triangle, the relationship between the sides and angles is given by:
a2=b2+c2−2bc⋅cos(A)
- In our case, since A=60∘, we know that cos(60∘)=12.
- Substitute cos(60∘) into the cosine rule:
a2=b2+c2−2bc⋅12
- Simplifying the equation gives:
a2=b2+c2−bc
- We can rewrite the equation as:
a2=AB2+AC2−AB⋅AC
- Here, a=BC, b=AC, and c=AB.
- Therefore, we have proved that:
BC2=AB2+AC2−AB⋅AC
Topper's Solved these Questions
- TRIANGLES Book:NAGEEN PRAKASHANChapter:TRIANGLES Exercise:Revision Exercise Very Short Questions
Explore 9 Videos
- TRIANGLES Book:NAGEEN PRAKASHANChapter:TRIANGLES Exercise:Revision Exercise Short Questions
Explore 10 Videos
- TRIANGLES Book:NAGEEN PRAKASHANChapter:TRIANGLES Exercise:Exercise 6c
Explore 11 Videos
- STATISTICS Book:NAGEEN PRAKASHANChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHANChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Revisions Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
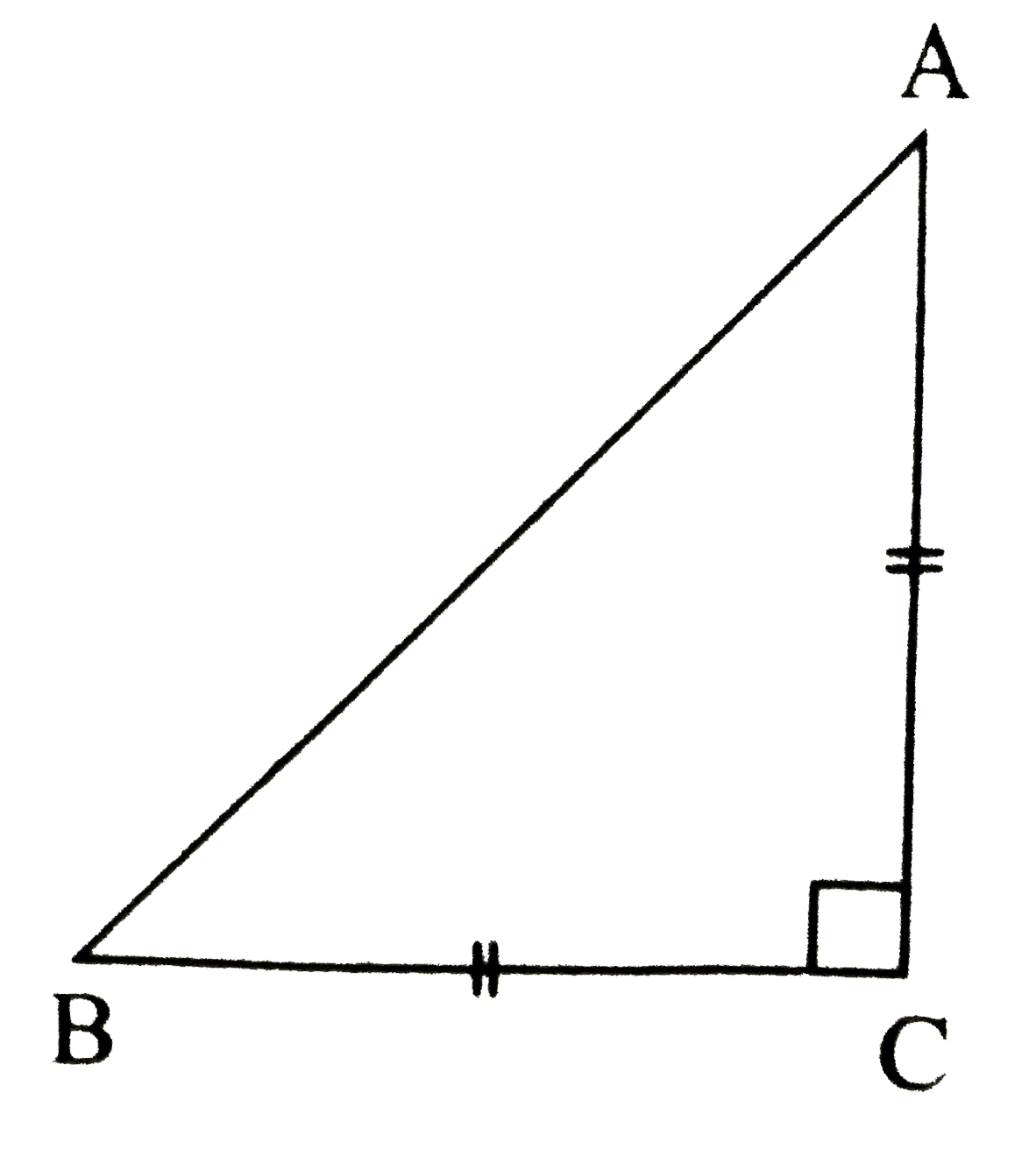
In ΔABC, AC=BC and ∠ACB=90∘ then prove AB2=2AC2
View Solutionin ΔABC,∠A=2∠B Prove that BC2=AC2+AC.AB
View SolutionIn Figure 2,AD⊥BC. Prove that AB2+CD2=BD2+AC2.
View SolutionIn the given figure if AD ⊥ BC prove that AB2+CD2=BD2+AC2
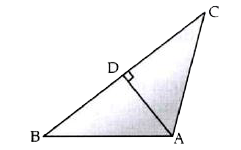
View SolutionIn figure, ∠B<90∘andAD⊥BC. Prove that AC2=AB2+BC2−2BC.BD
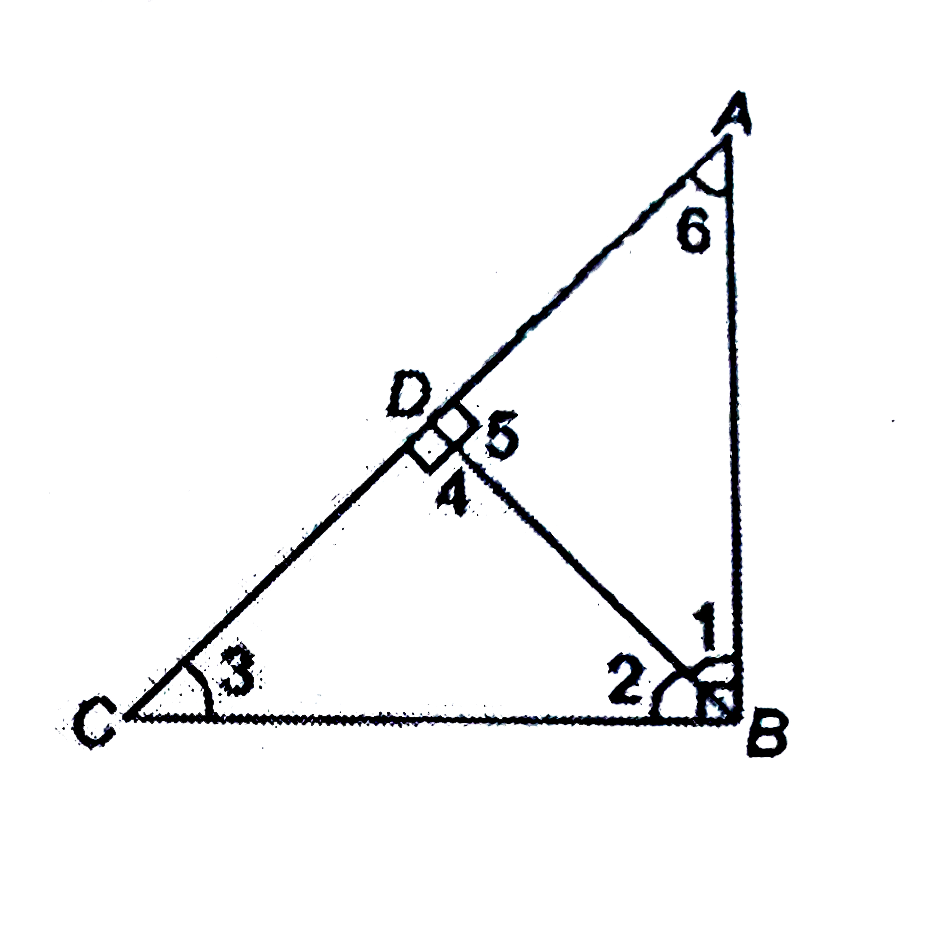
View SolutionIn the given figure if ∠B=90∘ and BD is perpendicular to AC then
prove that:
(i)△ADB~△BDC(ii)△ADB~△ABC
(iii) △BDC~△ABC(iv)BD2=AD×DC
(v) AB2=AD×AC(vi)BC2=CD×AC
(vii) AB2+BC2=AC2
View SolutionIn △ABC,AB=10cm,∠A=45∘ and ∠C=60∘. Find BC.
View Solution△ABC is an isosceles triangle with AC = BC. If AB2=2AC2 . Prove that △ABC is a right triangle.
View SolutionIn ABC , given that AB=AC and BD⊥AC . Prove that BC2=2 ACCD
View SolutionThe perpendicular AD on the base BC of a △ABC intersects BC at D, so that DB=3CD. Prove that 2AB2=2AC2+BC2
View Solution