In a rhombus ABCD, prove that AC2+BD2=4AB2
To prove that in a rhombus ABCD, AC2+BD2=4AB2, we can follow these steps:
Step 1: Understand the Properties of a Rhombus
A rhombus has all sides equal, so we have:
AB=BC=CD=DA
Let AB=a.
Step 2: Diagonals of the Rhombus
The diagonals of a rhombus bisect each other at right angles. Let O be the point of intersection of the diagonals AC and BD. Therefore, we have:
AO=AC2andBO=BD2
Also, since the diagonals intersect at right angles, we have:
∠AOB=90∘
Step 3: Apply the Pythagorean Theorem
In triangle AOB, we can apply the Pythagorean theorem:
AB2=AO2+BO2
Substituting the values of AO and BO:
a2=(AC2)2+(BD2)2
This simplifies to:
a2=AC24+BD24
Step 4: Multiply the Equation by 4
To eliminate the fractions, multiply the entire equation by 4:
4a2=AC2+BD2
Step 5: Conclusion
Since AB=a, we can rewrite the equation as:
AC2+BD2=4AB2
Thus, we have proved that in a rhombus ABCD:
AC2+BD2=4AB2
A rhombus has all sides equal, so we have:
AB=BC=CD=DA
Let AB=a.
The diagonals of a rhombus bisect each other at right angles. Let O be the point of intersection of the diagonals AC and BD. Therefore, we have:
AO=AC2andBO=BD2
Also, since the diagonals intersect at right angles, we have:
∠AOB=90∘
In triangle AOB, we can apply the Pythagorean theorem:
AB2=AO2+BO2
Substituting the values of AO and BO:
a2=(AC2)2+(BD2)2
This simplifies to:
a2=AC24+BD24
To eliminate the fractions, multiply the entire equation by 4:
4a2=AC2+BD2
Since AB=a, we can rewrite the equation as:
AC2+BD2=4AB2
Thus, we have proved that in a rhombus ABCD:
AC2+BD2=4AB2
Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Revision Exercise Very Short Questions
9 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Revision Exercise Short Questions
10 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6c
11 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
For a rhambus ABCD , prove that 4AB2=AC2+BD2
View SolutionIn a rhombus prove that sum of squares of sides is equal to sum of square of diagonals or, In a rhombus ABCD prove that
AB2+BC2+CD2+DA2=AC2+BD2
View SolutionIn rhombus ABCD, show that 4AB2=AC2+BD2
View SolutionIn a ΔABC,∠B is an acute-angle and AD⊥BC
Prove that :
(i) AC2=AB2+BC2−2BC×BD
(ii) AB2+CD2=AC2+BD2
View SolutionIn the given figure if AD ⊥ BC prove that AB2+CD2=BD2+AC2
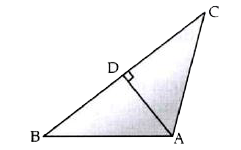
View SolutionABCD is a rhombus. Prove that AB2+BC2+CD2+DA2=AC2+BD2
View SolutionIn a quadrilateral ABCD prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2 where P and Q are middle points of diagonals AC and BD.
View SolutionIn a quadrilateral ABCD, prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2, where P and Q are middle points of diagonals AC and BD.
View SolutionIn any triangle ABC, prove that AB2+AC2=2(AD2+BD2) , where D is the midpoint of BC.
View SolutionIn a right triangle ABC, right angled at A, AD is drawn perpendicular to BC. Prove that:
AB2−BD2=AC2−CD2
View Solution
