Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals.
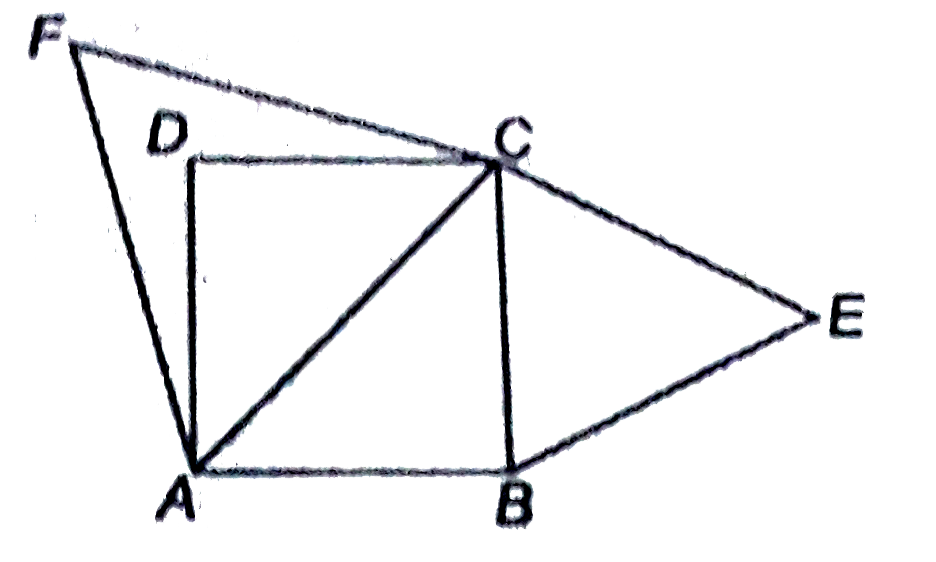
Given: A square ABCD and equilateral △BCEand△ACF have been described on side BC and diagonal AC respectively.
To prove: ar(△BCE)12ar(△ACF)
Proof: Since each of the △BCEand△ACF is an equilateral triangles so each angle of each are of them is 60∘.
Hence △BCE~△ACF
ar(△BCE)ar(△ACF)=BC2AC2=BC22(BC)2=12
Hence, ar(△BCE)12×ar(△ACF)
Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar
14 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a
23 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
Prove that the area of the equilateral triangle described on the side of a square is half the area of the equilateral triangle described on its diagonal.
View SolutionProve that the area of the equilateral triangle described on the side of a square is half the area of the equilateral triangle described on its diagonal.
View Solution
Knowledge Check
What is the area of an equilateral triangle with side 2cm?
A4√3cm2B3√3cm2C6cm2D√3cm2The area of an equilateral triangle of side 20 cm is
A100√3cm2B100√3cm2C100√32cm2D100√34cm2
Prove that the area of equilateral triangle described on the side of a square is half the area of the equilateral triangle described on its diagonal.
View SolutionProve that the area of an equilateral triangle described on a side of a right-angled isosceles triangle is half the area of the equilateral triangle described on its hypotenuse.
View SolutionWhat is the area of an equilateral triangle of side 10 cm?
View SolutionFind the area of an equilateral triangle of side 6 cm.
View SolutionFind the area of an equilateral triangle of side 6 cm.
View SolutionProve that area of an equilateral triangle formed on one side of a given square is one half of area of equilateral triangle formed on diagonal is the same square.
View Solution