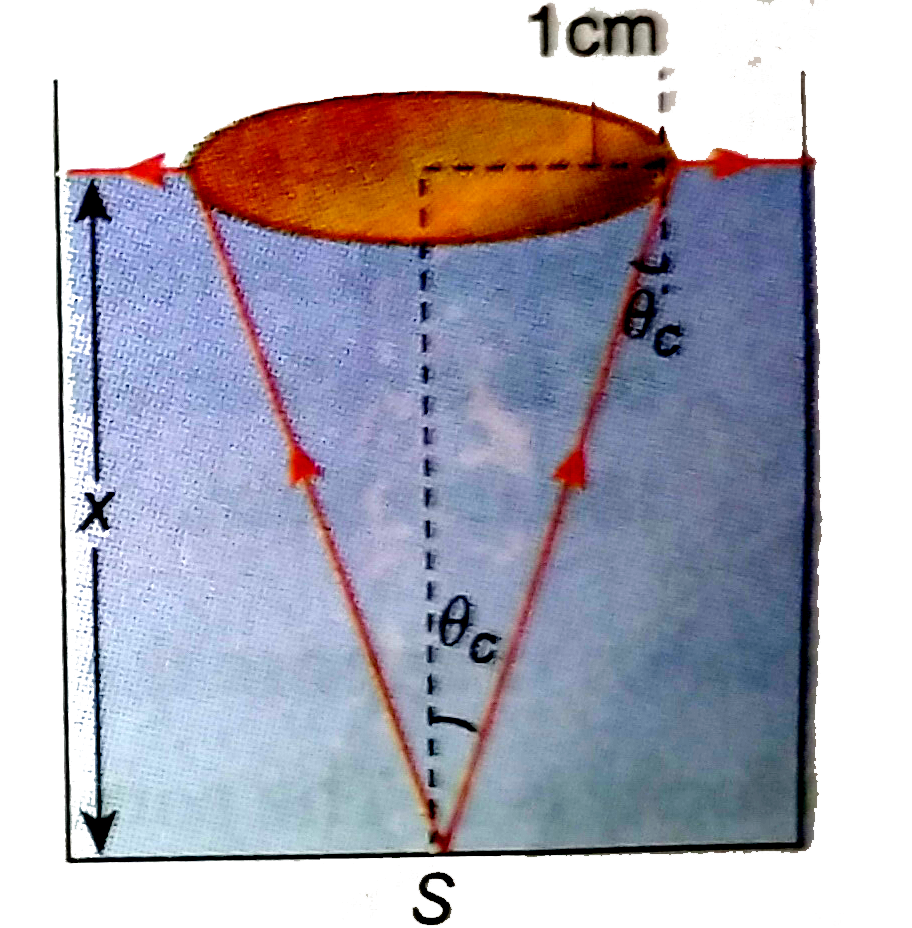
A vessel contains a liquid of refractive index . Inside the liquid, S is a point source which is observed from above the liquid. An opaque disc of radius 1cm is floating on the liquid such that its centre is just above the source. At this circumstances liquid of the vessel is leaving gradually through a hole. What is the depth of the liquid, so that the source no more remains visible from above?
Let x be the required depth obviously, at this position light rays from the source s must be incident at critical angle at the edge of the disc [Fig. 2.70].
Topper's Solved these Questions
- REFRACTION OF LIGHTBook:CHHAYA PUBLICATIONChapter:REFRACTION OF LIGHTExercise:HIGHER ORDER THINKING SKILL (HOTS QUESTION)
Explore 25 Videos
- REFRACTION OF LIGHTBook:CHHAYA PUBLICATIONChapter:REFRACTION OF LIGHTExercise:SECTION RELATED QUESTION
Explore 18 Videos
- REFLECTION OF LIGHTBook:CHHAYA PUBLICATIONChapter:REFLECTION OF LIGHTExercise:INTEGER ANSWER TYPE
Explore 3 Videos
- SEMICONDUCTORS AND ELECTRONICS Book:CHHAYA PUBLICATIONChapter:SEMICONDUCTORS AND ELECTRONICS Exercise:CBSE SCANNER
Explore 31 Videos
Similar Questions
A point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View SolutionA point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View Solution
Knowledge Check
A liquid is kept in a cylindrical vessel which is rotated along its axis. The liquid rises at the side. If the radius of the vessel is 5cm and the speed of rotation is 4rev/s, then the difference in the height of the liquid at the centre of the vessel and its sides is
A8cmB2cmC40cmD4cm
A point source of light S is placed at the bottom of a vessel containing a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of disc lies vertically above the source O. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot be seen at all.
View SolutionThe apparent depth of a liquid in a vessel is 15 cm, when its real depth is 20 cm. find the refractive index of the liquid.
View SolutionA liquid is kept in a cylindrical vessel which is rotated along its axis. The liquid rises at the side. If the radius of the vessel is 5cm and the speed of rotation is 4rev/s, then the difference in the height of the liquid at the centre of the vessel and its sides is
View SolutionA liquid is kept in a cylindrical vessel which is rotated along its axis. The liquid rises at the side. If the radius of the vessel is 5cm and the speed of rotation is 4rev/s, then the difference in the height of the liquid at the centre of the vessel and its sides is
View SolutionA disc is placed on the surface of pond filled with liquid of refractive index 53. A source of light is placed 4m below the surface of liquid. Calculate the minimum area of the disc so that light does not come out of liquid.
View SolutionA disc is placed on the surface of pond filled with liquid of refractive index 53. A source of light is placed 4m below the surface of liquid. Calculate the minimum area of the disc so that light does not come out of liquid.
View SolutionThe apparent depth of an object at the bottom of tank filled with a liquid of refractive index 1.3 is 7.7 cm. what is the actual depth of the liquid in the tank ?
View Solution
