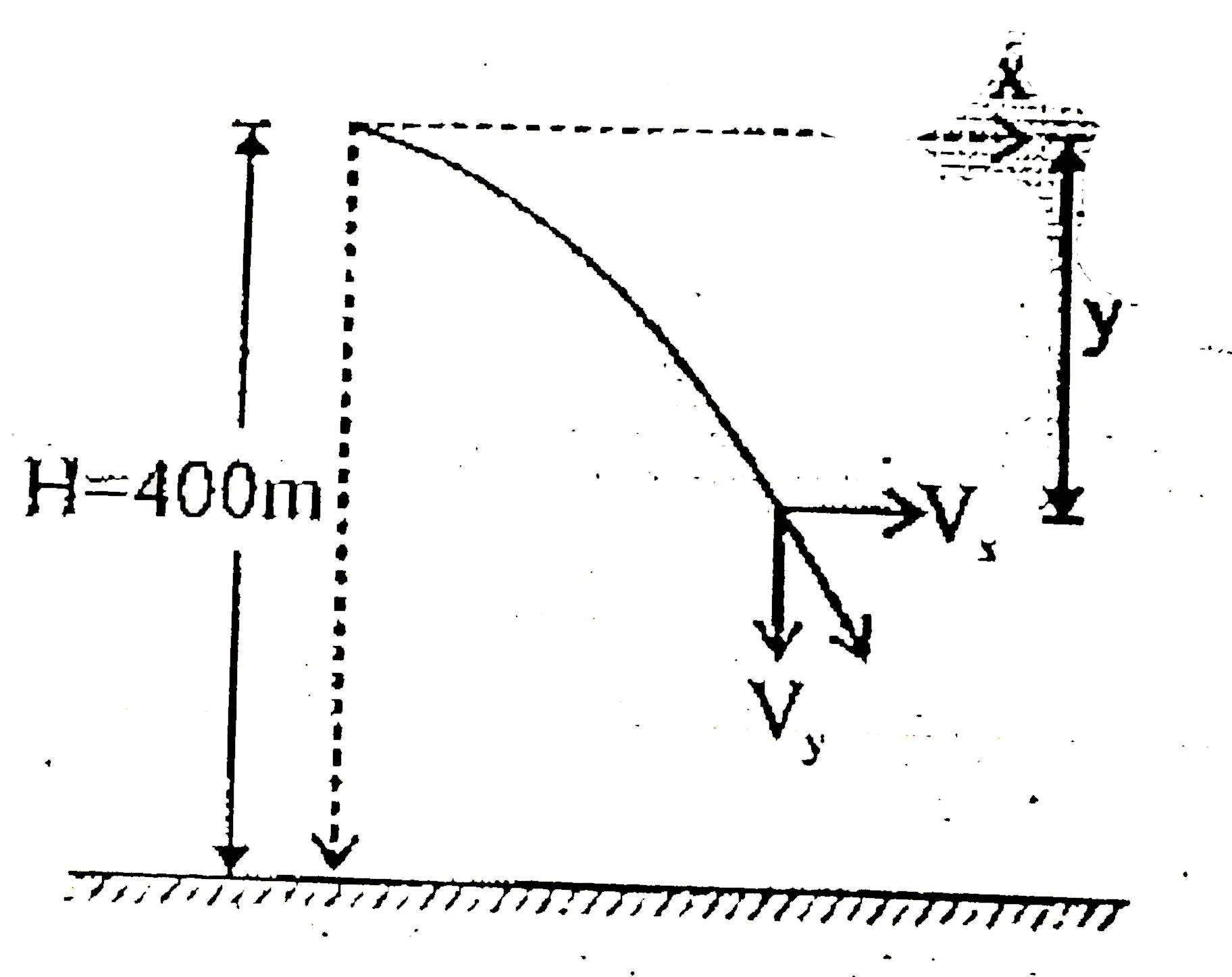
A particle is released from a certain height . Due to the wind, the particle gathers the horizontal velocity component and y is the vertical displacement of the particle from the point of release, then find
(a) the horizontal drift of the particle when it strikes the ground ,
(b) the speed with which particle strikes the ground.
2.67km
8.67km
1.67km
5.1km
. . . (1)
. . . .(2)
Dividing 1 by 2
or
Integrating
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Knowledge Check
In the figure shown, find
(a) the time when the particle strikes the ground at P.
(b) the horizontal distance QP
(c) velocity of the particle at P.
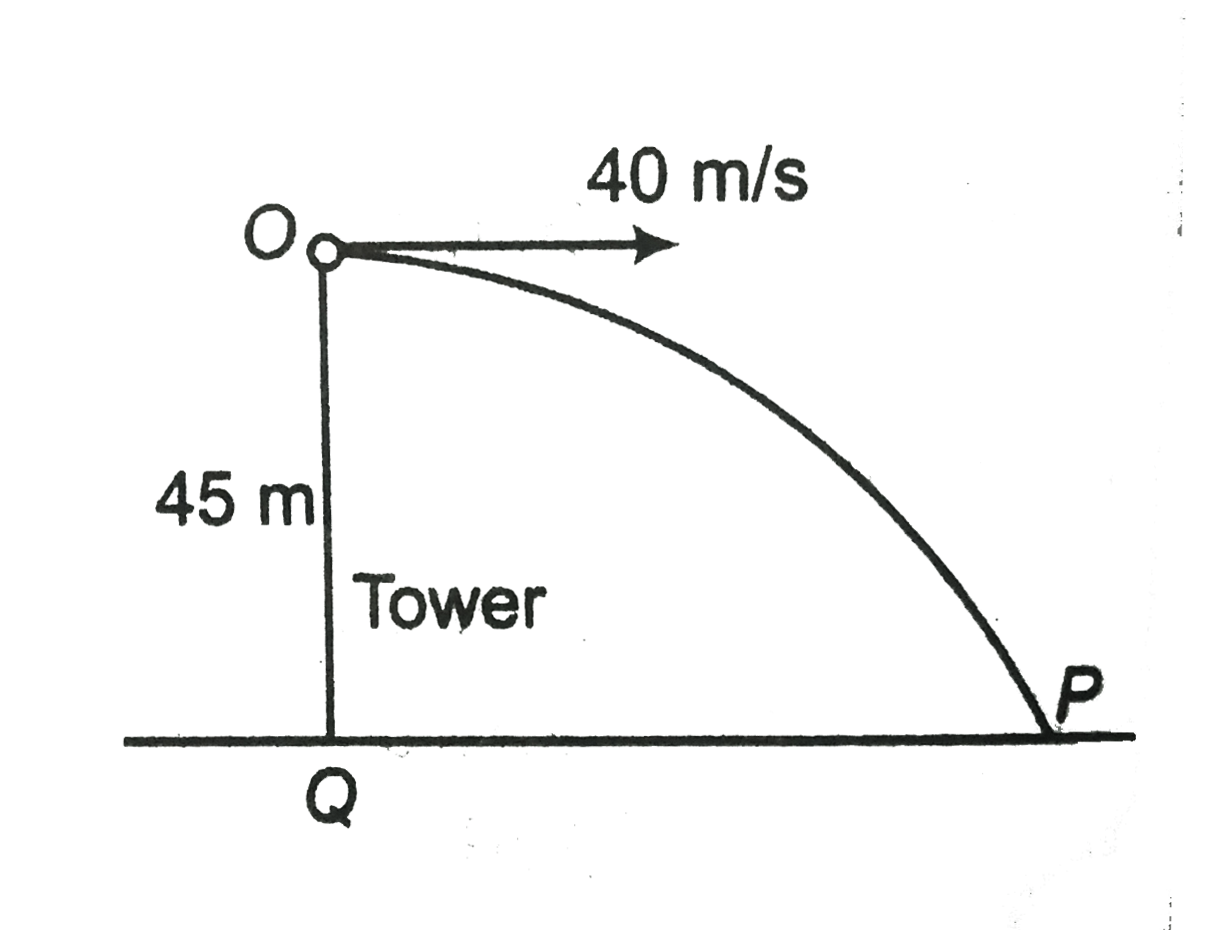 A6s , 120m , 40m/s , 50m/s 37∘B3s , 12m , 40m/s , 50m/s 37∘C3s , 120m , 4m/s , 50m/s 37∘D3s , 120m , 40m/s , 50m/s 37∘
A6s , 120m , 40m/s , 50m/s 37∘B3s , 12m , 40m/s , 50m/s 37∘C3s , 120m , 4m/s , 50m/s 37∘D3s , 120m , 40m/s , 50m/s 37∘in the question number 4, if the horizontal displacement of the particle as seen by an observer on the ground is zero, the speed of the box with respect to the ground at the instant when particle was projected is
AucosαBusinαcosθCucos(α+θ)cosθDusinθsinαcosθParticle is projected from the ground at a certain angle with horizontal . If particle takes time T to hit the ground again , then maximum height of the projectile is
AT2g2BT2g4CT2g8DT2g5
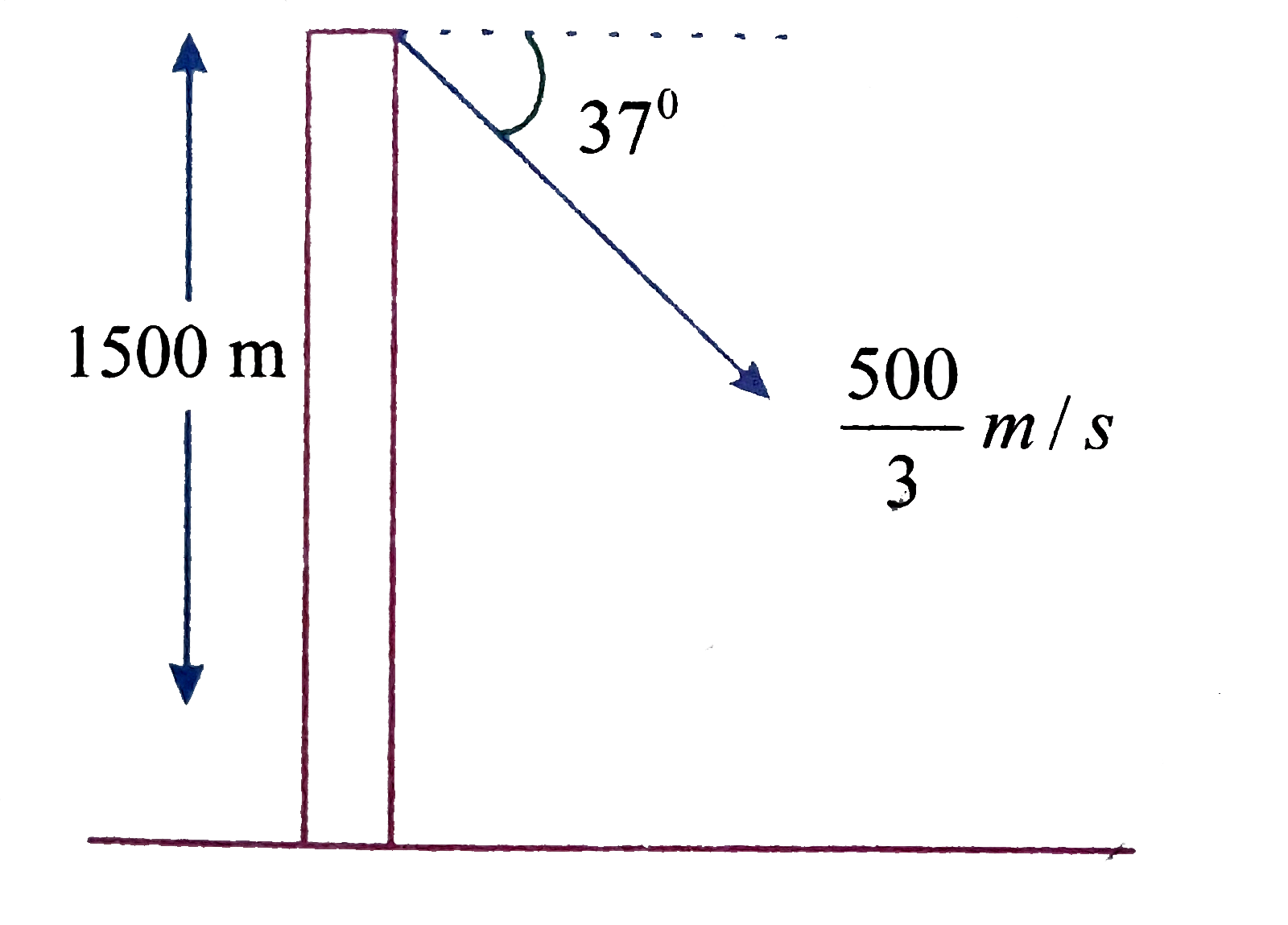
A particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
View SolutionWhat is the average velocity of a particle projected from the ground with speed u at an angle alpha with horizontal over a time interval from beginning till it strikes the ground again?
View SolutionFind the average velocity of a particle released from rest from a height of 125m over a time interval till it strikes the ground. Take g=10m/s2.
View Solution
A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:
View SolutionParticle is dropped form the height of 20m form horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
View Solution
