OX and OY are two coordinate axes. On OY is taken a fixed point P(0,c) and on OX any point Q. On PQ, an equilateral triangle is described, its vertex R being on the side of PQ away from O . Then prove that the locus of R is y=√3x−⋅
This given fixed point is P(0,c).
From given fig.,
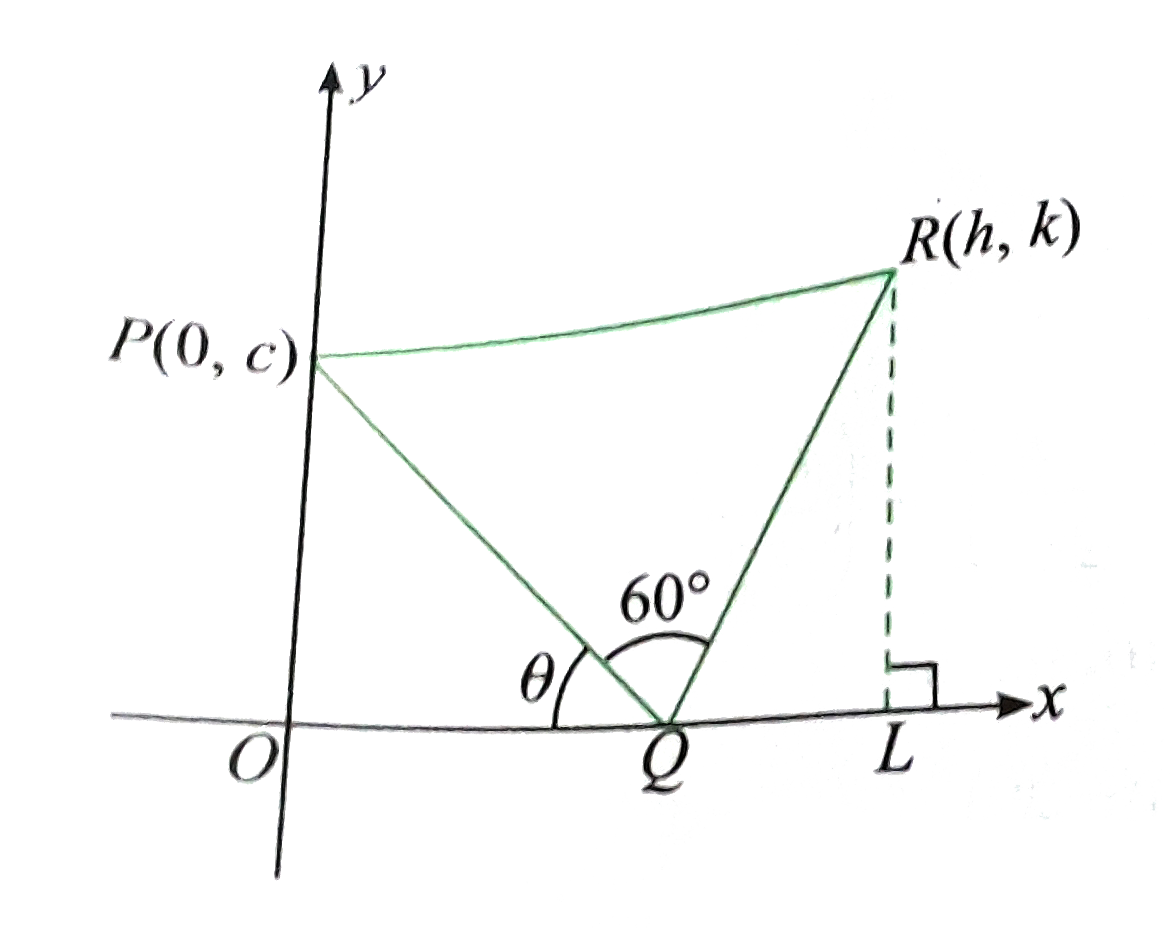
h=OL=OQ+QL
=ccotθ+Qrcos(180∘−60∘−θ)
=ccotθ+PQ{cos120∘.cosθ+sin120∘.sinθ}
=ccotθ−PQ2cosθ+√32PQsinθ
=otθ−12ccosecθcosθ+√32ccosecθsinθ [∵sinθ=cPQ]
∴h=c2(cotθ+√3)(1)
Now, k=RL=RQsin(180∘−60∘−θ)
=PQ{sinθ×12+cosθ×√32}
=ccosecθ{12+sinθ×√32cosθ}
∴ k=c2(1+√3cotθ)
Eliminating cotθ from (1) and (2) , we get k=√3h−c.
Therefore the required locus is y=√3x−c, a straight line.
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.1
6 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.2
8 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
OX and OY are two coordinate axes. On OY is taken a fixed point P(0,c) and on OX any point Q. On PQ, an equilateral triangle is described, its vertex R being on the side of PQ away from O . Then prove that the locus of R is y=√3x−⋅
View SolutionTwo points O(0,0) and A(3,√3) with another point P form an equilateral triangle. Find the coordinates of P.
View Solution
Knowledge Check
The solution of the equation (2+x)dy−ydx=0 represents a curve passing through a fixed point P, then the area of equilateral triangle with P as one vertex and x+y=0 as its one side, is
A2√3B√3C2√3D4√3Let P is a point on the line y+2x=2 and Q and R are two points on the line 3y+6x=3. If the triangle PQR is an equilateral triangle, then its area (in sq. units) is equal to
A115B15√3C13√5D12√15Two point charges +q and −q are held fixed at (−d,o) and (d,0) respectively of a x-y coordinate system. Then
A(a) The electric field E at all points on the x-axis has the same directionB(b) Electric field at all points on y-axis is along x-axisC(c) Work has to be done in bringing a test charge from ∞ to the originD(d) The dipole moment is 2qd along the x-axis
An equilateral triangle PQR is formed where P (1, 3) is fixed point and Q is moving point on line x = 3. Find the locus of R. 9.
View SolutionWrite the coordinate of each of the points P,Q, R,S,T and O from the figure

View SolutionP and Q are two points on the line x−y+1=0. If OP=OQ=6then length of median through O is
View SolutionThe straight line through a fixed point (2,3) intersects the coordinate axes at distinct point P and Q. If O is the origin and the rectangle OPRQ is completed then the locus of R is
View SolutionIf O is the origin and Q is a variable point on y2=x. Find the locus of the mid point of OQ.
View Solution
