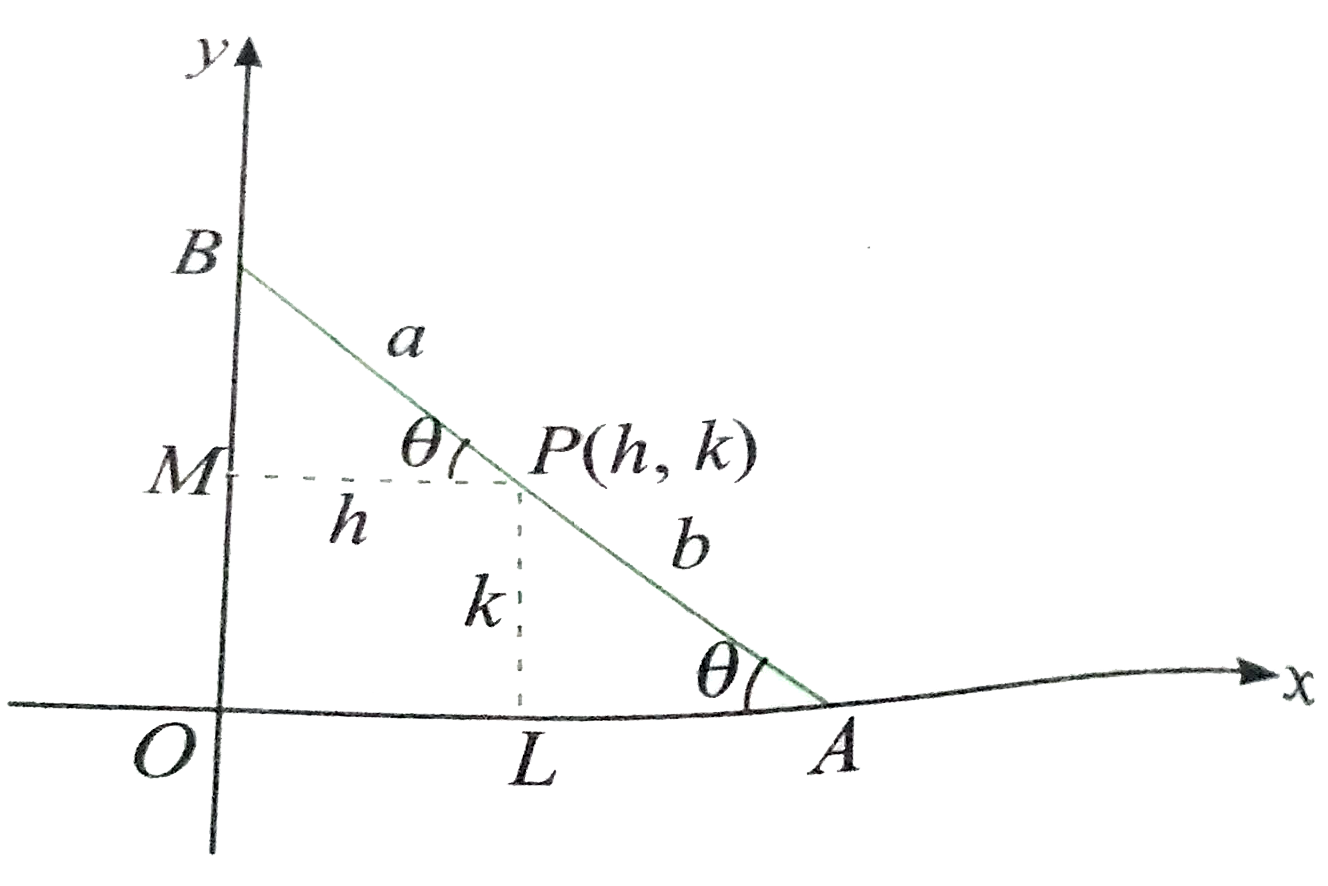
AB is a variable line sliding between the coordinate axes in such a way that A lies on the x-axis and B lies on the y-axis. If P is a variable point on AB such that PA=b,Pb=a , and AB=a+b, find the equation of the locus of P.
Let P(h,k) be a variable point on AB such that ∠OAB=θ.
Here, θ is a variable from triangles ALP and PMB.we have
sinθ=kb (1)
cosθ=ha (2)
Here, θ is a variable so, we have to elimiante θ. Squareing (1) and (2) and adding, we get
k2b2+h2a2=1
x2a2+y2b2=1
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.1
6 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.2
8 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
AB is a variable line sliding between the coordinate axes in such a way that A lies on the x-axis and B lies on the y-axis. If P is a variable point on AB such that PA=b,Pb=a , and AB=a+b, find the equation of the locus of P.
View SolutionA is any point on X-axis and B is any point of Y- axis and P is a variable point on AB with PA=b and PB=aand AB=a+b. Find the equation of locus of P
View Solution
Knowledge Check
In a ΔABC vertex A and B lies on 2 - axis and y -axis respectively, where A(a,0) is a fixed point, B is a variable point such that ∠C=tan−143 and AC = BC, then locus of C
A2x–4y+3a=0B4x+2y–3a=0C2x+4y+7a=0D4x–2y+5a=0If the mid point between the points (a+b,a−b) and (−a,b) lies on the line ax+by=k, what is k equal to ?
Aa/bBa+bCabDa−bA variable line passes through a fixed point (a,b) and meets the coordinate axes in A and B. The locus of the point of intersection of lines through A, B parallel to coordinate axes is
Axa+yb=1Bax+by=1Cxa+yb=2Dxa+yb=3
A rod AB of length 15 cm rests in between two coordinate axes in such a way that the end point A lies on x-axis and end point B lies on y-axis. A point P(x, y) is taken on the rod in such a way that AP = 6 cm. Show that the locus of P is an ellipse.
View SolutionIf A and B are two fixed points and P is a variable point such that PA+PB=4, the locus of P is
View SolutionA rod AB of length 15cm rests in between two coordinate axes in such a way that the end point A lies on x−axis and end point B lies on y-axis . A point is taken on the rod in such a way that AP=6cm . Show that the locus of P is an ellipse.
View SolutionIf the coordinates of a variable point P are (acosθ,bsinθ), where θ is a variable quantity, then find the locus of P.
View SolutionIf the coordinates of a variable point P be (acosθ,bsinθ), where θ is a variable quantity, find the locus of P.
View Solution
