Find the area enclosed by the curves $y = \sqrt{x} and x = - \sqrt{y}$ and the circle $x^{2} + y^{2} = 2$ above the x-axis.

Video Solution

Text Solution

Verified by Experts

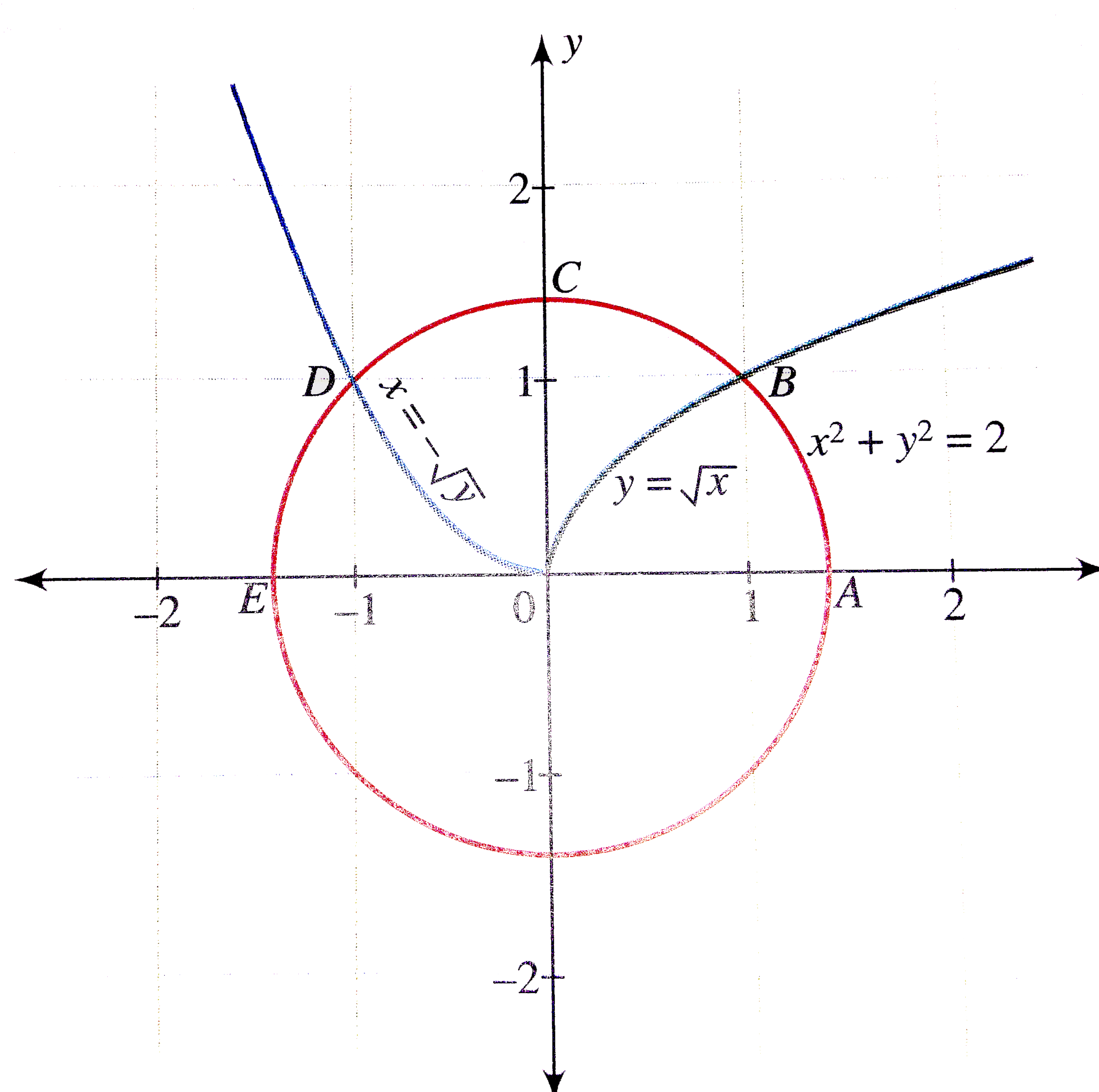
Required area = area of region OBCDO
= area of region OBCD + area of region OCDO
= area of region OBCD + area of region OABO
= area of sector OABCo
$= π / 2$

Updated on:21/07/2023

The area enclosed between the curves $y = x^{3}$ and $y = \sqrt{x}$ is
A $\frac{5}{3}$ sq units
B $\frac{5}{4}$ sq units
C $\frac{5}{12}$ sq units
D $\frac{12}{5}$ sq units
Find the area enclosed by the curves $y = 2 - | 2 - x | and y = \frac{3}{| x |}$
A $3 - 4 log 3$
B $\frac{4 - 3 log 3}{2}$
C $2 {log 3}^{3 / 2}$
D $2 - log 3$