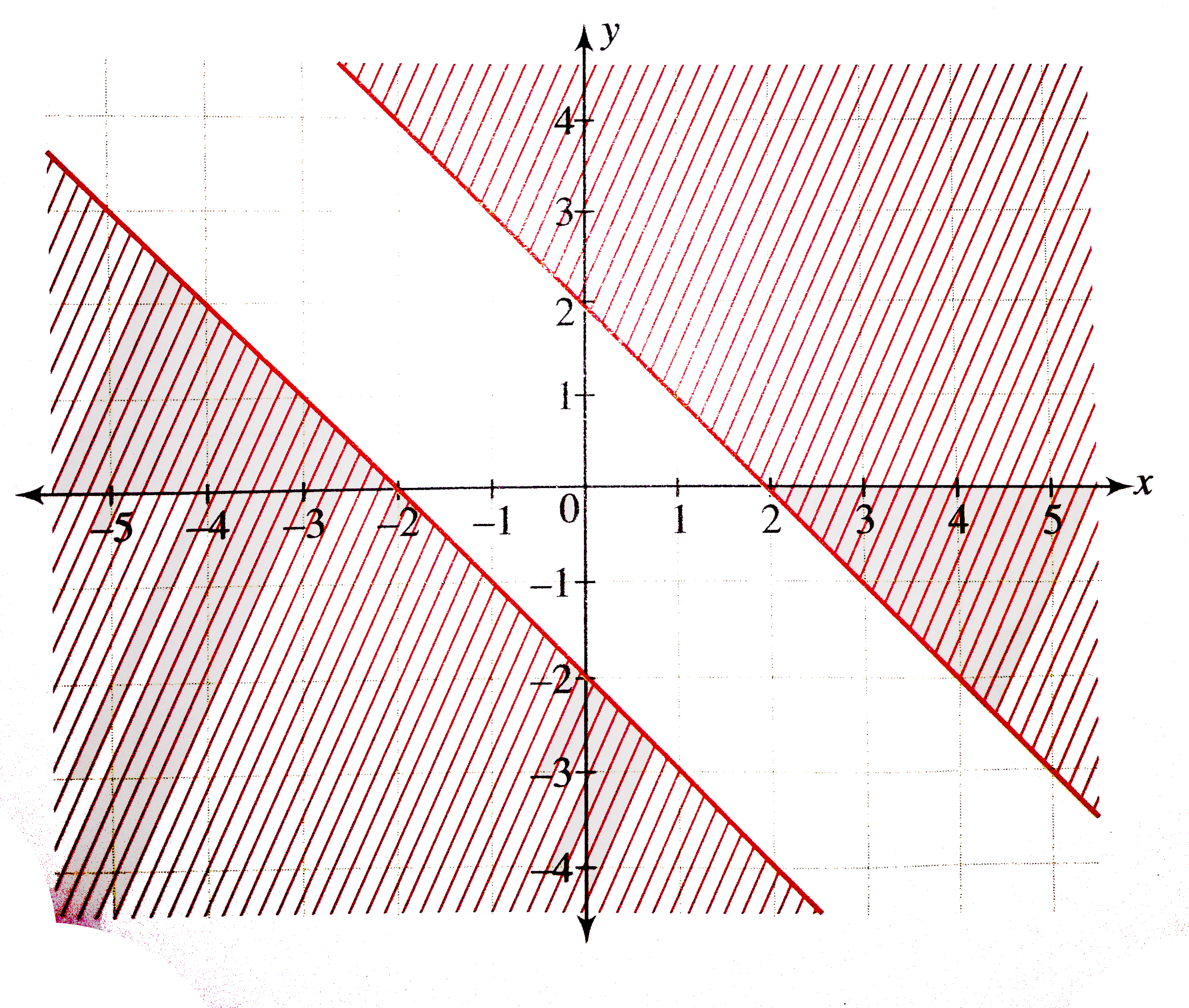
Sketch the regions which points satisfy |x+y|≥2.
Video Solution
Text Solution
|x+y|≥2
x+y≤−2oex+y≥2
That is,the points lie in the region satisfying the inequality x+y+2≥0orx+y−2≤0.
For x+y+2≤0, the points lie on the line x+y+2=0 or in the half region in which (0,0) does not lie as (0, 0) does not satisfy x+y+2≤0.
For x+y−2≥0, the points lie on the (0, 0) does not satisfy x+y−2≥0.
Hence the required region is as shown in the following figure.
|
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Shade the regions where points satisfy |x−y|<1.
View SolutionFind the area of the region in which points satisfy
3≤|x|+|y|≤5.
View SolutionSketch the region of the points satisfying max.{|x|,|y|}≤4.
View SolutionSketch the origin in which the points satisfying the following inequalities lie.
(i)|x+y|<2 (ii) |2x−y|>3 (iii) |x|>|y|
View SolutionSketch the region for ∣y⊨sinx.
View SolutionFind the area of the region formed by the points satisfying
|x|+|y|+|x+y|≤2.
View SolutionSketch the region satisfying |x|<|y|.
View SolutionSketch the region for y=sin(x−[x]).
View SolutionPlot the region of the points P(x,y) satisfying 2> max.
{|x|,|y|}.
View SolutionPlot the region of the points P (x,y) satisfying |x|+|y|<1.
View Solution
