Supose a planet is revolving around the sun in an elliptical path given by x2a2+y2b2=1
Find time period of revolution Angular momentum of the planet about the sun is L
 .
.
Video Solution
Text Solution
Rate of area swept dAdt=L2m =constant
⇒dA=L2mdt
A=πab∫A=0dA=t=T∫t=0L2mdt
⇒πab=L2mT⇒T=2πmabL .
|
Topper's Solved these Questions
- GRAVITATIONBOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - SOLVED MISCELLANEOUS PROBLEMS
5 Videos
- GRAVITATIONBOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXAMPLE
2 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems
35 Videos
- NUCLEAR PHYSICSBOOK - RESONANCECHAPTER - NUCLEAR PHYSICSEXERCISE - Advanced level solutions
16 Videos
Similar Questions
Knowledge Check
A planet is revolving around the sun as shown in elliptical path. The correct option is
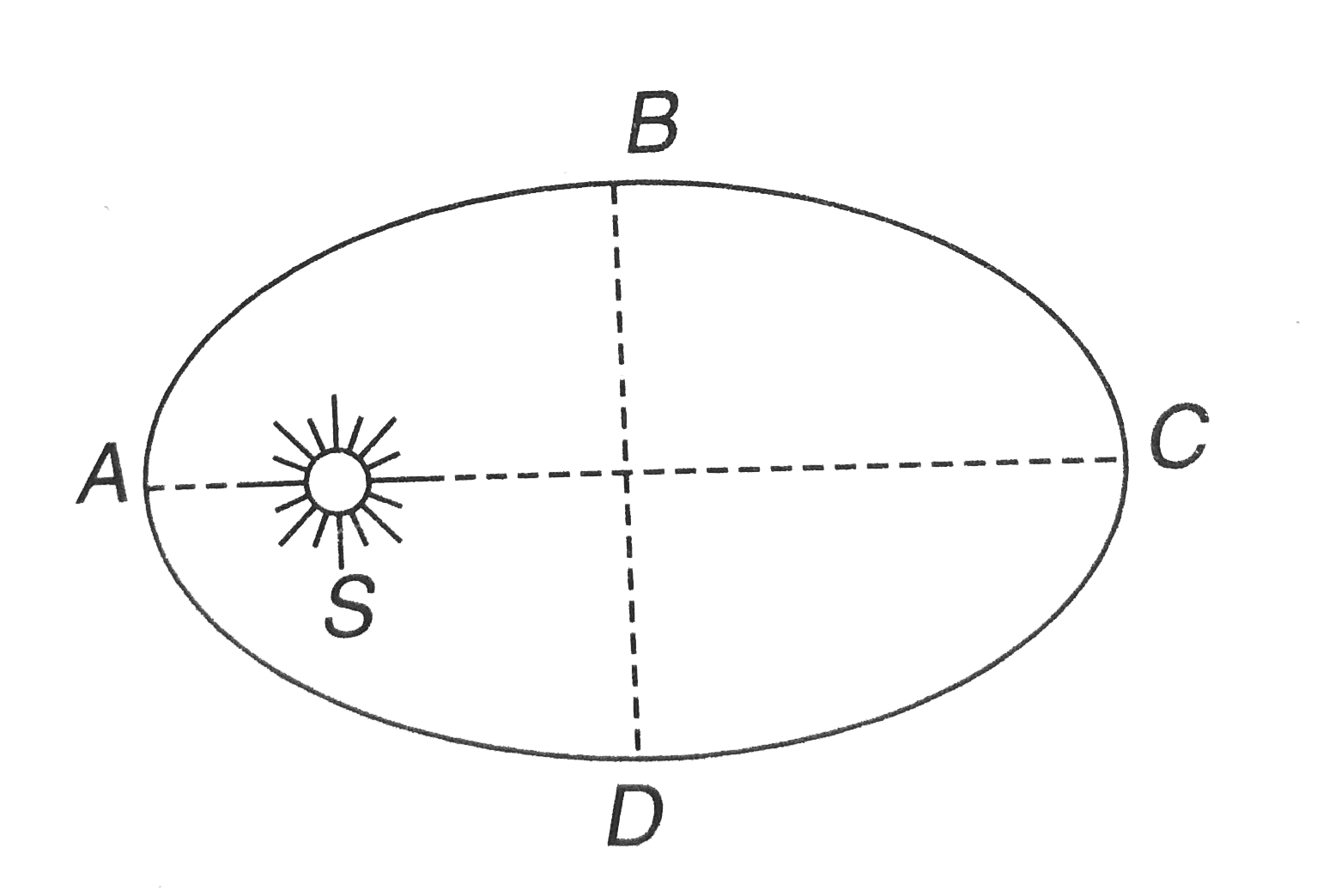 AThe time taken in travelling DAB is less than that for BCDBThe time taken in travelling DAB is greater than that for BCDCThe time taken in travelling CDA is less than that for ABCDThe time taken in travelling CDA is greater than that for ABC
AThe time taken in travelling DAB is less than that for BCDBThe time taken in travelling DAB is greater than that for BCDCThe time taken in travelling CDA is less than that for ABCDThe time taken in travelling CDA is greater than that for ABCA planet revolves round the sun in an elliptical orbit of semi minor and semi major axes x and y respectively. Then the time period of revolution is proportional to
A(x+y)32B(y−x)32Cx32Dy32A planet is revolving around the Sun in an elliptical orbit. Its closest distance from the Sun is r and farthest distance is R. If the orbital velocity of the planet closest to the Sun is v, then what is the velocity at the farthest point?
AvrRBvRrCv√rRDv√Rr
For planets revolving round the sun, show that T2∝r3, where T is the time period of revolution of the planet and r is its distance from the sun.
View SolutionFind the period of revoluition of planet Mars about the sun if mean distance of the Mars from the sun is 1.52AU.
View SolutionFor a planet revolving around Sun in an elliptical orbit, to obtain its velocity at any point we have to apply
View SolutionA planet revolves around the sun in elliptical orbit of eccentricity 'e'. If 'T' is the time period of the planet then the time spent by the planet between the end of the minor axis and close to the sun is
View SolutionAll planets revolve around the Sun in fixed elliptical paths called orbits. This motion is called ______ .
View Solution
