Derive the expression of electric field intensity at a point 'P' which is situated at a distance x on the axis of uniformly charges disc of radius R and surface charge density σ. Also, derive results for
(i) x>>R (ii) x<<R
The disc can be considered to be a collection of large number of concentric rings. Consider an element of the shape of rings of radius r and of width dr. electric field due to this ring at P is
dE=K.σ2πr.dr.x(r2+x2)3/2
Put, r2+x2=y2
2rdr=2ydy
∴dE=K.σ2πy.dy.xy3=2Kσπ.xydyy3
Electric field at P due to all tings is along the axis :
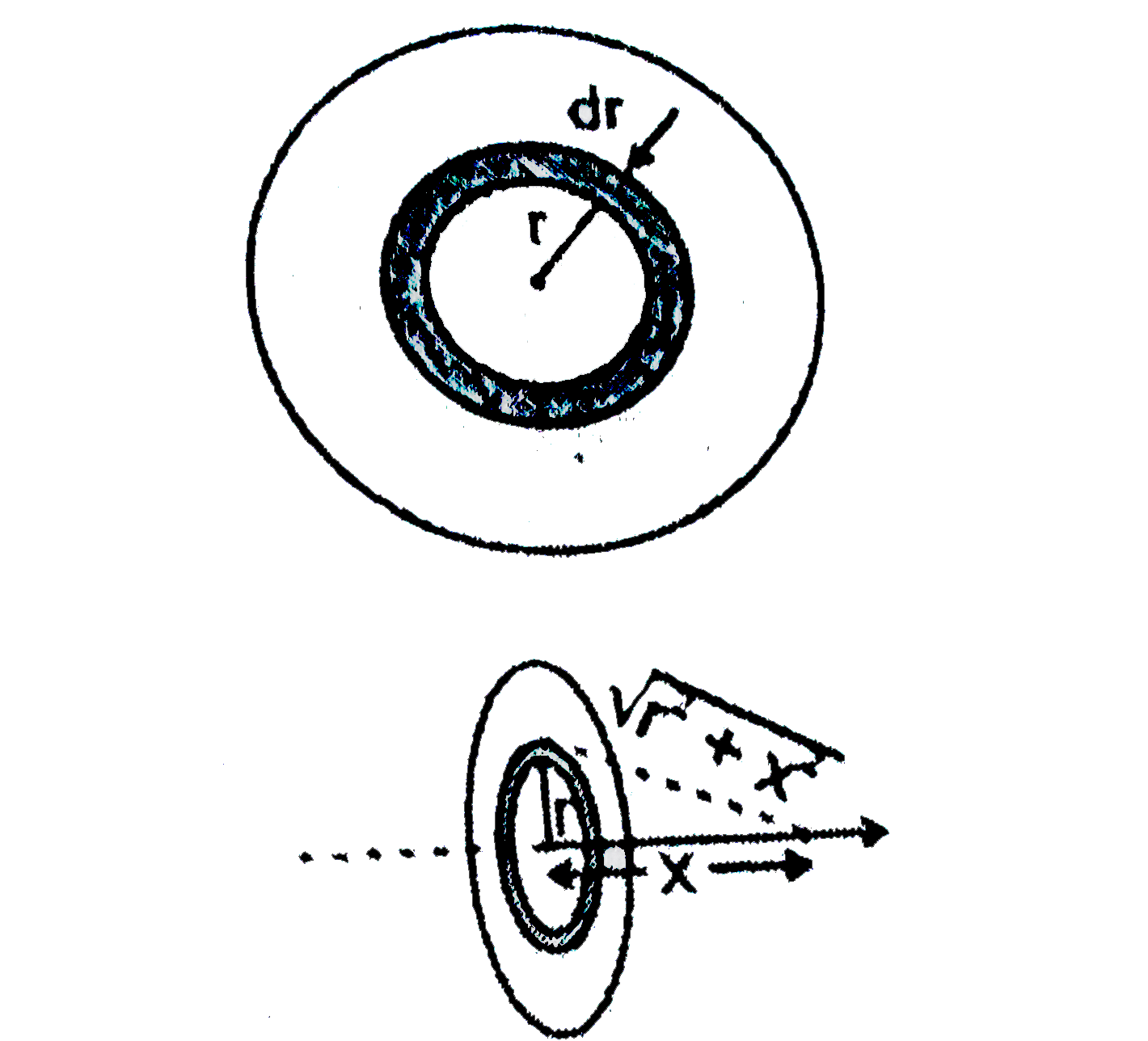
∴E=∫dE⇒E=2Kσπx√R2+x2∫x1y2dy=2Kρπx.[−1y]√R2+x2x
=2Kσπx[+1x−1√R2+x2]=2Kσπ[1−x√R2+x2]
=σ2ε0[1−x√R2+x2] along the axis
Cases : (i) If x>>R
E=σ2ε0⎡⎢
⎢⎣1−xx√R2x2+1⎤⎥
⎥⎦=σ2ε0[1−(1+R2x2)−1/2]
σ2ε0=[1−1+12R2x2+ higher order terms]=σ4ε0R2x2=σπR24πε0x2=Q4πε0x2
i.e., behaviour of the disc is like a point charge.
(ii) If x<<R
E=σ2ε0[1−0]=σ2ε0
i.e., behaviour of the disc is like infinite sheet.
Topper's Solved these Questions
- ELECTROSTATICSBook:RESONANCEChapter:ELECTROSTATICSExercise:Problems
Explore 22 Videos
- ELECTROSTATICSBook:RESONANCEChapter:ELECTROSTATICSExercise:Exercise-1 Section (A)
Explore 9 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCEChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCEChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
Similar Questions
Knowledge Check
The electric field intensity in free space at a distance 'r' outside a charged conducting sphere of radius 'R' in terms of surface charge density σ is
Aσε0[Rr]2Bε0σ[Rr]2CRr[σε0]2DRσ[rε0]2The electric field intensity in free space at a distance 'r' outside a charged conducting sphere of radius 'R' in terms of surface charge density σ is
Aσε0[Rr]2Bε0σ[Rr]2CRr[σε0]2DRσ[rε0]2The magnitude of an electric intensity at a point which is at a distance 'r' from the centre of a charged spherical conductor of radius 'R' in terms of the surface charge density 'σ' is given by 'E' where
AE=σKε0r2BE=σRKε0r2CE=σR2Kε0r2DE=σ2RKε0r2
Calculate electric field at a point on axis, which at a distance x from centre of uniformly charged disc having surface charge density σ and R which also contains a concentric hole of radius r.
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View Solution8.The diagram shows a part of uniformly charged disc of radius R having surface charge density sigma .If electric potential at the center 0 is (1)/(N)times(sigma R)/(2 varepsilon_(0)) Then find N .
View SolutionThe electric field intensity at a point located at distance r(r<R) from the center of a spherical conductor (radius R) charged Q will be-
View SolutionThe electric intensity of outside a charged sphere of radius R at a distance r(r>R) is
View Solution