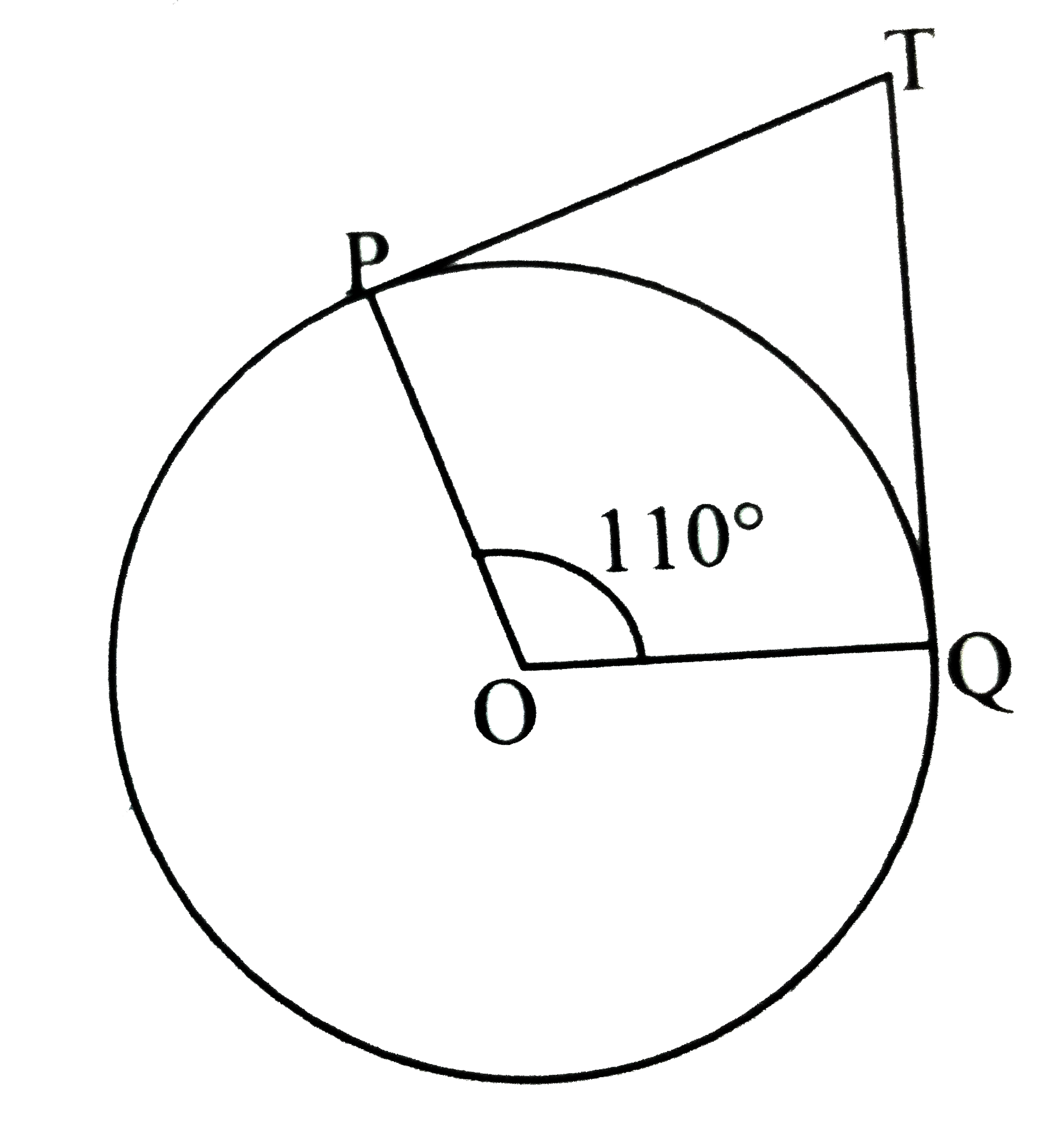
If TP and TQ are the two tangents to a circle with centre O so that ∠POQ=110∘, then ∠PTQ is equal to
To solve the problem, we need to find the angle ∠PTQ given that ∠POQ=110∘ and that TP and TQ are tangents to the circle at points P and Q respectively.
1. Identify the Given Information:
- We have a circle with center O.
- TP and TQ are tangents to the circle at points P and Q.
- The angle ∠POQ=110∘.
2. Use the Property of Tangents:
- The radius at the point of contact is perpendicular to the tangent. Therefore:
- OP⊥TP which means ∠OPT=90∘.
- OQ⊥TQ which means ∠OQT=90∘.
3. Analyze Quadrilateral OPTQ:
- The angles in a quadrilateral sum up to 360∘.
- Therefore, we can write the equation:
∠O+∠P+∠T+∠Q=360∘
4. Substitute the Known Angles:
- We know:
- ∠O=110∘
- ∠P=90∘
- ∠Q=90∘
- Let ∠T=∠PTQ (the angle we need to find).
- Substitute these values into the equation:
110∘+90∘+∠PTQ+90∘=360∘
5. Simplify the Equation:
- Combine the known angles:
290∘+∠PTQ=360∘
6. Solve for ∠PTQ:
- Rearranging gives:
∠PTQ=360∘−290∘=70∘
Final Answer:
Thus, ∠PTQ=70∘.
---
- We have a circle with center O.
- TP and TQ are tangents to the circle at points P and Q.
- The angle ∠POQ=110∘.
- The radius at the point of contact is perpendicular to the tangent. Therefore:
- OP⊥TP which means ∠OPT=90∘.
- OQ⊥TQ which means ∠OQT=90∘.
- The angles in a quadrilateral sum up to 360∘.
- Therefore, we can write the equation:
∠O+∠P+∠T+∠Q=360∘
- We know:
- ∠O=110∘
- ∠P=90∘
- ∠Q=90∘
- Let ∠T=∠PTQ (the angle we need to find).
- Substitute these values into the equation:
110∘+90∘+∠PTQ+90∘=360∘
- Combine the known angles:
290∘+∠PTQ=360∘
- Rearranging gives:
∠PTQ=360∘−290∘=70∘
Thus, ∠PTQ=70∘.
Topper's Solved these Questions
- CIRCLESBOOK - NCERTCHAPTER - CIRCLESEXERCISE - Exercise 10.1
2 Videos
- CIRCLESBOOK - NCERTCHAPTER - CIRCLESEXERCISE - SOLVED EXAMPLES
3 Videos
- ARITHMETIC PROGRESSIONSBOOK - NCERTCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Exercise 5.3
20 Videos
- CONSTRUCTIONSBOOK - NCERTCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE 11.2
7 Videos
Similar Questions
Knowledge Check
If TP and TQ are two tangents to a circle with centre O, so that ∠POQ=120∘, then ∠PTQ is equal to
A120∘B30∘C60∘D90∘TP and TQ are the tangents to a circle, with centre O. Find x.
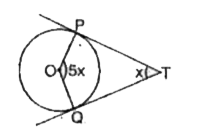 A15∘B60∘C30∘D45∘
A15∘B60∘C30∘D45∘In the figure, TP and TQ are tangents drawn to the circle with centre at O. If ∠POQ=115∘ then ∠PTQ is:
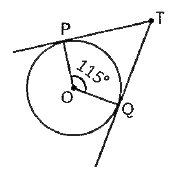 A115∘B57.5∘C55∘D65∘
A115∘B57.5∘C55∘D65∘
If TP and TQ are the two tangents to a circle with centre O. So that ∠PQR=105∘, then ∠PTQ is ………….. .
View SolutionIf TPandTQ are the two tangents to a circle with centre O. so that ∠POQ=115∘, then ∠PTQ=……….(65∘).
View SolutionIn the given figure ,if TP and TQ are tangents to a circle with centre O, so that ∠POQ=110∘ ,then ∠PTQ is
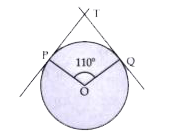
View SolutionIn Fig. 10.96, if TP and TQ are tangents drawn from an external points T to a circle with centre O such that ∠TQP=60o , then ∠OPQ= (FIGURE) 25o (b) 30o (c) 40o (d) 60o
View SolutionTP and TQ are the tangents from the external point T of a circle with centre O. If ∠OPQ=30∘ then find the measure of ∠TQP.
View Solution