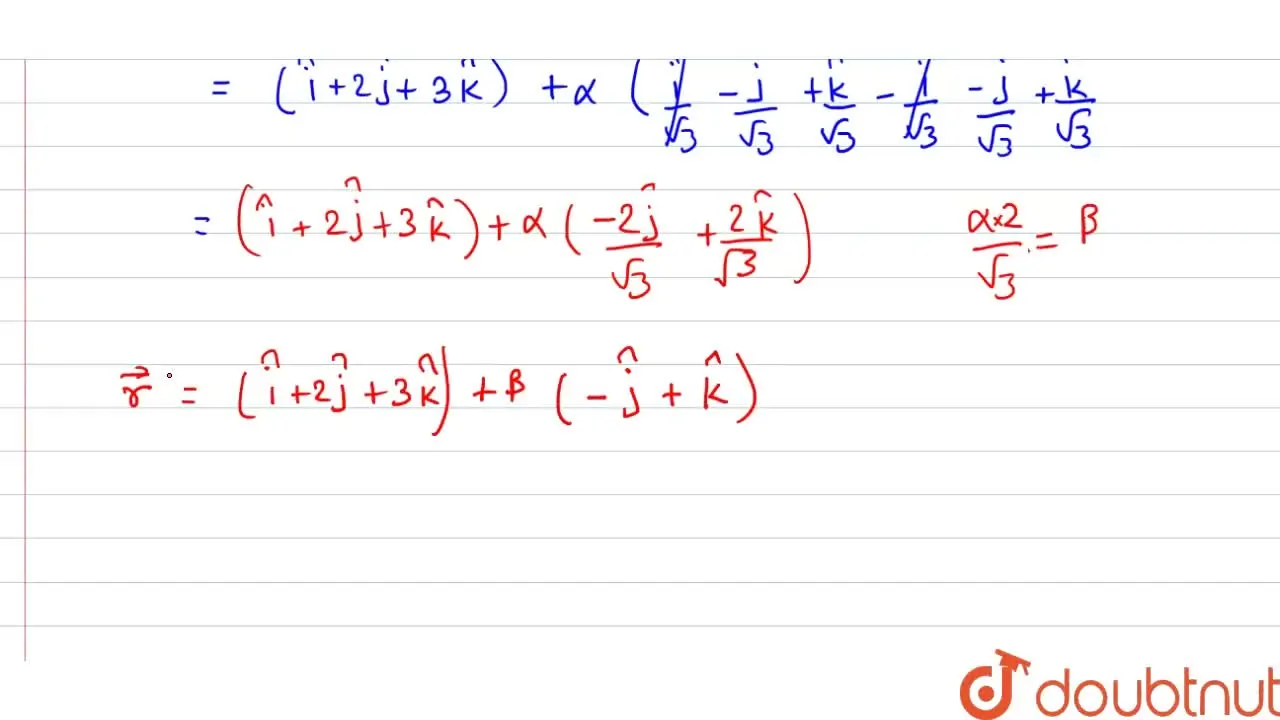
If $\to r = (ˆ i + 2 ˆ j + 3 ˆ k) + λ (ˆ i - ˆ j + ˆ k) and \to r = (ˆ i + 2 ˆ j + 3 ˆ k) + μ (ˆ i + ˆ j - ˆ k)$ are two lines, then find the equation of acute angle bisector of two lines.

Video Solution

Text Solution

Verified by Experts

Lines are $\to r = (ˆ i + 2 ˆ j + 3 ˆ k) + λ (ˆ i - ˆ j + ˆ k) and \to r = (ˆ i + 2 ˆ j + 3 ˆ k) + μ (ˆ i + ˆ j - ˆ k)$
along vectors $(ˆ i - ˆ j + ˆ k) and (ˆ i + ˆ j - ˆ k)$ , respectively.
Angle between two lines = ${cos}^{- 1} (\frac{(1) \times (1) + (- 1) (1) + (1) (- 1)}{\sqrt{3} \sqrt{3}}) = {cos}^{- 1} (- \frac{1}{3})$
which is an obtuse angle.
$∴$ Vector along acute angle bisector = $λ [\frac{ˆ i - ˆ j + ˆ k}{\sqrt{3}} - \frac{ˆ i + ˆ j - ˆ k}{\sqrt{3}}] = \frac{2 λ}{\sqrt{3}} (- ˆ j + ˆ k)$
$∴$ Equation of acute angle bisector = $(ˆ i + 2 ˆ j + 3 ˆ k) + t (ˆ j - ˆ k)$

Updated on:21/07/2023

The lines $\to r = (ˆ i + ˆ j) + λ (ˆ i + ˆ k) and \to r = (ˆ i + ˆ j) + μ (- ˆ i + ˆ j - ˆ k)$ are
Aparallel
Bnon-intersecting
Cintersecting
DNone of these
The lines $\to r = (2 ˆ i - 3 ˆ j + 7 ˆ k) + λ (2 ˆ i + p ˆ j + 5 ˆ k)$ and $\to r = (ˆ i + 2 ˆ j + 3 ˆ k) + μ (3 ˆ i - p ˆ j + p ˆ k)$ are perpendicular it $p =$
A $- 6, - 1$
B $- 1, 6$
C $2, 3$
Dnone of these
The lines given by $\to r = (ˆ i - ˆ j) + λ (2 ˆ i + ˆ k)$ and $\to r = (2 ˆ i - ˆ j) + μ (ˆ i + ˆ j - ˆ k)$ are
Aparallel
Bperpendicular
Cskew
Dintersecting