Draw the graph of y=|sinx| and hence the graph of y=sin−1|sinx|.
Video Solution
Text Solution
The graph of y=sinx is as follows.
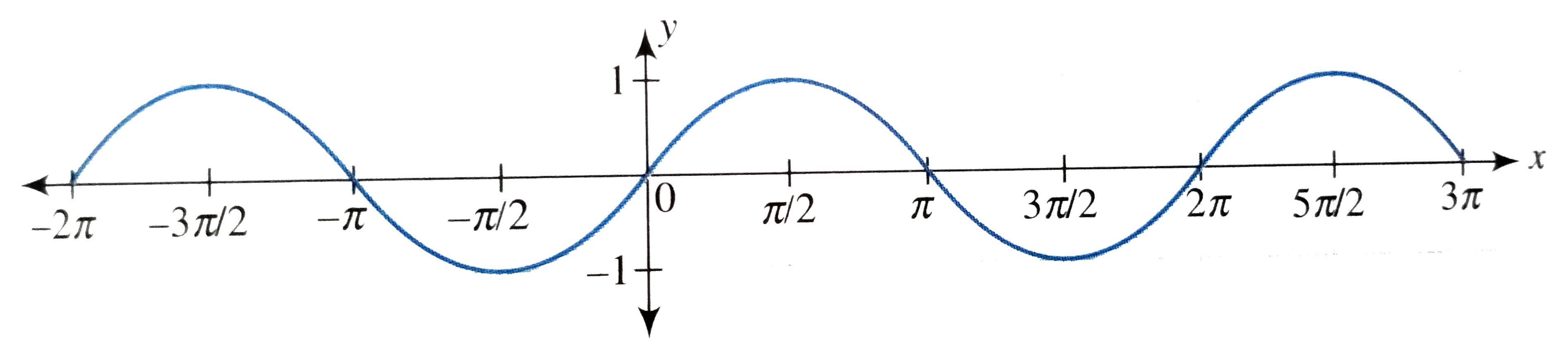 Therefore, the graph of y=|sinx| can be drawn as shown in the following figure.
Therefore, the graph of y=|sinx| can be drawn as shown in the following figure.
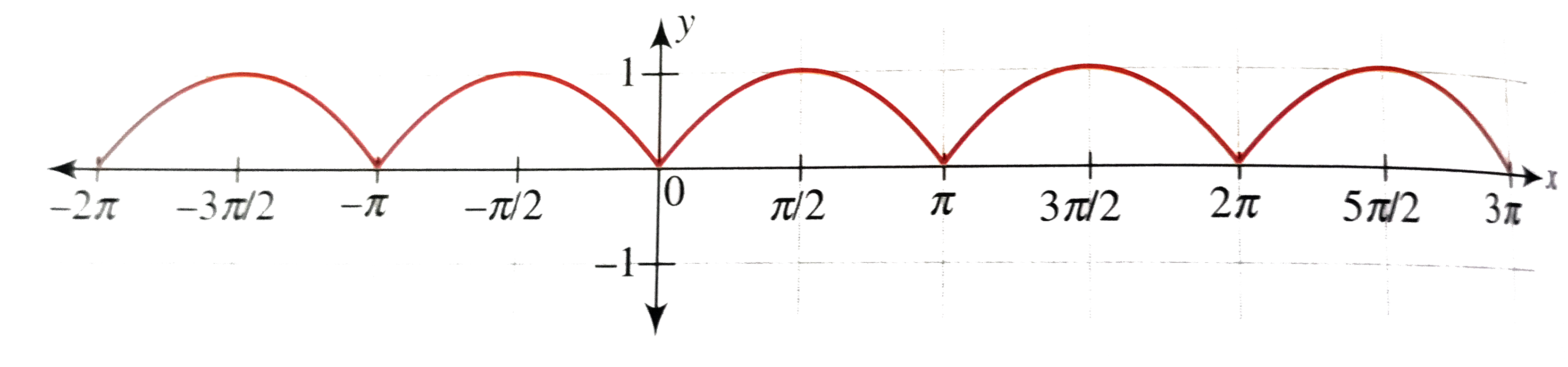
From the above graph, it is clear that y=|sinx| is non-differentiable for x=nπ,n∈Z Now y=f(x)=sin−1|sinx|.
Now it is easy to draw the graph of y=f(x)=sin−1|sinx| from the above graph.
f(0)=0,f(π/2)=π/2,f(π)=0. Also the period of y=f(x) is π.
Hence the graph of y=f(x) is as shown in the following figure.
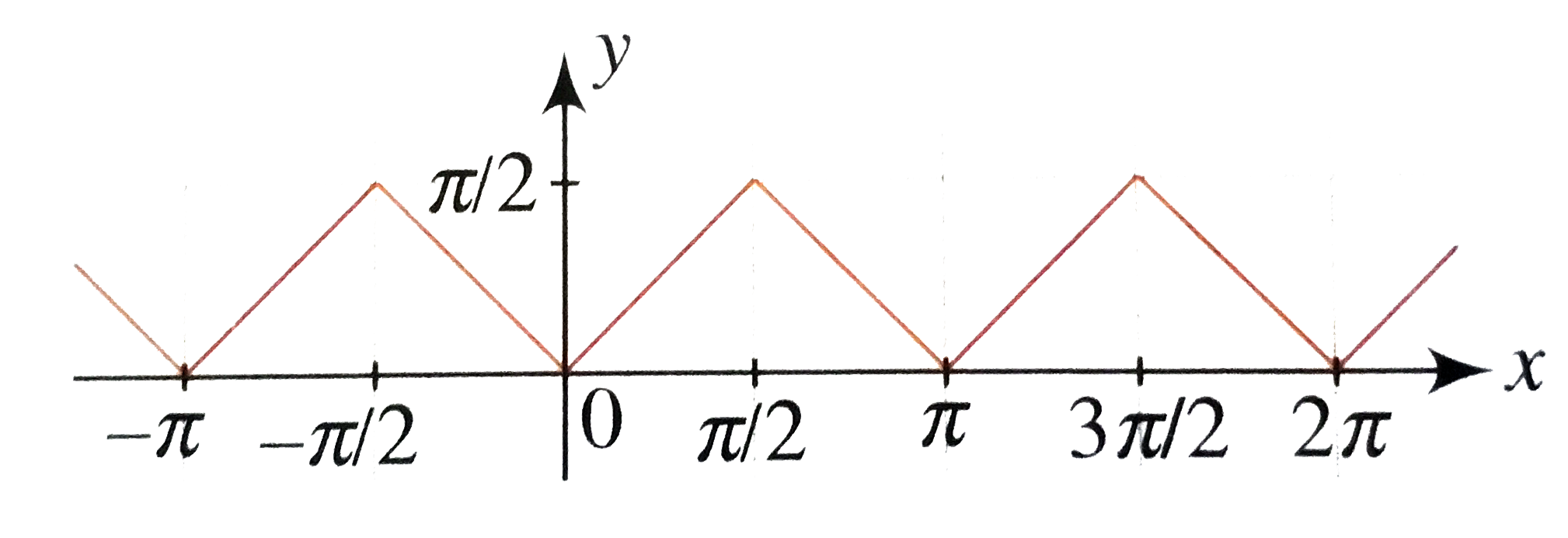
|
Topper's Solved these Questions
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise
22 Videos
- GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
18 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
Similar Questions
Draw the graph of y=x−sinx
View SolutionDraw the graph of y=|x|
View SolutionDraw the graph of y=[sinx].
View SolutionDraw the graph of y=sin|x|.
View SolutionDraw the graph of |y|=sinx.
View SolutionDraw the graph of y=sin−1|x|.
View SolutionDraw the graph of y=3sin2x.
View SolutionDraw the graph of y=−x
View SolutionDraw the graph of y=|x−3|+1.
View SolutionDraw the graph of y=2−|x−1|.
View Solution
