A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. The ratio AXBX is
√2√2+1
√2−1√2+1
√3−√2√3+√2
√2+1√2−1
The correct Answer is:B
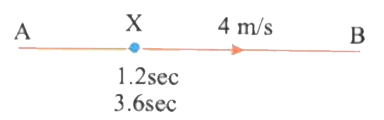
T=4.8sec,t=1.22=0.6sec=T8
x=−A√2,AXXB=A−|x|A+|x|
Topper's Solved these Questions
Similar Questions
A particle is executing SHM in straight line starting its motion at t1=0 with zero initial phase.It crosses a point during the motion at successive intervals at t2=t and t3=2t with a speed v. If its acceleration amplitude is 2πVNt then N is equal to
View Solution(i) The acceleration (a) of a particle moving along a straight line is related to time (t) as per the differential equation d2adt2=−ba. b is a positive constant. Is the particle performing SHM? If yes, what is the time period?
(ii) A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P (AP>PB) with a speed of 3m/s at times recorded as t = 0, 0.5 s, 2.0 s, 2.5 s, 4.0 s, 4.5 s......Determine the maximum speed of the particle and also the ratio AP/PB.
View Solution
Knowledge Check
A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. Amplitude of oscillation isA10√3πmB4√2πmC9√2πmD17√2πmX1 and X2 are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X(X1X<X2X) then particle crosses this point again at successive intervals of 2s and 4s with a speed of 5m/s.
The time period of SHM isA6sB3sC4sD2sA particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(AP<BP) at successive intervals of 0.5 sec and 1.5 sec with a speed of 3 m/s. Then
AThe maximum speed of particle is 3√2 m/sBthe maximum speed of particle is √2 m/sCthe ratio APBP is sqr2−1√2+1Dthe ratio APBP is 1√2
A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.
View SolutionA particle moves in a straight line so that its velocity at any point is given by v2=a+bx, where a,b≠0 are constants. The acceleration is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionThe time taken by a particle performing SHM on a straight line to pass from point A to B where its velocities are same is 2 seconds .After another 2 seconds it return to B The time period of oscillation is (in seconds)
View SolutionThe periodic time of a particle executing a linear S.H.M. is 2πω. If its velocity at a dis"tan"ce b from the mean position is √3bω, then the path length of the particle is
View Solution
