Light ray is travelling from a denser medium into a rarer medium. The velocity of light in the denser and rarer medium is 2×108 m/sec and 2.5×108 m / sec. The critical angle of the two media is
sin−1(54)
sin−1(45)
sin−1(12)
sin−1(35)
The correct Answer is:B
To find the critical angle for light traveling from a denser medium to a rarer medium, we can follow these steps:
Step 1: Identify the velocities of light in both media
- Given:
- Velocity of light in the denser medium, vd=2×108 m/s
- Velocity of light in the rarer medium, vr=2.5×108 m/s
Step 2: Calculate the refractive indices of both media
- The refractive index μ is given by the formula:
μ=cv
where c is the speed of light in vacuum (approximately 3×108 m/s).
- For the denser medium:
μd=cvd=3×1082×108=32
- For the rarer medium:
μr=cvr=3×1082.5×108=32.5=65
Step 3: Apply Snell's Law to find the critical angle
- Snell's Law states:
μdsinC=μrsin90∘
Since sin90∘=1, we can simplify this to:
μdsinC=μr
- Rearranging gives:
sinC=μrμd
Step 4: Substitute the values of the refractive indices
- Substituting the values we calculated:
sinC=6532=65×23=1215=45
Step 5: Calculate the critical angle
- To find the critical angle C, we take the inverse sine:
C=sin−1(45)
Final Result
- The critical angle C is:
C≈53.13∘
- Given:
- Velocity of light in the denser medium, vd=2×108 m/s
- Velocity of light in the rarer medium, vr=2.5×108 m/s
- The refractive index μ is given by the formula:
μ=cv
where c is the speed of light in vacuum (approximately 3×108 m/s).
μd=cvd=3×1082×108=32
μr=cvr=3×1082.5×108=32.5=65
- Snell's Law states:
μdsinC=μrsin90∘
Since sin90∘=1, we can simplify this to:
μdsinC=μr
sinC=μrμd
- Substituting the values we calculated:
sinC=6532=65×23=1215=45
- To find the critical angle C, we take the inverse sine:
C=sin−1(45)
- The critical angle C is:
C≈53.13∘
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (C.W)(REFRACTION THROUGH SPHERICAL SURFACES )
Explore 29 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (C.W)(REFRACTION THROUGH A PRISM )
Explore 8 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (C.W)(REFLECTION)
Explore 18 Videos
- NUCLEI Book:NARAYNAChapter:NUCLEI Exercise:ASSERTION & REASON
Explore 5 Videos
- SEMI CONDUCTOR DEVICESBook:NARAYNAChapter:SEMI CONDUCTOR DEVICESExercise:Level-II (H.W)
Explore 36 Videos
Similar Questions
Knowledge Check
When light passes from a denser medium to a rarer medium
AVelocity of light increasesBVelocity of light decreasesCVelocity of light remains unchangedDVelocity of light becomes infinityWhen a ray of light travels from a denser medium to a rarer medium, the ray will :
Aslow down and bend away from the normalBslow down and bend towards the normalCspeed up and bend away from the normalDspeed up and bend towards the normalThe velocities of light in two different mediums are 2×108ms−1 and 2.5×108ms−1 respectively. The critical angle for there mediums is
Asin−1(15)Bsin−1(45)Csin−1(12)Dsin−1(14)
A ray of light travelling obliquely from denser to rarer medium
View SolutionWhen a wave travels from a denser to rarer medium, then
View SolutionWhen a ray of light passes from a denser medium to a rarer medium, the ray …………. .
View SolutionA wave travels from a denser medium to rarer medium, then match the following two columns
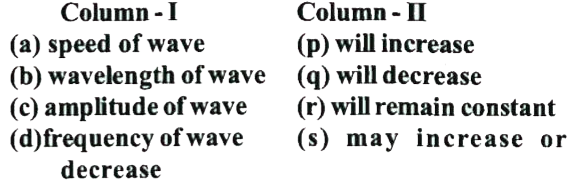
View SolutionThe ray of light travelling from an optically denser medium to optically rarer medium bends
View Solution