In a regular hexagon two −−→PQ=→A,−−→RP=→B. Express other vectors's in term of them :-
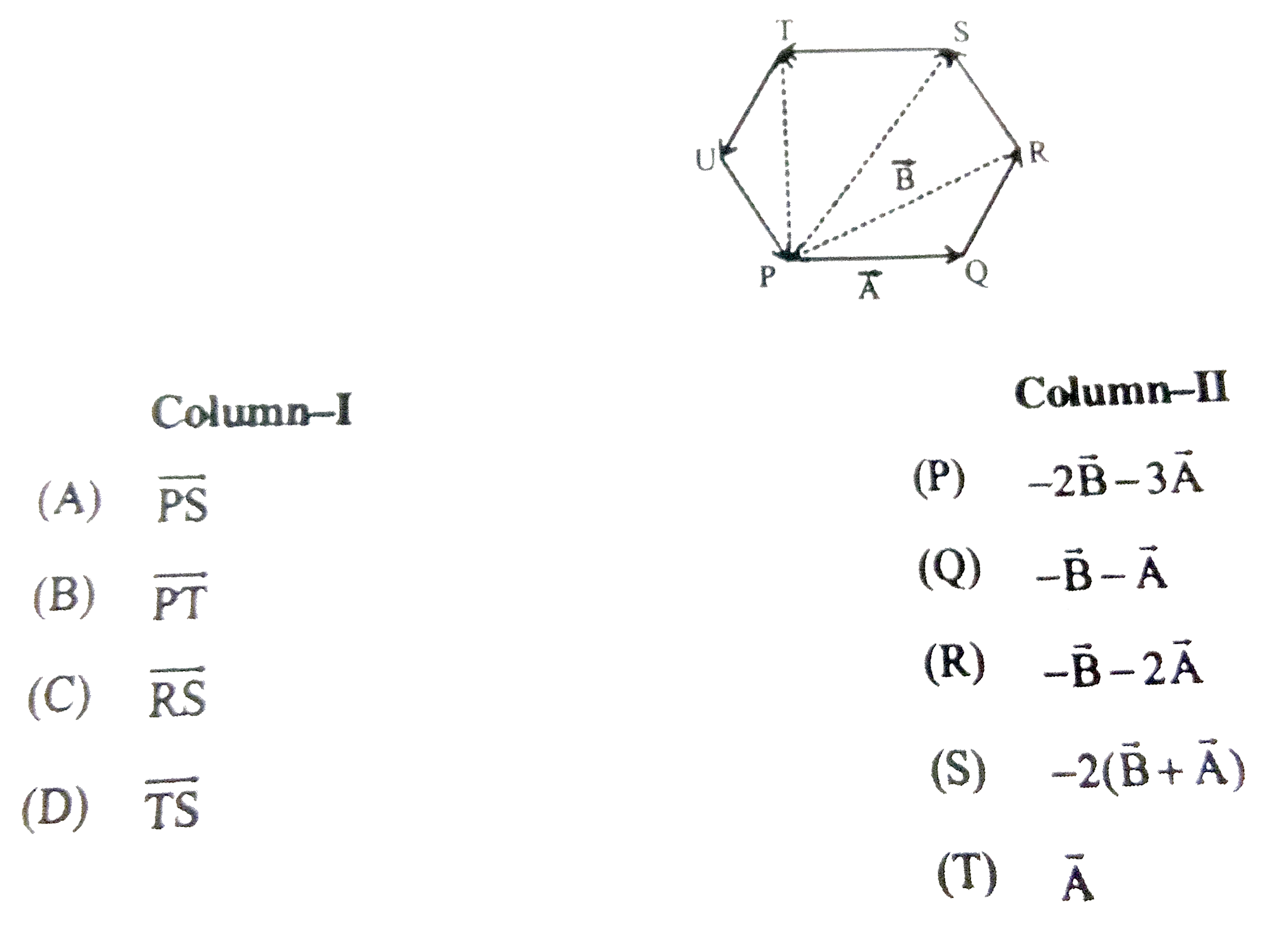
The correct Answer is:(A)-(S);(B)-(P);(C)-(R);(D)-(T)
Step by step video, text & image solution for In a regular hexagon two vec(PQ)=vecA, vec(RP)=vecB. Express other vectors's in term of them :- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-2) Single Correct Type Questions.17videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-2) Multiple Correct Type Questions.7videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) ParagraphType Questions.2videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHS18videos
TEST PAPERS
- BOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Previous Year Questions)7videos
WAVE OPTICS
Knowledge Check
- Question 1 - Select One
In a regualr hexagon ABCDEF, A→B=→a,B→C=→bandC→D=→c. Then A→E=
A→a+→b+→cB2→a+→b+→cC→a+→cD→a+2→b+2→c - Question 1 - Select One
Let O be the origin , and −−→OX,−−→OY,−−→OZ be three unit vector in the directions of the sides −−→OR,−−→RP,−−→PQ respectively, of a triangle PQR, Then , ∣∣∣−−→OX×−−→OY∣∣∣ =
Asin ( P +Q)Bsin 2RCsin (P +R)Dsin (Q +R) - Question 1 - Select One
Let O be the origin and−−→OX,−−→OY,−−→OZ be three unit vector in the directions of the sides −−→QR,−−→RP,−−→PQ respectively , of a triangle PQR.
∣∣∣−−→OX×−−→OY∣∣∣=Asin (P + Q)Bsin 2RCsin (P+R)Dsin (Q+R) - Question 1 - Select One
Let the vectors −−→PQ,−−→QR,−−→RS,−→ST,−−→TU and −−→UP represent the sides of a regular hexagon.
Statement I:−−→PQ×(−−→RS+−→ST)≠→0
Statement II: −−→PQ×−−→RS=→0 and −−→PQ×−−→RS=→0 and −−→PQ×−→ST≠→0
For the following question, choose the correct answer from the codes (A), (B) , (C) and (D) defined as follows:AStatement I is true , statement II is also true, Statement II is the correct explanation of statement I.Bstatement I is true, statement II is also true, statement II is not the corret explanation of statement I.CStatement I is false, statement II is false.DStatement I is false, statement II is true.
Similar Questions
In a regular hexagon ABCDEF, −−→AE
View SolutionABCDEF is a regular hexagon. Find the vector →AB+→AC+→AD+→AE+→AF in terms of the vector →AD
View SolutionIf ABCDEF is a regular hexagon with −−→AB=→a and −−→BC=→b, then −−→CE equals
View SolutionIn a regular hexagon ABCDEF, →AB=a,→BC=band→CD=c.Then,→AE=
View SolutionIn trapezium PQRS, given that QR∣∣PSand2QR=PS.If−−→PQ=→a,−−→QR=→band−−→RS=→c, express →q in terms →band→c
View SolutionLet ABCDEF be a regular hexagon and −−→AB=→a,−−→BC=→b,−−→CD=→c then −−→AE is
View Solution

