Two charges -q each are separated by dsitance 2d. A third charge +q is kept at mid-point O. find potential energy of +q as function of small distance x from 0 due to -q charges. Sketch PE Vs/x and convince yourself that the charge at 0 is in an unstable equilibrium.
let third charge +q is slightly displaced from mean position towards first charge. So, the total potential energy of the system is given by
U=14πε0{−q2(d−x)+−q2(d+x)}
U=−q24πε02d(d2−x2)
dUdx=−q22d4πε0⋅2x(d2−x2)2
The system will be in equilibrium, if
F=−dUdx=0
On solving, x=0 so for +q charge to be in stable/unstable equilibrium, finding second derivative of PE.
d2Udx2=(−2dq24πε0)[2(d2−x2)2−8x2(d2−x2)3]
=(2−dq24πε0)1(d2−x2)3[2(d2−x2)2−8x2]
at x=0
d2Udx2=(−2dq24πε0)(1d6)(2d2), which is lt0
This shows that system will be unstable equilibrium.
Topper's Solved these Questions
Similar Questions
Two charges -q each are separated by dsitance 2d. A third charge +q is kept at mid-point O. find potential energy of +q as function of small distance x from 0 due to -q charges. Sketch PE Vs/x and convince yourself that the charge at 0 is in an unstable equilibrium.
View SolutionTwo charges −q each are separated by distance 2d. A third charge +q is kept at mid-point O. Find potential energy of +q as a function of small distance x from O due to −q charges. Prove that the charge at O is in an unstable equilibrium.
View Solution
Knowledge Check
Two point charges Q and −Q/4 are separated by a distance X. Then
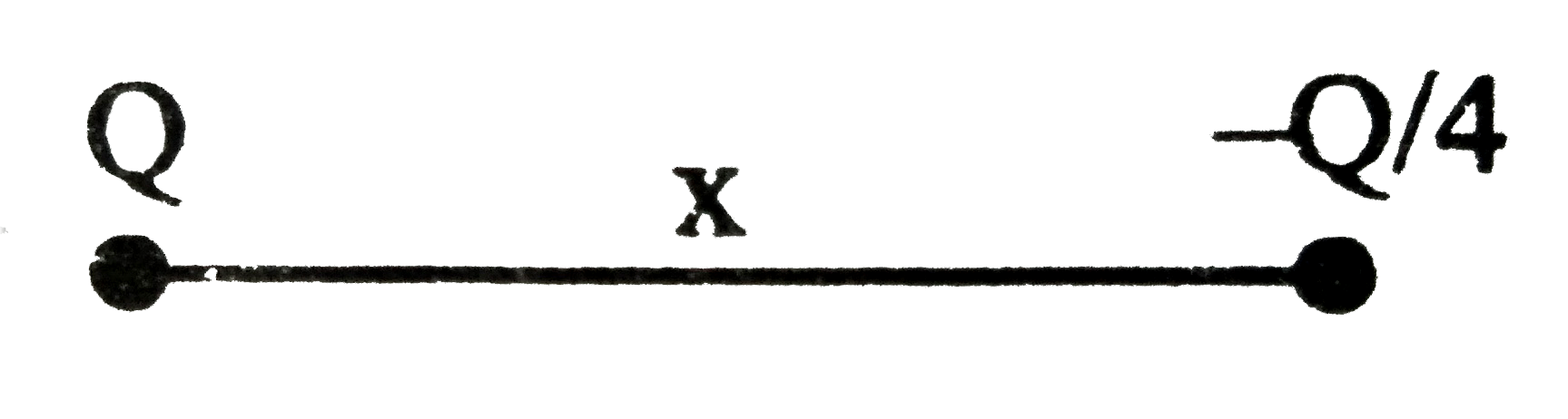 .Apotential is zero at a point on the axis which is x/3 on the right side of the cahrge −q/4Bpotential is zero at a point on the axis which is x/5 on the left side fo the charge −Q/4Celectric field is zero at a point on the axis which is at a distance x on the right side fo the charge −Q/4Dthere exist two points on the axis where electric field is zero.
.Apotential is zero at a point on the axis which is x/3 on the right side of the cahrge −q/4Bpotential is zero at a point on the axis which is x/5 on the left side fo the charge −Q/4Celectric field is zero at a point on the axis which is at a distance x on the right side fo the charge −Q/4Dthere exist two points on the axis where electric field is zero.Two point charges q each are fixed at (a,0) and (-a,0). A third charge Q is placed at origin. Electrons potential energy of the system will
Aincrease if Q is slightly displaced along x-axisBdecrease if Q is slightly displaced along x-axisCincrease if Q is slightly displaced along y-axisDdecrease if Q is slightly displaced along y-axisTwo unlike charges of magnitude q are separated by a distance 2d. The potential at a point midway between them is
AZeroB14πε0C14πε0.qdD14πε0.2qd2
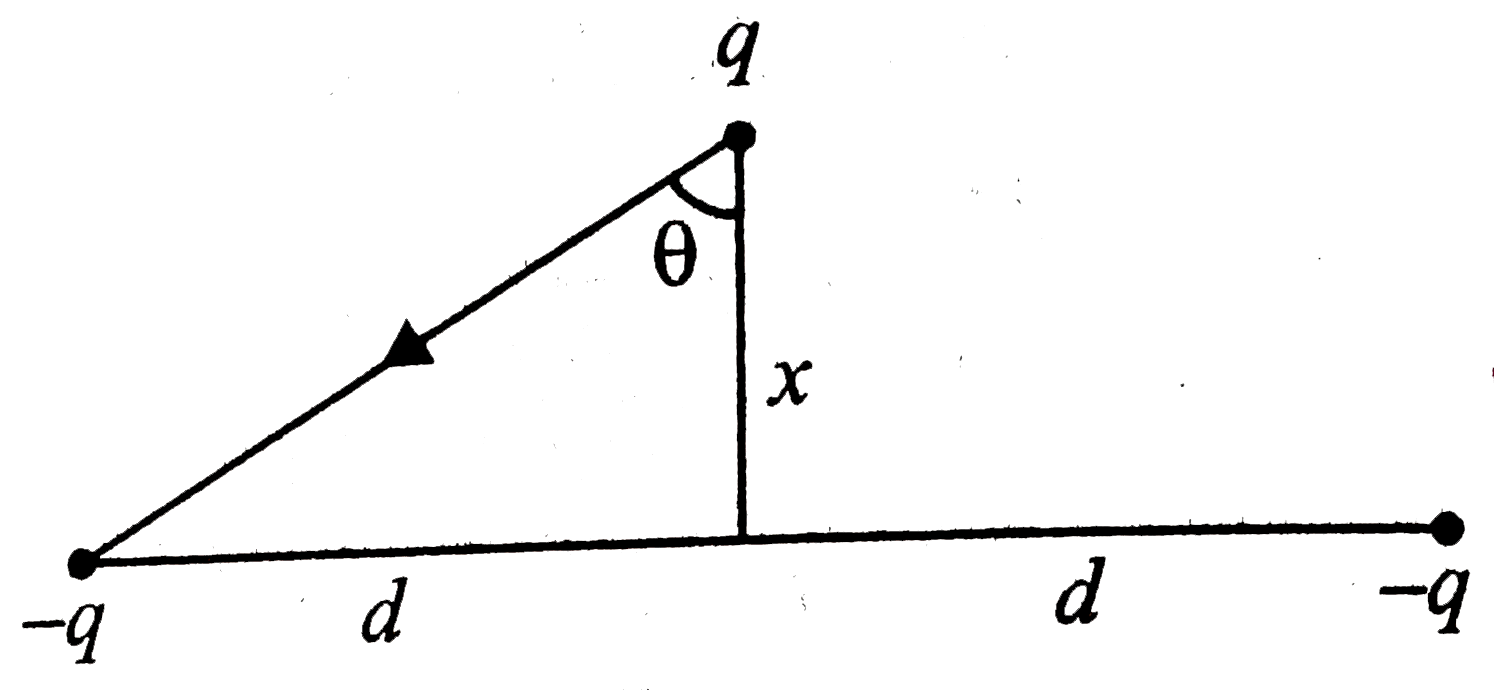
Two charges −q each are fixed separated by distance 2d. A third charge q of mass m placed at the mid-point is displaced at the mid-point is placed slightly by x(x<<d) perpendicular to the line joining the two fixed charges as shown in Fig. Show that q will perform simple harmonic oscillarion of time period.
T=[8π3∈0md3q2]1/2
View SolutionTwo free charges q and 4q are placed at a distance d apart A third charge Q is placed between them at a distance x from charge q such that the system is in equilibrium. Then
View SolutionTwo point charge (Q each) are placed at (0,y) and (0,-y). A point charge q of the same polarity can move along X-axis. Then :
View SolutionTwo charges of equal magnitude q are placed in air at a distance 2a apart and third charge −2q is placed at mid-point . The potential energy of the system is (ε0 = permittivity of free space)
View SolutionTwo point charges (Q each are placed at (0,y) and (0-y) A point charge q of the sme polarity can move along the x-axis. Then
View Solution
