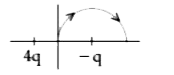
Two charges 4q and -q are kept on x-axis at (−d2,0) and (d2,0) resp. Then charge +q is moved from origin to x=d via semicircular path . Find change in its energy.
The correct Answer is:A, C, D
To find the change in energy when a charge +q is moved from the origin to x = d via a semicircular path in the presence of two other charges (4q at (-d/2, 0) and -q at (d/2, 0)), we can follow these steps:
Step 1: Understand the Initial Configuration
We have three charges:
- Charge 1: 4q located at (−d/2,0)
- Charge 2: −q located at (d/2,0)
- Charge 3: +q initially at the origin (0,0)
Step 2: Calculate Initial Potential Energy (E1)
The potential energy of a system of point charges is given by the formula:
U=kq1q2r
where k is Coulomb's constant, q1 and q2 are the magnitudes of the charges, and r is the distance between them.
1. Potential Energy between Charge +q and Charge 4q:
- Distance from +q (at origin) to 4q (at -d/2) is d/2.
- Potential Energy U1=k4q⋅qd/2=8kq2d.
2. Potential Energy between Charge +q and Charge -q:
- Distance from +q (at origin) to -q (at d/2) is d/2.
- Potential Energy U2=k−q⋅qd/2=−2kq2d.
3. Total Initial Potential Energy (E1):
E1=U1+U2=8kq2d−2kq2d=6kq2d
Step 3: Calculate Final Potential Energy (E2)
Now we need to calculate the potential energy when the charge +q is moved to x=d.
1. Potential Energy between Charge +q (at d) and Charge 4q:
- Distance from +q (at d) to 4q (at -d/2) is d+d/2=3d2.
- Potential Energy U3=k4q⋅q3d/2=8kq23d.
2. Potential Energy between Charge +q (at d) and Charge -q:
- Distance from +q (at d) to -q (at d/2) is d−d/2=d2.
- Potential Energy U4=k−q⋅qd/2=−2kq2d.
3. Total Final Potential Energy (E2):
E2=U3+U4=8kq23d−2kq2d
To combine these, convert −2kq2d to a common denominator:
−2kq2d=−6kq23d
Thus,
E2=8kq23d−6kq23d=2kq23d
Step 4: Calculate Change in Energy (ΔE)
The change in energy is given by:
ΔE=E2−E1
Substituting the values we found:
ΔE=2kq23d−6kq2d
To combine these, convert 6kq2d to a common denominator:
6kq2d=18kq23d
Thus,
ΔE=2kq23d−18kq23d=−16kq23d
Step 5: Final Result
The change in energy when the charge +q is moved from the origin to x=d is:
ΔE=−16kq23d
If we substitute k=14πϵ0, we have:
ΔE=−163⋅14πϵ0⋅q2d
We have three charges:
- Charge 1: 4q located at (−d/2,0)
- Charge 2: −q located at (d/2,0)
- Charge 3: +q initially at the origin (0,0)
The potential energy of a system of point charges is given by the formula:
U=kq1q2r
where k is Coulomb's constant, q1 and q2 are the magnitudes of the charges, and r is the distance between them.
- Distance from +q (at origin) to 4q (at -d/2) is d/2.
- Potential Energy U1=k4q⋅qd/2=8kq2d.
- Distance from +q (at origin) to -q (at d/2) is d/2.
- Potential Energy U2=k−q⋅qd/2=−2kq2d.
E1=U1+U2=8kq2d−2kq2d=6kq2d
Now we need to calculate the potential energy when the charge +q is moved to x=d.
- Distance from +q (at d) to 4q (at -d/2) is d+d/2=3d2.
- Potential Energy U3=k4q⋅q3d/2=8kq23d.
- Distance from +q (at d) to -q (at d/2) is d−d/2=d2.
- Potential Energy U4=k−q⋅qd/2=−2kq2d.
E2=U3+U4=8kq23d−2kq2d
To combine these, convert −2kq2d to a common denominator:
−2kq2d=−6kq23d
Thus,
E2=8kq23d−6kq23d=2kq23d
The change in energy is given by:
ΔE=E2−E1
Substituting the values we found:
ΔE=2kq23d−6kq2d
To combine these, convert 6kq2d to a common denominator:
6kq2d=18kq23d
Thus,
ΔE=2kq23d−18kq23d=−16kq23d
The change in energy when the charge +q is moved from the origin to x=d is:
ΔE=−16kq23d
If we substitute k=14πϵ0, we have:
ΔE=−163⋅14πϵ0⋅q2d
Topper's Solved these Questions
Similar Questions
Two point charges q and -2q are kept on x-axis at ( -a,0,0) and ( 2a,0,0) . Find the locus of all points wher electric potential is zero. Assume that electric potential at infinite distance from a point charge is zero.
View SolutionTwo charges q1andq2 are placed at (0,0,d) and (0,0,-d) respectively. Find locus of points where the potential is zero.
View Solution
Knowledge Check
A two point charges 4q and -q are fixed on the x - axis x=−d2andx=d2 , respectively. If a third point charge 'q' is taken from the origin to x = d along the semicircle as shown in the figure, the energy of the charge will
 Aincrease by 2q23πε0dBdecrease by 4q23πε0dCincrease by 3q24πε0dDdecrease by q24πε0d
Aincrease by 2q23πε0dBdecrease by 4q23πε0dCincrease by 3q24πε0dDdecrease by q24πε0dPoint charges +4q,−q and +4q are kept on the x-axis at points x=0,x=a and X=2a respectively, then
AOnly q is in stable equilibriumBNone of the charges are in equilibriumCAll the charges are in unstable equilibriumDAll the charges are in stable equlibriumTwo point charges +q and −q are held fixed at (−d,o) and (d,0) respectively of a x-y coordinate system. Then
A(a) The electric field E at all points on the x-axis has the same directionB(b) Electric field at all points on y-axis is along x-axisC(c) Work has to be done in bringing a test charge from ∞ to the originD(d) The dipole moment is 2qd along the x-axis
Two charges q1andq2 are placed at (0,0d)and(0,0−d) respectively. Find locus of points where the potential is zero.
View SolutionTwo charges Q1 and Q2 coulombs are shown in figure. A third charge Q3 coulombs is moved from points R to S along a circular path. Change in potential energy of the charge is -
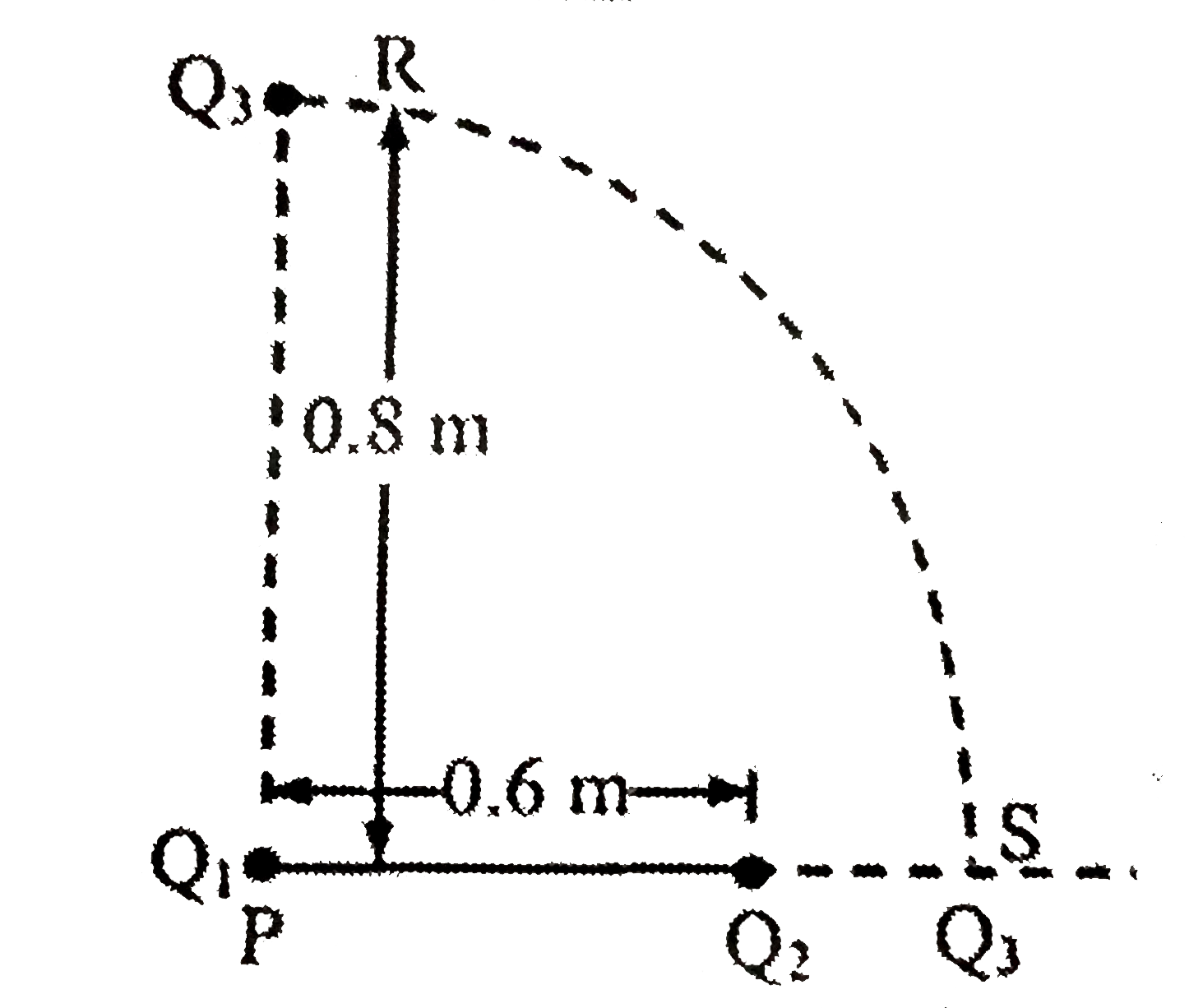
View SolutionTwo charges q and -q are placed at ( a,0) and (−a,0) on the x-axis. Another charge 2q is taken from ( 0,0) to ( 0,a) , then the work done to do so
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals

View Solutiontwo similar point charge + q are kept on x- axis at (-a,0)& (a,0) and another charge -Q of mass m Is kept at origin .If -Q si slightly displaced along y- axis & released them its time period of oscillation is -
View Solution
