$x_{1} = 5 sin ω t$
$x_{2} = 5 sin (ω t + 53^{\circ})$
$x_{3} = - 10 cos ω t$
Find amplitude of resultant SHM.

Video Solution

Text Solution

Verified by Experts

$x_{1} = 5 sin ω t$
$x_{2} = 5 sin (ω t + 53^{\circ})$
$x_{3} = - 10 cos ω t$
we can write $x_{3} = 10 sin (ω t + 270^{\circ})$
Finding the resultant amplitude by vactor notation.

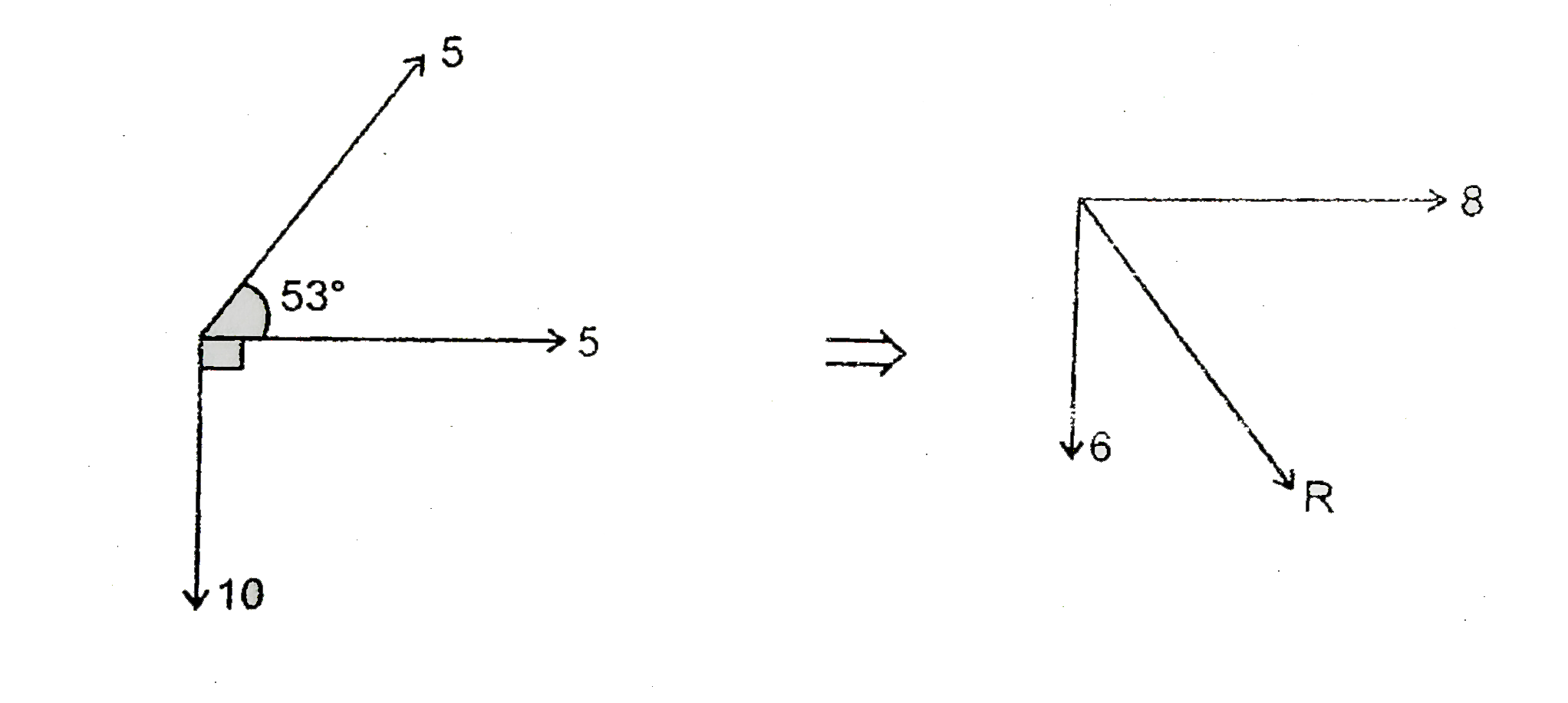
Resultant Amplitude $| R | = \sqrt{8^{2} + 6^{2}} = 10$ Ans.

Updated on:21/07/2023

|

Class 12 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Board Level Exercise
24 Videos
SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - I
25 Videos
SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos

Similar Questions

Knowledge Check

Find the resultant amplitude of the following simple harmonic equations $:$
$x_{1} = 5 sin ω t$
$x_{2} = 5 sin (ω t + 53^{\circ})$
$x_{3} = - 10 cos ω t$
A5
B10
C15
D20
If $i_{1} = 3 sin ω t and (i_{2}) = 4 cos ω t,$ then $(i_{3})$ is

A $5 sin (ω t + 53^{\circ})$
B $5 sin (ω t + 37^{\circ})$
C $5 sin (ω t + 45^{\circ})$
D $5 sin (ω t + 35^{\circ})$

RESONANCE-SIMPLE HARMONIC MOTION -Solved Miscellaneous Problems

Home
Ask Doubt
Profile