A rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
To find the speed of the center of mass of a rigid rod of mass m and length l when it is released from a horizontal position and becomes vertical, we can use the work-energy theorem. Here’s a step-by-step solution:
Step 1: Understand the System
The rod is pivoted at one end and is initially horizontal. When released, it rotates about the pivot due to the gravitational force acting on it.
Step 2: Identify the Work Done by Gravity
The work done by gravity as the rod moves from the horizontal to the vertical position can be calculated. The center of mass of the rod is located at a distance of l2 from the pivot.
The work done by gravity is given by:
W=mgh
where h is the vertical displacement of the center of mass. When the rod is horizontal, the center of mass is at a height h=l2. Thus, the work done by gravity is:
W=mg(l2)
Step 3: Apply the Work-Energy Theorem
According to the work-energy theorem, the work done by gravity is equal to the change in kinetic energy of the rod. Initially, the rod is at rest, so the initial kinetic energy is zero. The final kinetic energy when the rod is vertical can be expressed as:
KE=12Iω2
where I is the moment of inertia of the rod about the pivot and ω is the angular velocity.
Step 4: Calculate the Moment of Inertia
For a rod pivoted at one end, the moment of inertia I is given by:
I=13ml2
Step 5: Set Up the Equation
Setting the work done equal to the change in kinetic energy, we have:
mg(l2)=12(13ml2)ω2
Step 6: Simplify the Equation
Cancelling m from both sides and simplifying gives:
g(l2)=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Dividing both sides by l2:
ω2=3gl
Step 7: Find the Angular Velocity
Taking the square root gives:
ω=√3gl
Step 8: Calculate the Speed of the Center of Mass
The speed of the center of mass vcm is related to the angular velocity by:
vcm=ω⋅r
where r is the distance from the pivot to the center of mass, which is l2:
vcm=ω⋅(l2)=√3gl⋅(l2)
Thus,
vcm=l2√3gl=√3gl2
Final Answer
The speed of the center of mass of the rod when it becomes vertical is:
vcm=√3gl2
The rod is pivoted at one end and is initially horizontal. When released, it rotates about the pivot due to the gravitational force acting on it.
The work done by gravity as the rod moves from the horizontal to the vertical position can be calculated. The center of mass of the rod is located at a distance of l2 from the pivot.
W=mgh
where h is the vertical displacement of the center of mass. When the rod is horizontal, the center of mass is at a height h=l2. Thus, the work done by gravity is:
W=mg(l2)
According to the work-energy theorem, the work done by gravity is equal to the change in kinetic energy of the rod. Initially, the rod is at rest, so the initial kinetic energy is zero. The final kinetic energy when the rod is vertical can be expressed as:
KE=12Iω2
where I is the moment of inertia of the rod about the pivot and ω is the angular velocity.
For a rod pivoted at one end, the moment of inertia I is given by:
I=13ml2
Setting the work done equal to the change in kinetic energy, we have:
mg(l2)=12(13ml2)ω2
Cancelling m from both sides and simplifying gives:
g(l2)=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Dividing both sides by l2:
ω2=3gl
Taking the square root gives:
ω=√3gl
The speed of the center of mass vcm is related to the angular velocity by:
vcm=ω⋅r
where r is the distance from the pivot to the center of mass, which is l2:
vcm=ω⋅(l2)=√3gl⋅(l2)
Thus,
vcm=l2√3gl=√3gl2
The speed of the center of mass of the rod when it becomes vertical is:
vcm=√3gl2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBook:FIITJEEChapter:ROTATIONAL MECHANICSExercise:SOLVED PROBLEMS (SUBJECTIVE)
Explore 10 Videos
- ROTATIONAL MECHANICSBook:FIITJEEChapter:ROTATIONAL MECHANICSExercise:SOLVED PROBLEMS (OBJECTIVE)
Explore 14 Videos
- PHYSICS PART2Book:FIITJEEChapter:PHYSICS PART2Exercise:Numerical Based Question Decimal Type
Explore 6 Videos
- SEMICONDUCTOR AND DEVICEBook:FIITJEEChapter:SEMICONDUCTOR AND DEVICEExercise:SOLVED PROBLEMS Objective: Level-I
Explore 20 Videos
Similar Questions
A rod of mass m and length l is held vertically on a smooth horizontal floor. Now it is released from this position, find the speed of its centre of mass when it makes an angle θ with the vertical.
View SolutionA rod of mass m and length l is himged about one of its ends. The rod is released from horizontal position. When the rod becomes vertical, calculate (i) angular speed of the rod (ii) Hinge reaction.
View Solution
Knowledge Check
A metal rod of length l pivoted at is upper end. It is released from a horizontal position. There is a uniform magnetic field ⊥ to its plane of rotation. When it becomes vertical, the p.d. across its ends is proportional to
Al1/2BlCl3/2Dl2A rod of mass m and length l is hinged at 'its' end and released from rest in position shown. Find the force exerted by the hinge when the rod becomes horizontal
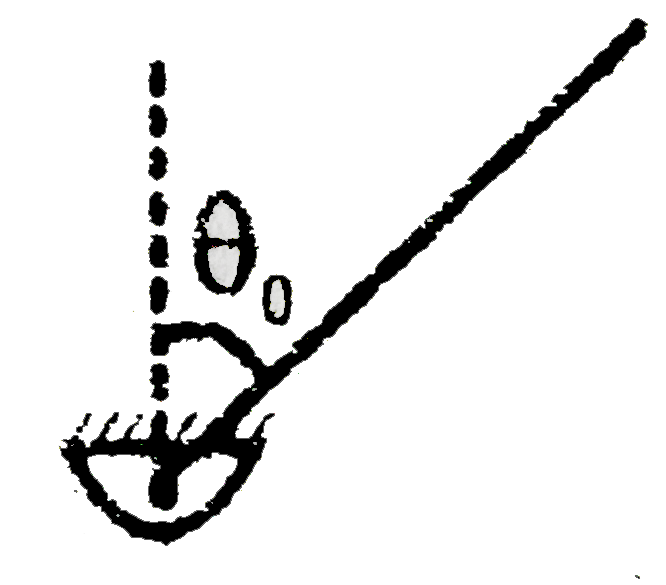 Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14
Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14One end of a uniform rod of length l and mass m is hinged at A. It is released from rest from horizontal position AB as shown in figure. The force exerted by the rod on the hinge when it becomes vertical is
 A32mgB52mgC3mgD5mg
A32mgB52mgC3mgD5mg
A uniform slender rod of mass M and length L is pivoted at its end. The rod is released from its nearly vertical position. What is the reaction at the pivot when the rod reaches the horizontal position? If the support is withdrawn at this instant, describe the subsequent motion of the rod.
View SolutionA uniform rod of mass M and length L is hinged at its end. The rod is released from its vertical position by slightly pushing it. What is the reaction at the hinge when the rod becomes horizontal, again vertical.
View SolutionAB is a mass less rigid rod of length 2l. It is free to rotate in vertical plane about a horizontal axis passing through its end A. Equal point masses (m each) are stuck at the centre C and end Bof the rod. The rod is released from horizontal position. Write the tension in the rod when it becomes vertical.
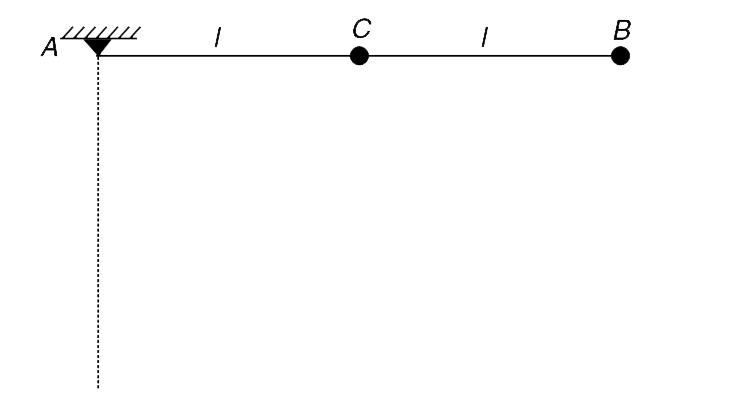
View SolutionA rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.

View SolutionA uniform rod smoothly pivoted at one of its ends is released from rest. If it swings in vertical plane, the maximum speed of the end P of the rod is.
 .
.
View Solution