A water tank is 20 m deep . If the water barometer reads 10 m at the surface , then what is the pressure at the bottom of the tank in atmosphere ?
To find the pressure at the bottom of a water tank that is 20 meters deep, given that the barometer reads 10 meters at the surface, we can follow these steps:
Step 1: Understand the Problem
We have a water tank that is 20 meters deep. The barometer reading at the surface indicates that the atmospheric pressure is equivalent to a 10-meter column of water.
Step 2: Identify the Variables
- Depth of the water tank (h) = 20 m
- Height of the water column indicated by the barometer (H) = 10 m
- Density of water (ρ) = 1000 kg/m³ (standard value)
- Acceleration due to gravity (g) = 9.81 m/s² (standard value)
Step 3: Calculate the Atmospheric Pressure (P0)
The atmospheric pressure at the surface can be calculated using the height of the water column indicated by the barometer:
P0=ρ⋅g⋅H
Substituting the values:
P0=1000kg/m3⋅9.81m/s2⋅10m
P0=98100Pa
Step 4: Calculate the Pressure at the Bottom of the Tank (PA)
The pressure at the bottom of the tank (P_A) can be calculated using the formula:
PA=P0+ρ⋅g⋅h
Where:
- h = 20 m (depth of the tank)
Substituting the values:
PA=98100Pa+(1000kg/m3⋅9.81m/s2⋅20m)
PA=98100Pa+196200Pa
PA=294300Pa
Step 5: Convert Pressure to Atmospheres
To convert the pressure from Pascals to atmospheres, we use the conversion factor:
1atm=101325Pa
So,
PA (in atm)=PA (in Pa)101325
PA (in atm)=294300101325≈2.9atm
Final Answer
The pressure at the bottom of the tank is approximately 2.9 atm.
---
We have a water tank that is 20 meters deep. The barometer reading at the surface indicates that the atmospheric pressure is equivalent to a 10-meter column of water.
- Depth of the water tank (h) = 20 m
- Height of the water column indicated by the barometer (H) = 10 m
- Density of water (ρ) = 1000 kg/m³ (standard value)
- Acceleration due to gravity (g) = 9.81 m/s² (standard value)
The atmospheric pressure at the surface can be calculated using the height of the water column indicated by the barometer:
P0=ρ⋅g⋅H
Substituting the values:
P0=1000kg/m3⋅9.81m/s2⋅10m
P0=98100Pa
The pressure at the bottom of the tank (P_A) can be calculated using the formula:
PA=P0+ρ⋅g⋅h
Where:
- h = 20 m (depth of the tank)
PA=98100Pa+(1000kg/m3⋅9.81m/s2⋅20m)
PA=98100Pa+196200Pa
PA=294300Pa
To convert the pressure from Pascals to atmospheres, we use the conversion factor:
1atm=101325Pa
So,
PA (in atm)=PA (in Pa)101325
PA (in atm)=294300101325≈2.9atm
The pressure at the bottom of the tank is approximately 2.9 atm.
Topper's Solved these Questions
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - ASSIGHNMENT PROBLEMS (SUBJECTIVE ) SHORT ANSWER TYPE QUESTIONS (LEVEL -I)
4 Videos
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - ASSIGHNMENT PROBLEMS (SUBJECTIVE ) FILL IN THE BLANKS (LEVEL -I)
5 Videos
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - MATRIX MATCH TYPES
6 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
- GENERAL PHYSICSBOOK - FIITJEECHAPTER - GENERAL PHYSICSEXERCISE - MATRIX MATCH TYPE
3 Videos
Similar Questions
P is the atmospheric pressure and the pressure at the bottom of a tank of water is 3P. If the water is drawn out to lower the level of water by one fifth, then the pressure at the bottom of the tank will be
View SolutionThe pressure at the bottom of a water tank is 4 P, where P is .atmospheric pressure. If water is drawn out till the water level decreases by —35th, then pressure at the bottom of the tank is
View Solution
Knowledge Check
P is the atmospheric pressure and the pressure at the bottom of a tank of water is 3P. If the water is drawn out to lower the level of water by one fifth, then the pressure at the bottom of the tank will be
A2PB(13/5)PC(8/5)PD(4/5)PThe pressure at the bottom of an open tank of water is 3p where p is the atmospheric pressure. If the water is drawn out till the level of water remains one fifth, the pressure at the bottom of the tank will now be
A2pB(13/5)pC(7/5)pD(4/5)pA hole is made at the bottom of the tank filled with water (density =1000kgm−3). If the total pressure at the bottom of the tank is three atmospheres (1 atmosphere =105Nm−2), then the velocity of efflux is nearest to
A√400ms−1B√200ms−1C√600ms−1D√500ms−1
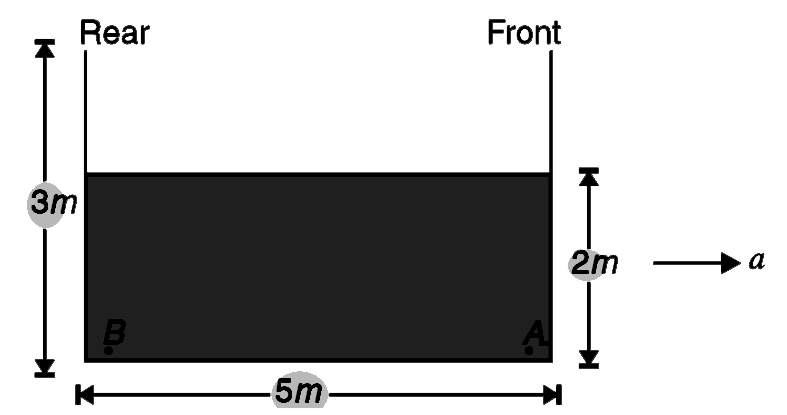
An open rectangular tank 5mxx4mxx3m in dimension is containing water up to a height of 2m the tank is accelerated horizontally along the longer side. Assuming water to be and ideal liquid find-
(a) the maximum acceleration with which the tank can be moved so that water does not fall from the rear side.
(b) the gauge pressure at the bottom of the front and back of the tank (points A and B) if the tank is closed at the top and is then acclerated horizontally at 9m/s2. Assume that the top cover has a small hole at the right side of the tank so taht pressure of air inside the tank is maintained at atmospheric pressure. Gauge pressure at a point is difference in absolute pressure at the point and atmospheric pressure.
View SolutionWater is filled in a tank upto a height of 2m .A wooden cylinder of weight 10kg is floating on the surface of water partially immersed.If cross sectional area of tank is 1m2 and that of cylinder is 0.5m2, then the total pressure (in Pa) at the bottom of tank is (Po=atmospheric pressure )
View SolutionA tank 5 m high is half filled with water and then is filled to the top with oil of density 0.85 g The pressure at the bottom of the tank, due to these liquids is
View SolutionA tank 5 m high is half filled with water and then is filled to top with oil of density 0.85g/cm3 The pressure at the bottom of the tank, due to these liquids is
View SolutionA primitive diving bell consists of a cylindrical tank with one end open and one end closed. The tank is lowered into a freshwater lake, open end downward. Water rises into the tank, compressing the trapped air, whose temperature remains constant during the descent. The tank is brought to a halt when the distance between the surface of the water in the tank and the surface of the lake is 40.0 m. Atmospheric pressure at the surface of the lake is 1.01×105 Pa. Find the fraction of the tank's volume that is filled with air.
View Solution
