Question

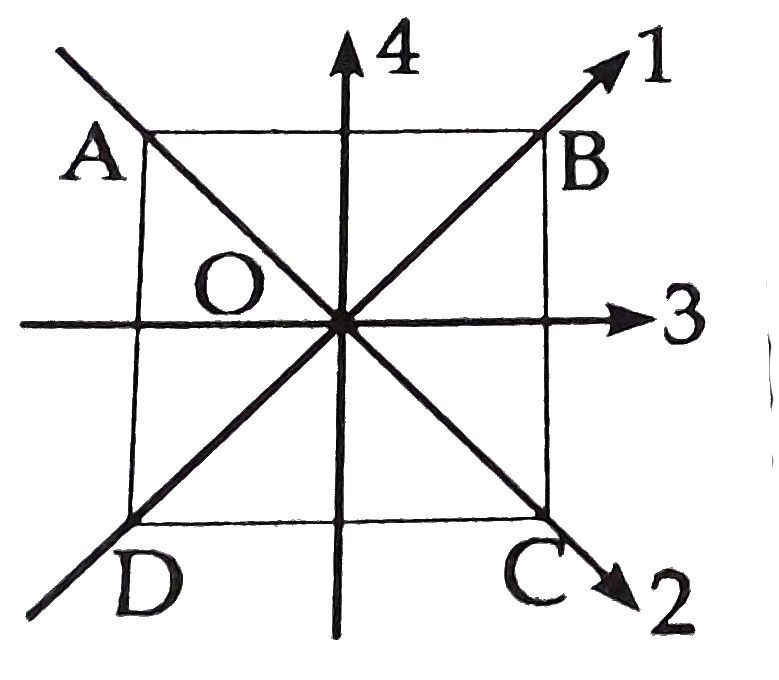
Let l be the moment of inertia of a uniform square plate about an axis AB that passes through its centre and is parallel to two of its sides. CD is a line in the plane of the plate that passes through the centre of the plate and makes an angle $\theta$ with AB. The moment of inertia of the plate about the axis CD is then equal to

Options

A. I
B. I `sin^(2) theta `
C. `I cos^(2) theta`
D. `I cos^(2) (theta/2)`.

Answer

A

Explanation

In the figure, `A^(')B^(')` is perpendicular to AB
`C^(')D^(')` is perpendicular to CD
By symmetry, `I_(AB) = I_(A^(')B^('))`
`I_(CD) = I_(C^(')D^('))`
By theorem of perpendicular axes,
`I_(Z) = I_(AB) + I_(A^(')B^(')) = 2I_(AB)` .............(i)
Again, `I_(Z) = I_(CD) + I_(C^(')D^(')) = 2I_(CD)` ..............(ii)
`therefore` From (i) and (ii),
`2I_(AB) = 2I_(CD)` or `I_(AB) = I_(CD)` or `I= I_(CD)`
Hence moment of inertia about CD axis= I.