Question

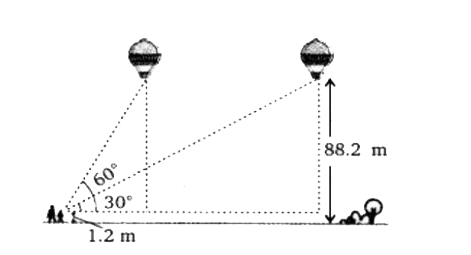
A 1.2 m tall girl spots a ballon moving with wind in a horizontal line at a height of 88.2 m from the ground. The angle of elevation of the balloon from the eyes of the girl at any instant is ${60}^{\circ }$. After some time, the angle of elevation reduces to ${30}^{\circ }$. Find the distance travelled by the balloon during the interval.

Explanation

To solve the problem step by step, we will use trigonometric principles to find the distance traveled by the balloon.

Step 1: Understand the scenario
The girl is 1.2 m tall, and the balloon is at a height of 88.2 m from the ground. The height of the balloon above the girl's eyes is:
\( \text{Height of the balloon above the girl's eyes} = 88.2 \, \text{m} - 1.2 \, \text{m} = 87 \, \text{m} \)

Step 2: Set up the first triangle
When the angle of elevation is \(60^\circ\), we can set up a right triangle where:
- The height (perpendicular) from the girl's eye level to the balloon is 87 m.
- The angle of elevation is \(60^\circ\).

Using the tangent function:
\( \tan(60^\circ) = \frac{\text{opposite}}{\text{adjacent}} = \frac{87}{x} \)
Where \(x\) is the horizontal distance from the girl to the point directly below the balloon.

Step 3: Solve for \(x\)
From the tangent of \(60^\circ\):
\( \tan(60^\circ) = \sqrt{3} \implies \sqrt{3} = \frac{87}{x} \)
Rearranging gives:
\( x = \frac{87}{\sqrt{3}} = 29\sqrt{3} \, \text{m} \)

Step 4: Set up the second triangle
When the angle of elevation reduces to \(30^\circ\), we set up another triangle:
- The height remains 87 m.
- The angle of elevation is \(30^\circ\).

Using the tangent function again:
\( \tan(30^\circ) = \frac{87}{y} \)
Where \(y\) is the new horizontal distance from the girl to the point directly below the balloon.

Step 5: Solve for \(y\)
From the tangent of \(30^\circ\):
\( \tan(30^\circ) = \frac{1}{\sqrt{3}} \implies \frac{1}{\sqrt{3}} = \frac{87}{y} \)
Rearranging gives:
\( y = 87\sqrt{3} \, \text{m} \)

Step 6: Calculate the distance traveled by the balloon
The distance traveled by the balloon is the difference between the two horizontal distances:
\( \text{Distance traveled} = y - x = 87\sqrt{3} - 29\sqrt{3} = (87 - 29)\sqrt{3} = 58\sqrt{3} \, \text{m} \)

Final Answer
The distance traveled by the balloon during the interval is:
\( \boxed{58\sqrt{3} \, \text{m}} \)