AB and CD are two slabs. The medium between the slabs has refractive index 2 . Find the minium angle of incidence of Q , so that the ray is totally reflected by both the slabs .
_E01_252_Q01.png)
The correct Answer is:60∘
Critical anglest at 1 and 2
_E01_252_S01.png)
θC1=sin−1(μ1μ2)=sin−1(1√2)=45∘
θC2=sin−1(μ3μ2)=sin−1(√32)=60∘
Therefore , minimum angle of incidence for total internal reflection to take place on both slabs should be 60∘
imin=60∘
Topper's Solved these Questions
Similar Questions
AB and CD are surfaces ot two slabs as shown in Figure . The medium between the slabs has refractive indes 2. Refractive indes of the slab above Ab is √2 and below CD is √3 . Find the minimum angle of incidence at Q, so that the ray is totally reflected by both the slabs.

View SolutionAB and CD are surfaces of two slabs as shown in figure. The medium between the slabs has refractive index 2, refractive index of slab above AB is √2 and below CD is √3. Find the minimum angle of incidence, so that the ray is totally reflected by both the slabs.

View Solution
Knowledge Check
A ray of light falls on a transparent glass slab of refractive index 1.62. What is the angle of incidence if the reflected ray and refracted ray are mutually perpendicular?
Atan−1(1.62)Btan−1(11.162)C1tan−1(1.62)Dnone of theseA ray of light strikes a tansparent rectangular slab (of refractive index √2) At an angle of incidence of 45∘. The angle between the reflected and refracted rays is
A75∘B90∘C105∘D120∘A ray of light strikes a transparent rectangular slab of refractive index √2 at an angle of incidence of 45∘. The angle between the reflected and refracted rays is
A75∘B90∘C105∘D120∘
A rectangular slab ABCD, of refractive index n1 , is immersed in water of refractive index n2(n1<n2). A ray of light is incident at the surface AB of the slab as shown in Fig. Find the maximum value of angle of incidence αmax, such that the ray comes out only from the other surface CD.
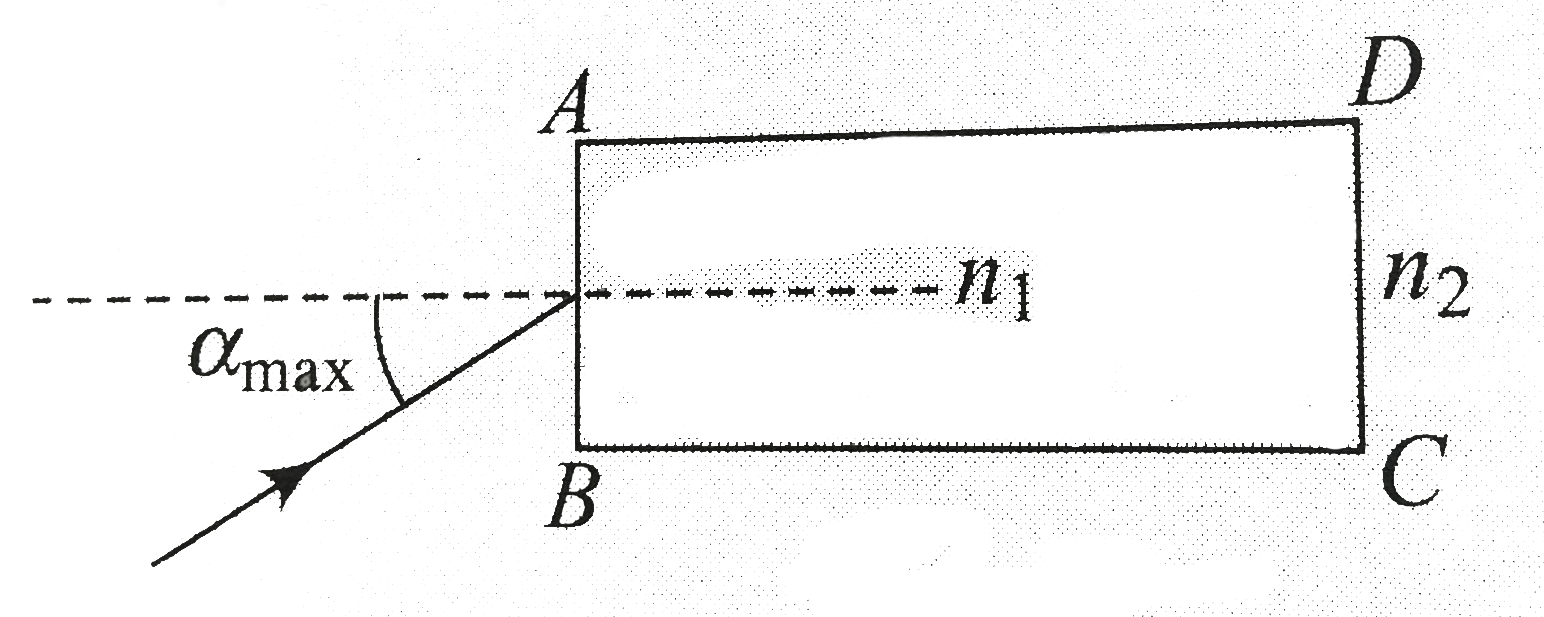
View SolutionA ray of light travelling in air is incident on a composite transparent slab at an angle of incidence i=45°. The composite slab consist of 100 parallel faced slices of equal thickness. The refractive index of the nth slice (counting from the one on which light is incident) is given by μn=1.0+0.01n. The medium on the other side of the slab has refractive index of √2 . Calculate the angular deviation suffered by the ray as it comes out of the slab.
View SolutionGive a formula to find refractive index of a glass slab in terms of angle of incidence and angle of refraction.
View SolutionA ray of light is incident on a parallel slab of thickness t and refractive index n. If the angle of incidence θ is small, than the lateral displacement in the incident and emergent ray will be
View SolutionWhen light rays are incident on a thick transparent slab then these rays are partly reflected and partly refracted into the slab. At a certain angle of incidence θ, it is found that reflected and refracted rays are mutually perpendicular. What is refractive index of material ?
View Solution
