Question

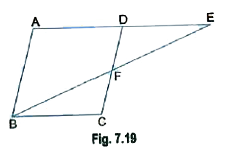
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that $\Delta ABE~\Delta CFB$.

Explanation

To show that triangle ABE is similar to triangle CFB, we will use the Angle-Angle (AA) similarity criterion. Here’s a step-by-step solution:

Step 1: Identify the triangles
We have two triangles to consider: triangle ABE and triangle CFB.

Step 2: Identify the angles in triangle ABE
- Angle BAE (∠BAE) is one angle in triangle ABE.
- Angle AEB (∠AEB) is another angle in triangle ABE.

Step 3: Identify the angles in triangle CFB
- Angle CBF (∠CBF) is one angle in triangle CFB.
- Angle CFB (∠CFB) is another angle in triangle CFB.

Step 4: Show that angle BAE is equal to angle BCF
Since ABCD is a parallelogram, opposite angles are equal. Therefore:
- ∠BAE = ∠BCF (opposite angles of the parallelogram).

Step 5: Show that angle CBF is equal to angle AEB
Since BC is parallel to AD (as opposite sides of a parallelogram), by the Alternate Interior Angle Theorem:
- ∠CBF = ∠AEB.

Step 6: Conclude the similarity of triangles
Now we have:
1. ∠BAE = ∠BCF
2. ∠AEB = ∠CBF

Thus, we can say:
- Triangle ABE ~ Triangle CFB by AA similarity criterion (since two angles of one triangle are equal to two angles of the other triangle).

Final Conclusion
Therefore, triangle ABE is similar to triangle CFB.

---