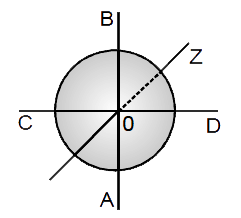
Find the moment of inertia, about a diameter, of a uniform ring of mass M = 8kg and radius a = 1m?

We know that the moment of inertia, Ioz, of the ring about OZ is Ma2. We also know that, from symmetry, the moment of inertia about any one diameter is the same as that about any other diameter, i.e., I(OX)=IOY Using the perpendicular axes theorem gives IOZ=IOX+IOY⇒Ma2=21OX=21OY The moment of inertia of the ring about any diameter =12Ma2=4kgm2.
Similar Questions
Knowledge Check
The moment of inertia of a thin uniform ring of mass I kg about an axis passing through the centre and perpendicular to the plane of the ring is 0.25 kg m2. Then the diameter of the ring is
A0.25mB0.5mC0.75mD1mMoment of inertia of a uniform circular ring of mass 2kg and diameter 1m about its diameter is
A0.25kgm2B0.5kgm2C1kgm2D2kgm2
Find the out the moment of inertia of a ring having uniform mass distribution of mass M and radius R about an axis which is tangent ot the ring and a in the plane of the ring b. perpendicular to the plane of the ring.
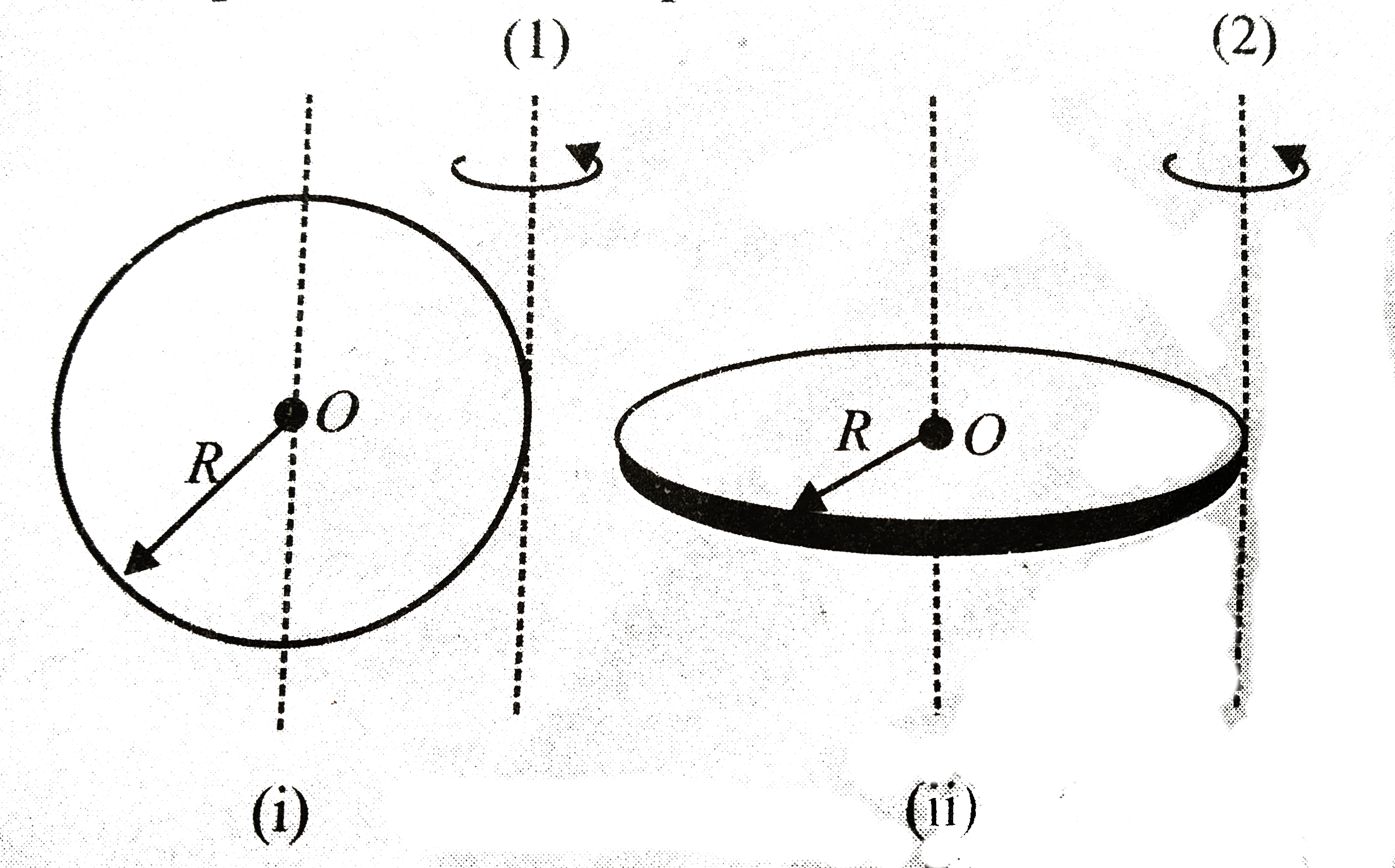
View SolutionFind the moment of inertia of a half uniform ring (mass m, radius r) about its center.
View SolutionFind the moment of inertia of a uniform sphere of mass m and radius R about a tangent if the spheres (1) solid (ii) hollow?
View SolutionUse the parallel axis theorem to find the moment of inertia ofa uniform rod of mass M = 3kg and length 2a (a = 1m), about a perpendicular axis through one end?
View SolutionWhat is the moment of inertia of a
(i) uniform circular ring of mass 2 kg about its 4 m diameter ?
(ii) a thin disc about an axis coinciding with a diameter ?
View SolutionCalculate moment of inertia of a uniform circular ring of mass 1.5kg and diameter 50cm about a diameter of the ring.
View Solution