Statement - 1 : If a concentric spheerical Gaussian surface is drawn inside thin spheical shell charge, electric field (E) at each point of surface must be zero.
Statement - 2 : In accordance with Gauss's law
ϕE=∮→E.d→A=Qnet enclosedε0
Qnet enclosed=0 implies ϕE=0
Video Solution
Text Solution
No, this →E field can be due to all the charges inside as well as outside.
|
Similar Questions
Knowledge Check
Statement-1: Suppose that a Gaussian surface enclose no net charge. Gauss law requires that electric field equals zero for all points on the surface.
Statement-2: If electric field equals zero everywhere on the surface, Gauss law requires that there be no net charge inside.AStatement-1 is true, statement-2 is true and statement-2 is correct explanation for statement-1.BStatement-1 is true, statement-2 is true and statement-2 is NOT the correct explanation for statement-1.CStatement-1 is true, statement-2 is false.DStatement -1 is false, statement -2 is true.If net charge enclosed by a Gaussian surface is zero, then
Assertion :- →E at any point on Gaussian surface is 0.
Reason :- No net charge is enclosed by Gaussian surface, so E = 0AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.Assertion(A):If Gaussian surface does not enclose any charge then →E at any point on the Gaussian surface must be zero.
Assertion(R ):No net charge is enclosed by Gaussians surface,so net flux passing through the surface is zero.ABoth A and R are true and R is the correct explanation of ABBoth A and R are True but R is not the correct explanation of A.CA is true and R is falseDA is false and R is true.
Assertion: Two charged metallic surfaces parallel to each other always carry equal and opposite charges.
Reason: Gauss's law: ∮→E⋅d→S=qInε0
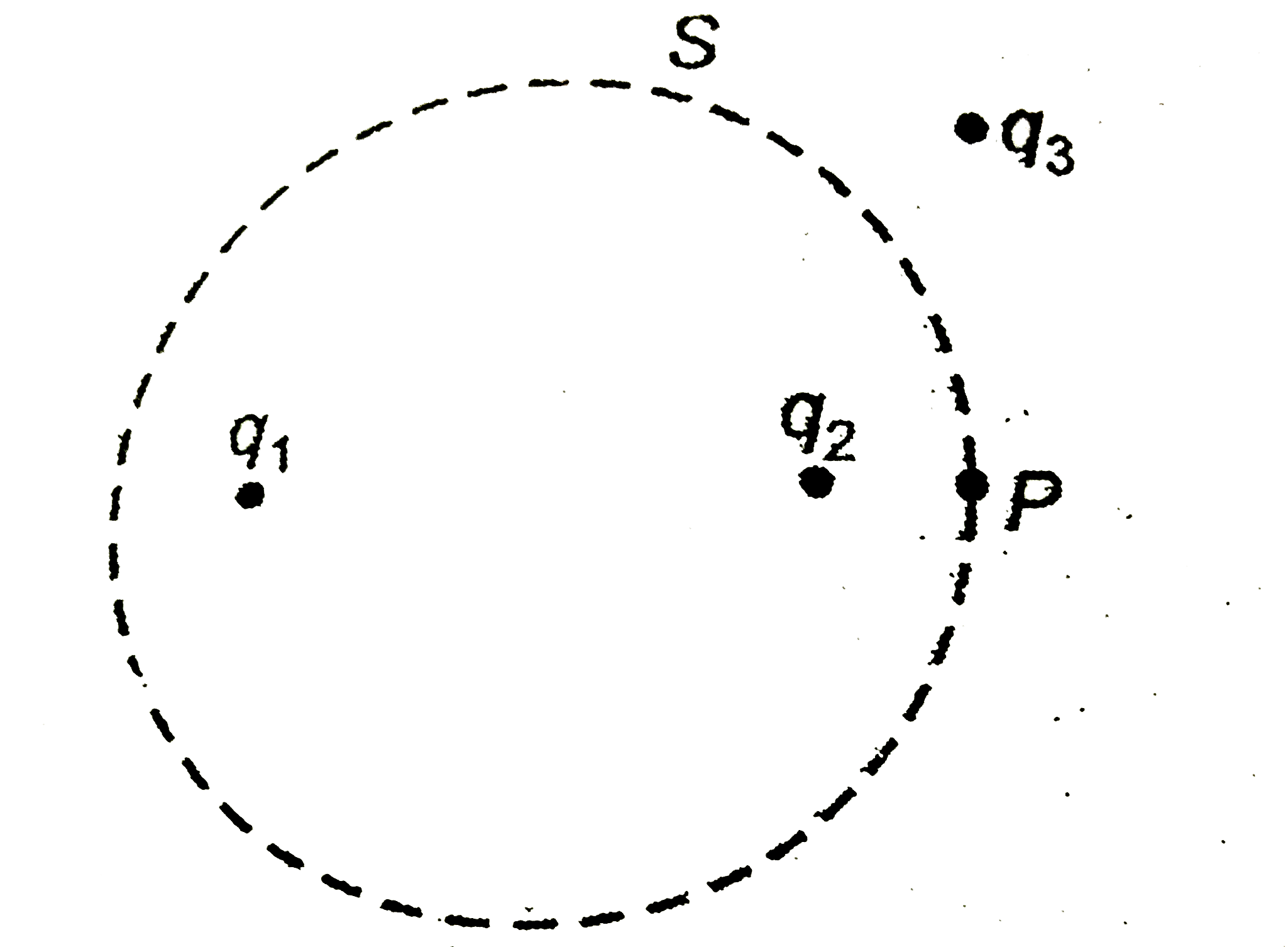
View SolutionThree point charges are placed as shown in the figure. S is a gaussian surface ϕ is the net flux passing through surface A and E be the electric field at point P on the surface, then
View SolutionForce →F acting on a test charge q0 in a uniform electric field →E is
View SolutionForce →F acting on a test charge q0 in a uniform electric field →E is
View SolutionA : We may have a Gaussian surface in which less number of field lines enter and more field lines come out.
R : The electric field E in the Gauss's law is only due to the enclosed charges.
View Solution