Statement-1: Suppose that a Gaussian surface enclose no net charge. Gauss law requires that electric field equals zero for all points on the surface.
Statement-2: If electric field equals zero everywhere on the surface, Gauss law requires that there be no net charge inside.
Statement-1 is true, statement-2 is true and statement-2 is correct explanation for statement-1.
Statement-1 is true, statement-2 is true and statement-2 is NOT the correct explanation for statement-1.
Statement-1 is true, statement-2 is false.
Statement -1 is false, statement -2 is true.
The correct Answer is:D
ϕ=0⇒q=0⇒∫→Ed→s=0 This does not necessarily implies that E=0.
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Multiple objective
Explore 131 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Subjective
Explore 107 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Comphrehension
Explore 134 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:PYQS AIEEE
Explore 10 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
If the total charge enclosed by a surface is zero, does it imply that the electric field everywhere on the surface is zero ? Conversely, if the electric field everywhere on a surface is zero, does it imply that net charge inside is zero.
View SolutionThe position of the point where the net electric field will be zero is
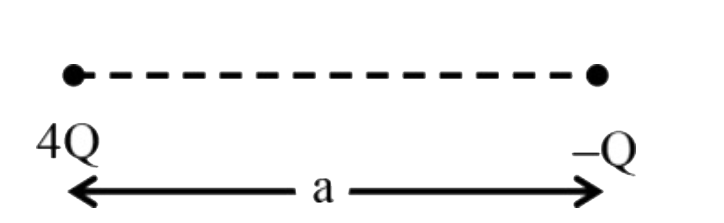
View Solution
Knowledge Check
The Gaussian surface needed for calculating the electric field due to a charge distribution is
Aany surface around the charge distributionBonly spherical surface.Cany closed surface around the charge.Donly cylindrical surface.If the net electric field flux passing through a closed surface is zero, then the electric field at the surface will be
A0Bsame at all placesCdepends upon the location of pointsDinfiniteA Gaussian surface enclosed no charge. Which of the following is true for a point inside it ?
Aelectric field must be zeroBelectric potential must be zeroCboth electric potential and intensity must be zeroDnone of these
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.
View SolutionShow that the electric field at the surface of a charged conductor is proportional to the surface charge density.
View SolutionIf the flux of the electric field through a closed surface is zero ,
(i) the electric field must be zero everywhere on the surface
(ii) the electric field may be zero everywhere on the surface
(iii) the charge inside the surface must be zero
(iv) the charge in the vicinity of the surface must be zero
View SolutionThe Gaussian surface for calculating the electric field due to a charge distribution is
View SolutionThe Gaussian surface for calculating the electric field due to a charge distribution is
View Solution