A simple pendulum is oscillating in a trolley moving on a horizontal straight road with constant acceleration a. If direction of motion of trolley is taken as positive x direction and vertical upward direction as positive y direction then the mean position of pendulum makes an angle
tan−1(ga) with y axis in +x direction
tan−1(ag) with y axis in -x direction
tan−1(ag) with y axis in +x direction
tan−1(ga) with y axis in -x direction
The correct Answer is:B
To solve the problem of a simple pendulum oscillating in a trolley that is accelerating horizontally, we can follow these steps:
Step 1: Understanding the Forces Acting on the Pendulum
When the trolley accelerates with a constant acceleration a, the pendulum experiences two forces:
1. The gravitational force acting downward, mg.
2. The tension T in the string, which acts along the string.
In the non-inertial frame of the accelerating trolley, a pseudo force Fpseudo=ma acts on the pendulum in the opposite direction of the trolley's acceleration (to the left if the trolley accelerates to the right).
Step 2: Setting Up the Equilibrium Conditions
At the mean position of the pendulum, the net force in both the x (horizontal) and y (vertical) directions must be zero.
In the vertical direction (y-axis):
Tcosθ−mg=0
This implies:
Tcosθ=mg(1)
In the horizontal direction (x-axis):
Tsinθ−ma=0
This implies:
Tsinθ=ma(2)
Step 3: Dividing the Two Equations
To eliminate T, we can divide equation (2) by equation (1):
TsinθTcosθ=mamg
This simplifies to:
tanθ=ag
Step 4: Finding the Angle θ
To find the angle θ that the pendulum makes with the vertical, we take the inverse tangent:
θ=tan−1(ag)
Conclusion
Thus, the mean position of the pendulum makes an angle θ with the vertical given by:
θ=tan−1(ag)
When the trolley accelerates with a constant acceleration a, the pendulum experiences two forces:
1. The gravitational force acting downward, mg.
2. The tension T in the string, which acts along the string.
At the mean position of the pendulum, the net force in both the x (horizontal) and y (vertical) directions must be zero.
Tcosθ−mg=0
This implies:
Tcosθ=mg(1)
Tsinθ−ma=0
This implies:
Tsinθ=ma(2)
To eliminate T, we can divide equation (2) by equation (1):
TsinθTcosθ=mamg
This simplifies to:
tanθ=ag
To find the angle θ that the pendulum makes with the vertical, we take the inverse tangent:
θ=tan−1(ag)
Thus, the mean position of the pendulum makes an angle θ with the vertical given by:
θ=tan−1(ag)
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section - B) (OBJECTIVE TYPE QUESTIONS)
30 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section C) (PREVIOUS YEARS QUESTIONS)
43 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Exercise
20 Videos
- NUCLEIBOOK - AAKASH INSTITUTECHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
5 Videos
Similar Questions
Knowledge Check
A seconds pendulum is suspended from the ceiling of a trolley moving horizontally with an acceleration of 4m/s2 . Its period of oscillation is
A1.90sB1.70sC2.30sD1.40sA simple pendulum is set up in a trolley which moves to the right with an acceleration a on a horizontal plane. Then the thread of the pendulum in the mean position makes an angle θ with the vertical
Atan−1(ag) in the forward directionBtan−1(ag) in the backward directionCtan−1(ga) in the backward directionDtan−1(ga) in the forward directionAn electron moving with a uniform velocity along the positive x-direction enters a magnetic field directed along the positive y-direction. The force on the electron is directed along
APositive y− directionBNegative y-directionCPositive z- directionDNegative z-direction
A simple pendulum is hanging from the roof of a trolley which is moving horizontally with acceleration g. If length of the string is L and mass of the bob is m, then time period of oscillation is
View SolutionWhat are the direction of acceleration at various point during the oscialltion of a simple pendulum?
View SolutionFor a particle moving along the vertical direction, assume s,v,a and t represent displacement, velocity, acceleration and time respectively. Assume the vertical upward direction as the positive direction.
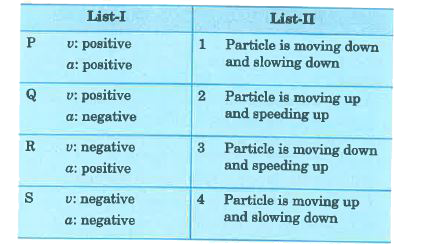
View SolutionAt mean position of an oscillating simple pendulum
View SolutionA simple pendulum is suspended from the roof of a trolley which moves in a horizontal direction with an acceleration α, then the time period is given by T=2π√(IT) where g is equal to
View Solution

