A uniform thin ring of radius R and mass m suspended in a vertical plane from a point in its circumference. Its time period of oscillation is
2π√2Rg
π√2Rg
π2√Rg
π√R2g
The correct Answer is:A
To find the time period of oscillation of a uniform thin ring of radius R and mass m suspended from a point in its circumference, we can follow these steps:
Step 1: Understand the Setup
The ring is suspended from a point on its circumference and can oscillate in a vertical plane. The center of mass of the ring is at its geometric center.
Step 2: Identify the Forces
When the ring is displaced by a small angle θ, the gravitational force mg acts downwards through the center of mass. The component of this force that acts to restore the ring back to its equilibrium position is mgsinθ.
Step 3: Calculate the Torque
The torque τ about the point of suspension due to the weight of the ring is given by:
τ=−mgsinθ⋅R
The negative sign indicates that the torque acts in the opposite direction of the displacement.
Step 4: Use Small Angle Approximation
For small angles, we can approximate sinθ≈θ (in radians). Thus, the torque can be rewritten as:
τ≈−mgθR
Step 5: Relate Torque to Angular Acceleration
According to the rotational dynamics, torque is also related to angular acceleration α by:
τ=Iα
where I is the moment of inertia of the ring about the point of suspension.
Step 6: Calculate the Moment of Inertia
Using the parallel axis theorem, the moment of inertia I of the ring about the point of suspension is:
I=Icm+md2
where Icm=mR2 (moment of inertia about its center) and d=R (distance from the center of mass to the point of suspension). Thus,
I=mR2+mR2=2mR2
Step 7: Substitute into the Torque Equation
Now substituting I into the torque equation:
−mgθR=2mR2α
Since α=d2θdt2, we can rewrite this as:
−mgθR=2mR2d2θdt2
Step 8: Rearrange to Form a Simple Harmonic Motion Equation
Dividing through by mR:
−gθ=2Rd2θdt2
Rearranging gives:
d2θdt2+g2Rθ=0
This is the standard form of simple harmonic motion.
Step 9: Identify the Angular Frequency
From the equation, we can identify the angular frequency ω:
ω2=g2R
Thus,
ω=√g2R
Step 10: Calculate the Time Period
The time period T of oscillation is given by:
T=2πω=2π√2Rg
Final Answer
The time period of oscillation of the ring is:
T=2π√2Rg
---
The ring is suspended from a point on its circumference and can oscillate in a vertical plane. The center of mass of the ring is at its geometric center.
When the ring is displaced by a small angle θ, the gravitational force mg acts downwards through the center of mass. The component of this force that acts to restore the ring back to its equilibrium position is mgsinθ.
The torque τ about the point of suspension due to the weight of the ring is given by:
τ=−mgsinθ⋅R
The negative sign indicates that the torque acts in the opposite direction of the displacement.
For small angles, we can approximate sinθ≈θ (in radians). Thus, the torque can be rewritten as:
τ≈−mgθR
According to the rotational dynamics, torque is also related to angular acceleration α by:
τ=Iα
where I is the moment of inertia of the ring about the point of suspension.
Using the parallel axis theorem, the moment of inertia I of the ring about the point of suspension is:
I=Icm+md2
where Icm=mR2 (moment of inertia about its center) and d=R (distance from the center of mass to the point of suspension). Thus,
I=mR2+mR2=2mR2
Now substituting I into the torque equation:
−mgθR=2mR2α
Since α=d2θdt2, we can rewrite this as:
−mgθR=2mR2d2θdt2
Dividing through by mR:
−gθ=2Rd2θdt2
Rearranging gives:
d2θdt2+g2Rθ=0
This is the standard form of simple harmonic motion.
From the equation, we can identify the angular frequency ω:
ω2=g2R
Thus,
ω=√g2R
The time period T of oscillation is given by:
T=2πω=2π√2Rg
The time period of oscillation of the ring is:
T=2π√2Rg
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section - A) (OBJECTIVE TYPE QUESTIONS)
60 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section - B) (OBJECTIVE TYPE QUESTIONS)
30 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - EXAMPLE
21 Videos
- NUCLEIBOOK - AAKASH INSTITUTECHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
5 Videos
Similar Questions
Knowledge Check
A uniform thin ring of radius R and mass m suspended in a vertical plane from a point in its circumference. Its time period of oscillation is
A2π√rgB2pi sqrt((5 R)/(3 g))C2π√7R5gD2π√2R5gA uniform disc of radius R is pivoted at point O on its circumstances. The time period of small oscillations about an axis passing through O and perpendicular to plane of disc will be
A2π(Rg)B2π√2R3gC2π√2RgD2π√3R2g)A disc of radius R is suspended from its circumference and made to oscillate. Its time period will be:
A2π√3R2gB2π√4RgC2π√RgD2π√2Rg
A ring of the radius r is suspended from a point on its circumference. If the ring is made to oscillate in the plane of the figure, then the angular frequency of these small oscillations is
View SolutionA uniform disc of mass m and radius r is suspended through a wire attached to its centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionA circular ring of weight 4kg and radius 60 cm is suspended on a horizontal nail at its circumference. The frequency of oscillation for small oscillations was found to be____Hz. (Take √8.17=2.85)
View SolutionA uniform ring of radius 'R' is suspended from a horizontal nail 'A' as shown. Find time period of its small oscillations.
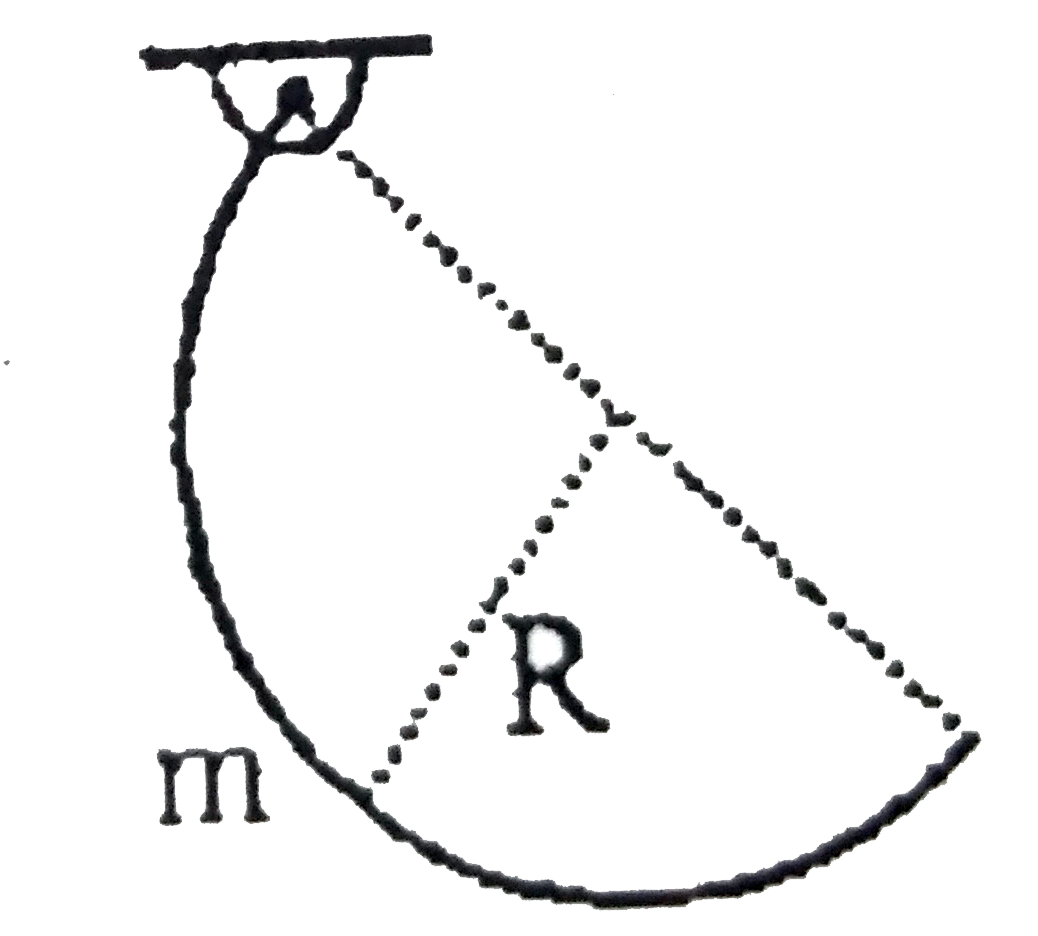
View SolutionA uniform semi circular ring of radius R and mass m is free to oscillate about its one end in its vertical plane as shown in the figure. Find time period of SHM of its centre of mass of the ring (take π2=10)
View Solution

