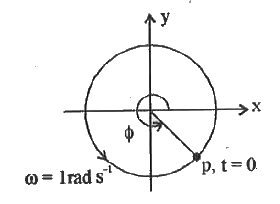
Plot the corresponding SHM of particle. Indicate the intial (t=0) position of the particle, the radius of the circle and angular speed of the rotating particle. Consider sense of rotation to be anticlockwise and x in cm and t is in s.
(b) x=cos(π6−t)Solution in Kannada
x=cos(π6−t)=1.cos[−(t−π6)]=1.cos(t−π6).
orx=cos(1.t−π6)=cos(t+11π6).
Comparing this with x=Acos(ωt+ϕ) we get,
A=1cm,ω=1 rad s1,ϕ=−π6 rad 2π−π6=11π6
Topper's Solved these Questions
- OSCILLATIONSBOOK - SUBHASH PUBLICATIONCHAPTER - OSCILLATIONSEXERCISE - FIVE MARKS QUESTIONS WITH ANSWERS
4 Videos
- MOTION IN PLANEBOOK - SUBHASH PUBLICATIONCHAPTER - MOTION IN PLANEEXERCISE - NUMERICALS WITH SOLUTIONS
47 Videos
- PHYSICAL WORLDBOOK - SUBHASH PUBLICATIONCHAPTER - PHYSICAL WORLDEXERCISE - TWO MARKS QUESTIONS WITH ANSWERS
30 Videos
Similar Questions
Arrive an expression for time period of simple pendulum.
View SolutionTwo forces 8N and 5N are acting at a point on a body at an angle 120∘ then find the (i) magnitude and (ii) direction of their resultant.
View SolutionMathematics is the language of physics. Justify the statement.
View SolutionPlot the corresponding reference circle for each of the following simple hoarmonic motions. Indicate the intial (t=0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case : (x is in cm and t is in s ). (a) x=-2 sin(3t+pi//3) (b) x=cos(pi//6-t) (c) x=3sin(2pit+pi//4) (d) x=2cospit
View SolutionPlot the corresponding reference circle for given SHM x=2cos(π3−t) .Indicate the initial (t−0) position of the particle , the radius of the circle and the angular speed of the rotating particle . Consider anticlockwise direction for rotation.
Hint : x=2cos(π3−t)=2cos(t−π3) as cos(−θ)=cosθ . Compare with x=Acos(ωt+ϕ)
View SolutionPlot the corresponding SHM of particle. Indicate the intial (t=0) position of the particle, the radius of the circle and angular speed of the rotating particle. Consider sense of rotation to be anticlockwise and x in cm and t is in s. (a) =-2sin(3t(pi)/(3))
View SolutionPlot the corresponding SHM of particle. Indicate the intial (t=0) position of the particle, the radius of the circle and angular speed of the rotating particle. Consider sense of rotation to be anticlockwise and x in cm and t is in s.
(c ) x=3sin(2πt+π4)
View SolutionPlot the corresponding SHM of particle. Indicate the intial (t=0) position of the particle, the radius of the circle and angular speed of the rotating particle. Consider sense of rotation to be anticlockwise and x in cm and t is in s. (d) x=2 cos pit
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).
View SolutionPlot the corresponding reference circle for given SHM x = 2 cos ((pi)/(3) -t) .Indicate the initial ( t- 0) position of the particle , the radius of the circle and the angular speed of the rotating particle . Consider anticlockwise direction for rotation. Hint : x = 2 cos ((pi)/(3) -t)=2 cos ( t- (pi)/(3)) as cos ( - theta) = cos theta . Compare with x= A cos ( omegat + phi)
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t = 0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case : (x is in cm and t is in s). a) x=-2 sin (3t + pi//3) b) x= cos (pi//6 -t) c) x=3 sin (2pi t+ pi//4) d) x=2 cos pit .
View SolutionPlot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s). x =-2 sin (3t+(π/3))
View Solution
