Plot the corresponding reference circle for given SHM .Indicate the initial position of the particle , the radius of the circle and the angular speed of the rotating particle . Consider anticlockwise direction for rotation.
Hint as . Compare with
as
Compare with
The reference circle is as shown in figure

Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION -A)
58 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION-B )
25 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
13 Videos
- NUCLEIBOOK - AAKASH INSTITUTECHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
5 Videos
Similar Questions
Plot the corresponding reference circle for given SHM, indicate the initial (t=0) position of the paritcle, the radius of the circle , and the angular speed of the rotating paritcle. Consider anticlockwise direction for rotation. x=−3sin(2t+π4) (Express in the form , x= Acos ( omega t + phi)).
View SolutionPlot the corresponding reference circle for each of the following simple hoarmonic motions. Indicate the intial(t=0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case :(x is in cm and t is in s ).
(a) x=−2sin(3t+π/3) (b)x=cos(π/6−t) (c) x=3sin(2πt+π/4) (d) x=2cosπt
View Solution
Knowledge Check
cos(3π2+θ)cos(2π+θ)×[cot(3π2−θ)+cot(2π+θ)]=?
A0B1CsinθDcosθ
Prove that: 4 cosθcos (π3+θ)cos(π3−θ)=cos3θ
View Solution2cos(π2−θ)+3sin(π2+θ)−(3sinθ+2cosθ)=
View SolutionProve that cosx+cos(2π3−x)+cos(2π3+x)=0
View SolutionThe corresponding reference circle for a given SHM has been shown in the figure. Given π=7π4 . Write the corresponding equation of SHM. Consider anticlockwise direction for rotation.
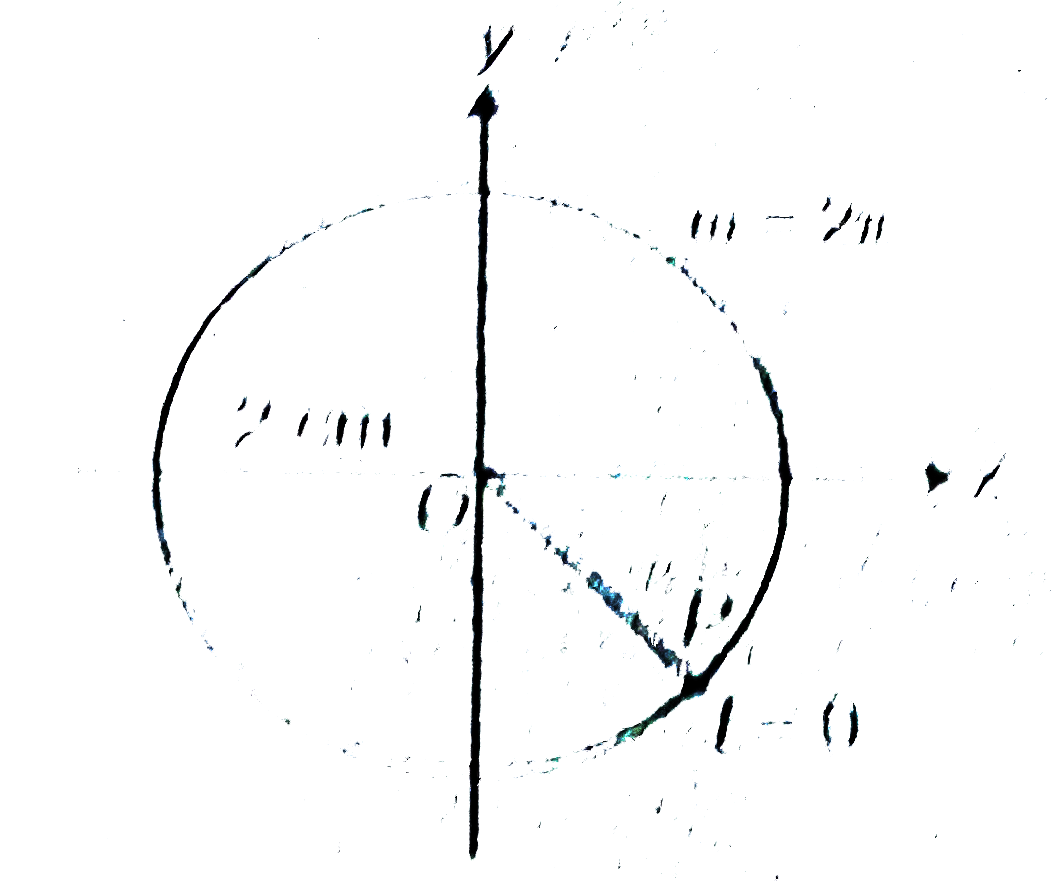
Hint :x=2cos(2πt+7π4)
2cos(3π2+(2πt+π4))
View Solutioncos(3π2+θ)⋅cos(2π+θ)=
View Solutionprove: 12(cost+√3sint)=cos(π3−t)
View Solutionprove: 12(cost+√3sint)=cos(π3−t)
View Solution
