A mass is suspended separately by two springs of spring constants k1 and k2 in successive order. The time periods of oscillations in the two cases are T1 and T2 respectively. If the same mass be suspended by connecting the two springs in parallel, (as shown in figure) then the time period of oscillations is T. The correct relations is

T2=T21+T22
T−2=T−21+T−22
T−1=T−11+T−12
T=T1+T2
The correct Answer is:B
(b) T=2π√mk or T∝1√k or k=αT2
Now , ke=k1+k2
∴αT2=αT21+αT22
or T−2=T−21+T−22
Similar Questions
A block of mass m is suspended by different springs of force constant shown in figure. Let time period of oscillation in these four positions be T1,T2,T3andT4 Then -
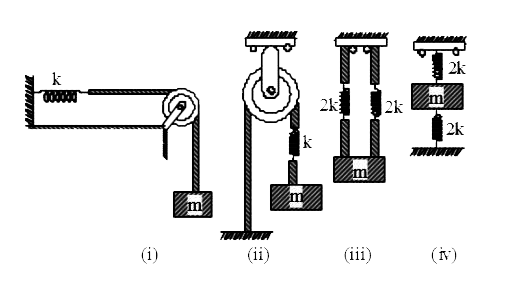 View Solution
View SolutionA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A mass m is suspended separately by two different springs of spring constant k1 and k2 given the time period t1 and t2 respectively. If the same mass m is shown in the figure then time period t is given by the relation
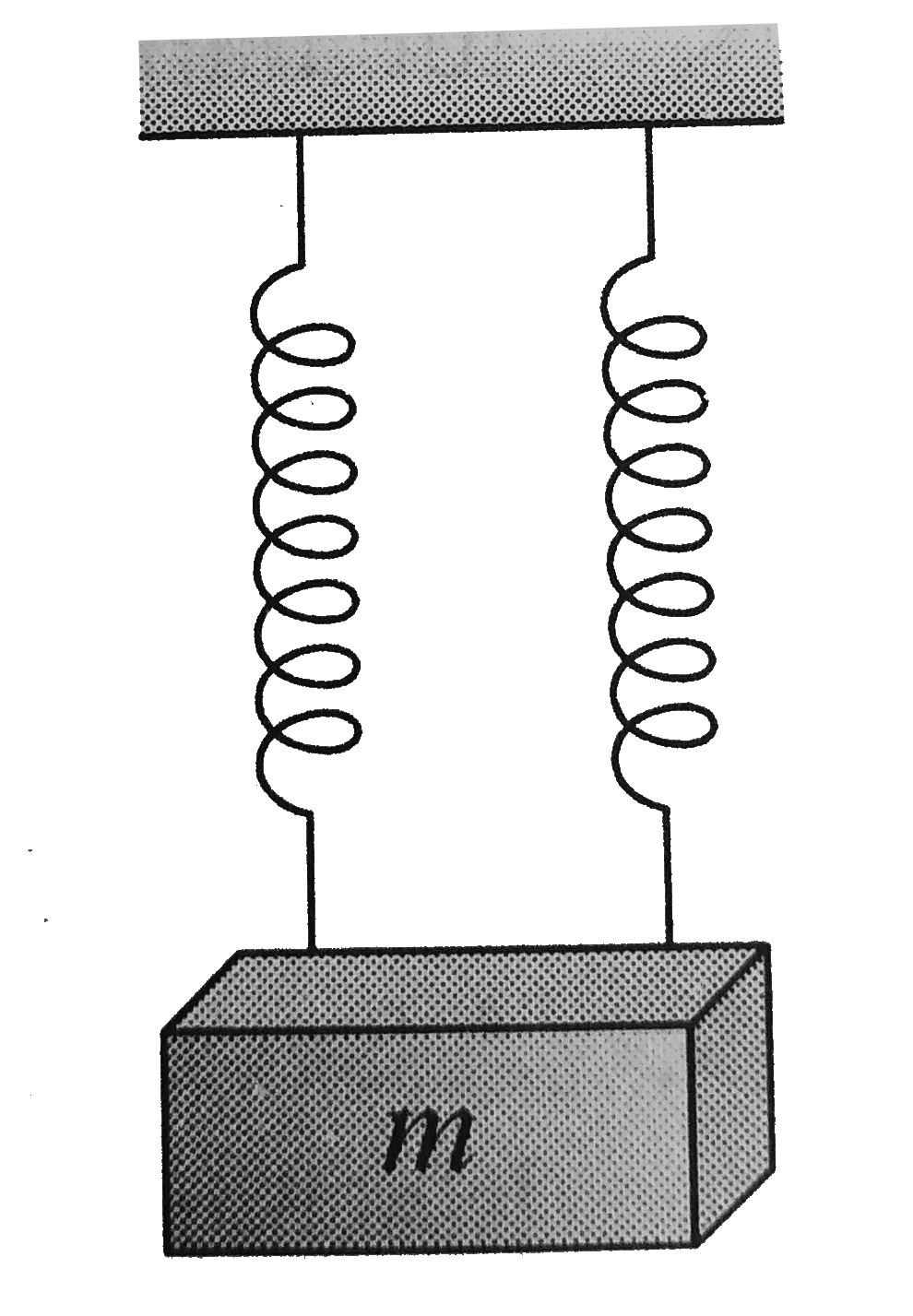 At=t1+t2Bt=t1t2t1+t2Ct2=t21+t22Dt−2=t−21+t−22
At=t1+t2Bt=t1t2t1+t2Ct2=t21+t22Dt−2=t−21+t−22 - Question 2 - Select One or More
When a body is suspended from two light springs separately, the periods of vertical oscillations are T1 and T2. When the same body is suspended from the two spring connected in series, the period will be
ATp<T1<T2<TsBT2s=T21+T22C√Ts=√T1+√T2D1T2p=1T21+1T22 - Question 3 - Select One
A mass M is suspended by two springs of force constants K1andK2 respectively as shown in the diagram. The total elongation (stretch) of the two springs is
 AmgK1+K2Bmg(K1+K2)K1K2CmgK1K2K1+K2DK1+K2K1K2mg
AmgK1+K2Bmg(K1+K2)K1K2CmgK1K2K1+K2DK1+K2K1K2mg
T1,T2 are time periods of oscillation of a block when individually suspended to springs of force constants K1,K2 respectively. If same block is suspended to series combination of same two springs, then it time period of oscillation.
View SolutionA block of mass m is suspended by different springs of force constant shown in figure Let time period of oscillation in these four positions be T1,T2,T3 and T4. Then :
 View Solution
View SolutionTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
 View Solution
View SolutionTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
 View Solution
View SolutionA mass is suspended separately by two different springs in successive order then time periods is t1 and t2 respectively. If it is connected by both spring as shown in figure then time period is to, the correct relation is
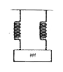 View Solution
View Solution

