A block of mass 10 kg is suspended by three strings as shown in figure . Tension T2 is

A
100N
B
100√3N
C
√3×100N
D
50√3N
Video Solution
Text Solution
The correct Answer is:D
(d) T3sin60∘=T2sin30∘
∴T3=T2√3
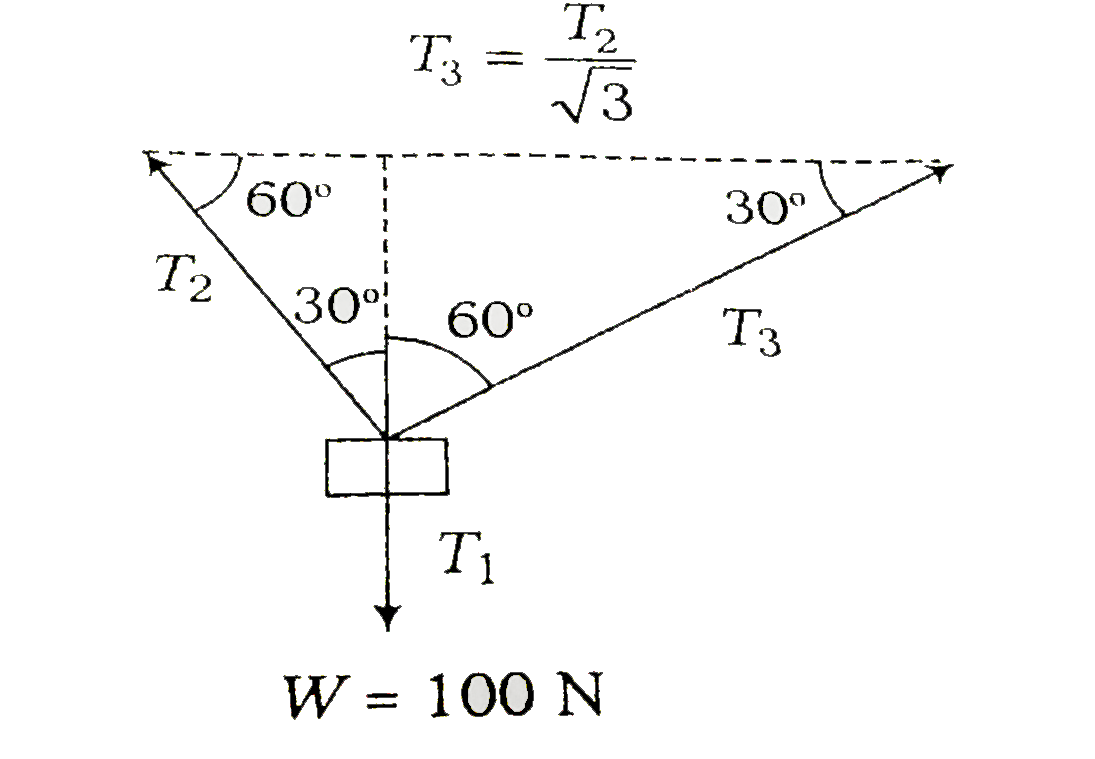
W=100 N
Now, T2cos30∘+T3cos60∘=T1=100
or √3T22+T2√3×12=100 or 8T24√3=100 or T2=50√3N
|
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.3
20 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.1
20 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Knowledge Check
A body of mass 60 kg suspended by means of three strings, P, Q and R as shown in the figure is in equlibrium. The tension in the string P is
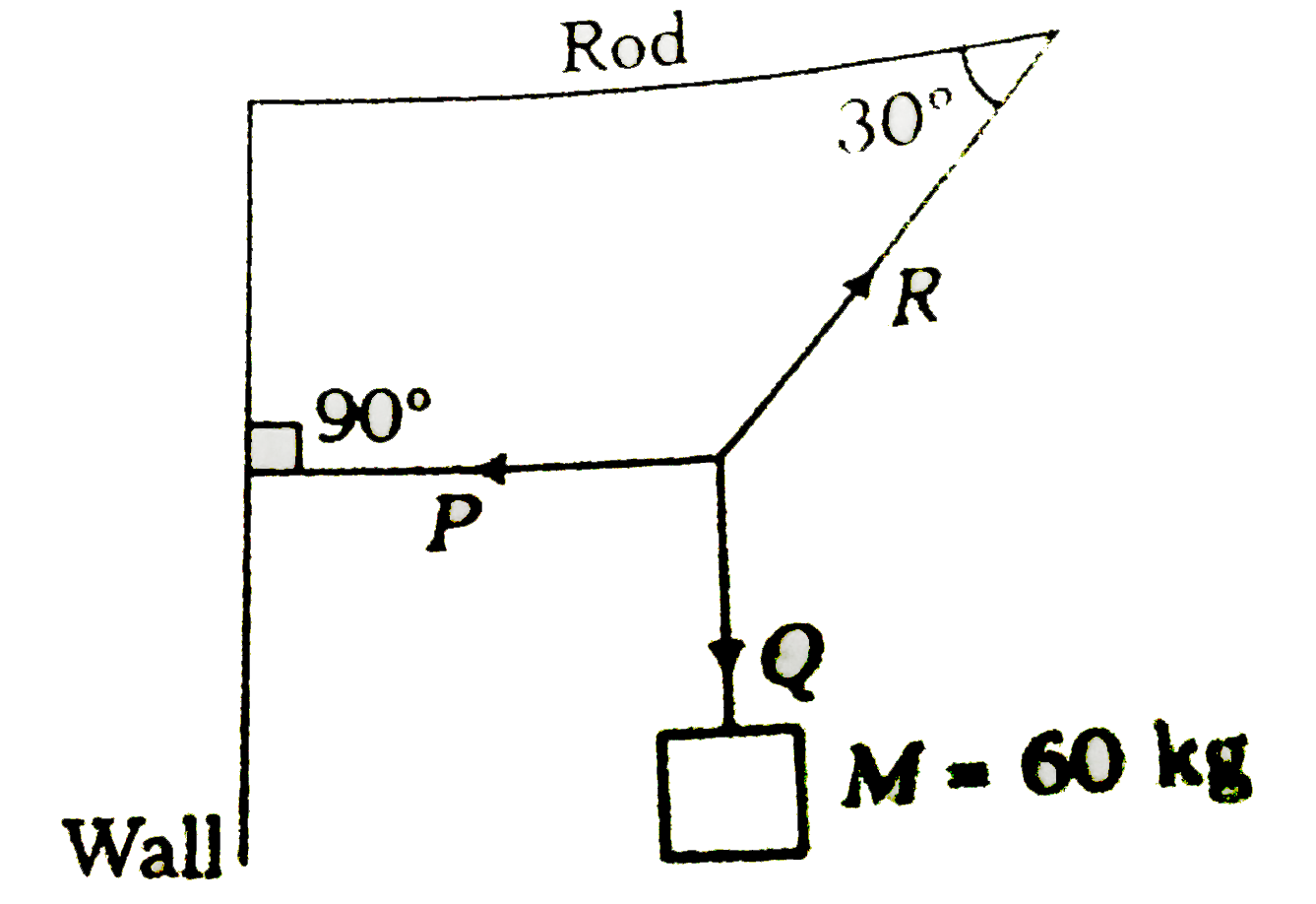 A130.9 kgfB60 kgfC50 kgfD103.9 kgf
A130.9 kgfB60 kgfC50 kgfD103.9 kgfA block of weight W is supported by three strings as shown in figure. Which of the following relations is true for tension in the string ? (Here, T1,T2 and T3 are the tension in the strings A,B and C respectively)
 AT1=T2BT1=T3CT2=T3DT1=T2=T3
AT1=T2BT1=T3CT2=T3DT1=T2=T3A body of weight 2 kg is suspended as shown in the figure. The tension T1 in the horizontal string (in kg wt ) is
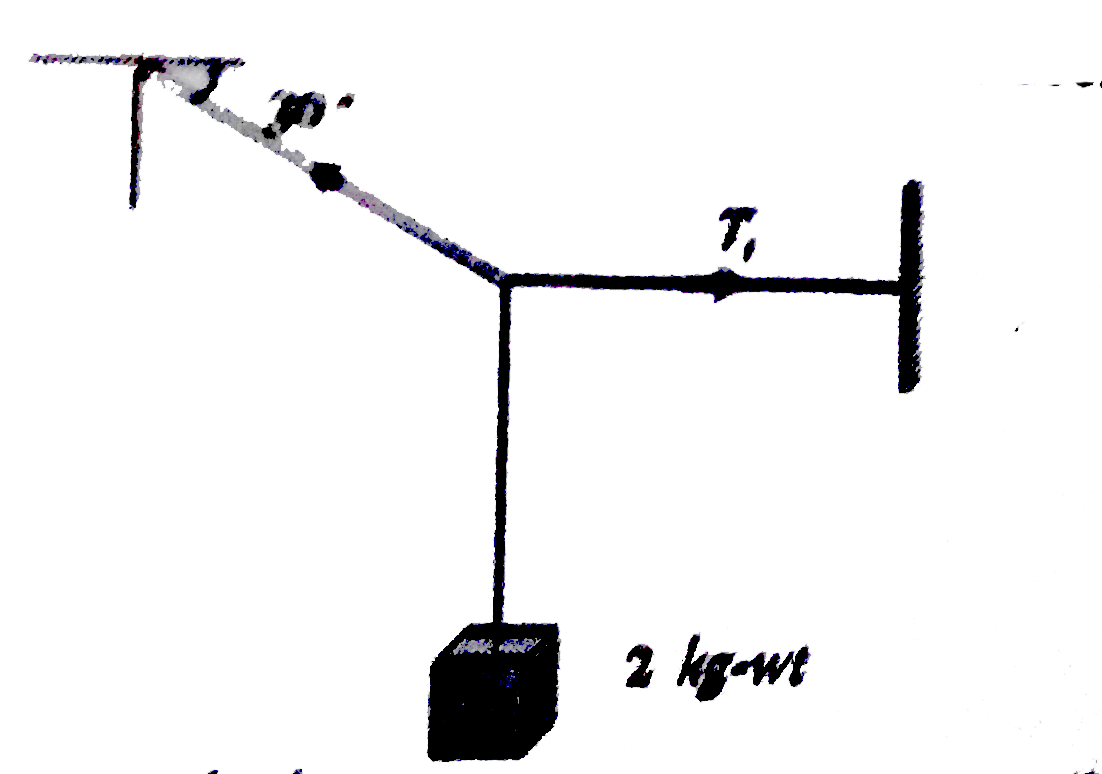 A2/√3B√3/2C2√3D2
A2/√3B√3/2C2√3D2
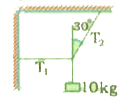
A block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions T1andT2 in the two string.
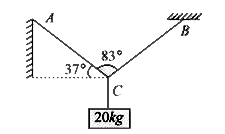
View SolutionA block of mass 20kg is balanced by three strings A, B & C as shown in figure. Ratio of tensions in strings AC and BC(TA/TB) is
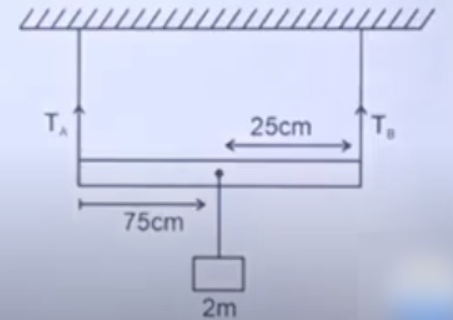
View SolutionA block of mass 10 kg is suspended throug two light spring balances as shown in figure

View SolutionA block of mass 6 kg is kept in equilibrium with the help of strings as shown in figure. The tension in string PQ is (g = 10 m/s2)
View SolutionA block of mass 3m is suspended by a meter scale rod of mass m as shown in figure. If the tension in the string A is equal to kmg in equilibrium, then value of k will be
View Solution