The verical of point above the ground is twice that of Q. A particle is projected downward with a speed of 5ms−1 from P and at the same time another particle is projected upward with the same speed from W. Both particle reach the ground simultaneously, then
PQ = 30 m
time of flight of stones = 3 s
Both (a) and (b) are correct
Both (a) and (b) are wrong
The correct Answer is:C
−h=5t−5t2 and −2h=−5t−5t2
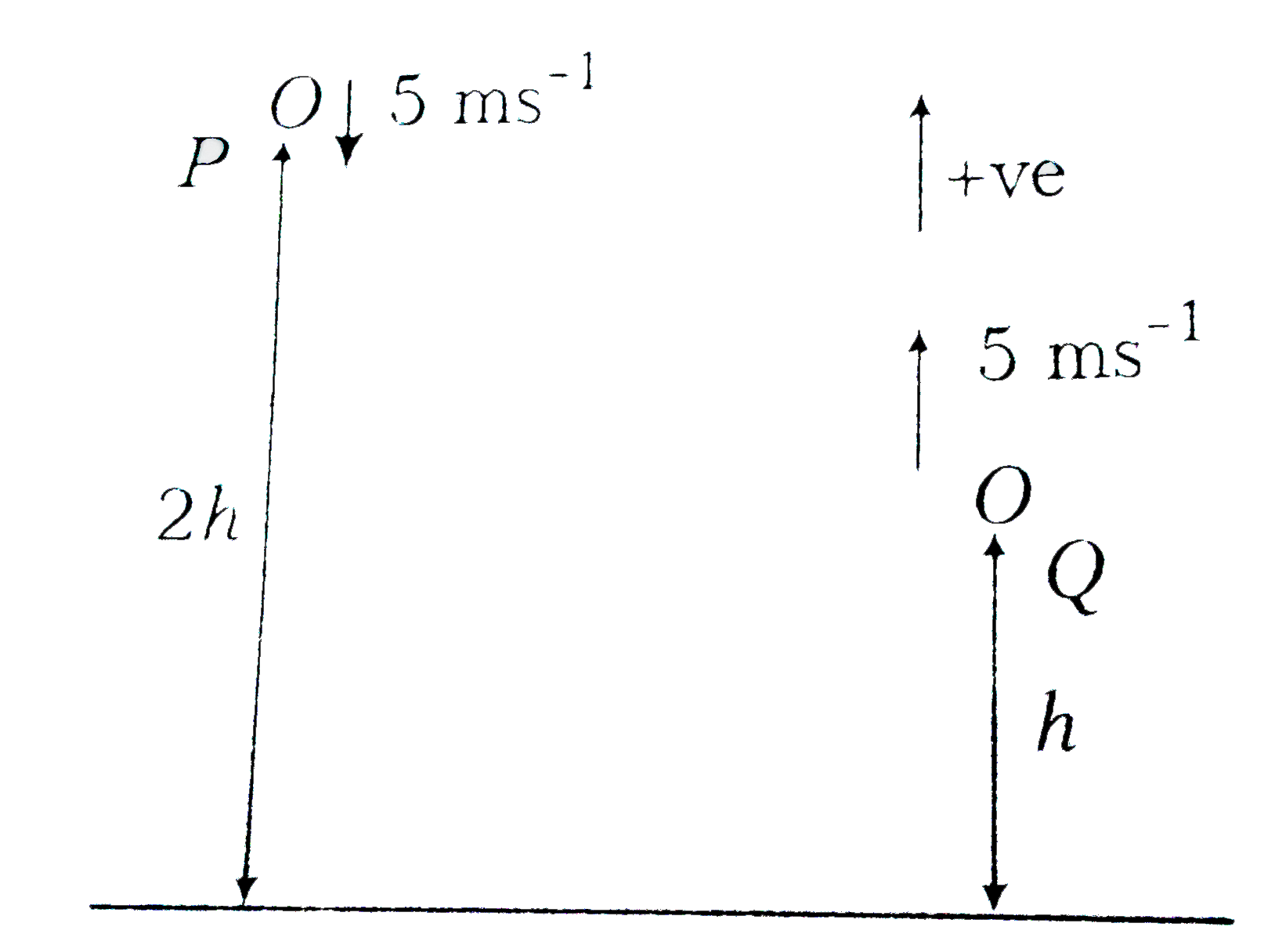
From these two equations we get, t = 3 s and h = distance between P and Q = 30 m.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Assertion and reason)
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Mathch the columns)
6 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.7
15 Videos
- MOTIONBOOK - DC PANDEYCHAPTER - MOTIONEXERCISE - Medical entrances gallery
19 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
Similar Questions
Height of a tower is 125m and a particle is dropped from rest from the top of the tower. After two seconds of its fall, another particle is projected downwards with a speed u such that both the particles reach the ground simultaneously. What is the value of u?
View SolutionThere is one tower of height h. one particle A is projected upward from top of the tower with a speed u1 at time t=0 . At time t=t0 another particle B is projected upward from the bottom of the tower with a speed u2. When will the particles cross each other?
View Solution
Knowledge Check
A particle is projected horizontally will speed 20ms−1 from the top of a tower. After what time velocity of particle will be at 45∘ angle from initial direction of projection.
A1sB2sC3sD4sTwo particles A and B are projected simultaneously from a fixed point of the ground. Particle A is projected on a smooth horizontal surface with speed v, while particle B is projected in air with speed v, while particle B is projected in air with speed 2v√3. If particle B hits the particle A, the angle of projection of B with the vertical is
A30∘B60∘CBoth (a) and (b)D45∘A particle is projected at an angle θ from ground with speed u (g = 10 m/s2 )
Aif u = 10 m/s and θ=30∘, then time of flight will be 1 secBIf u=10√3m/sandθ=60∘, then time of flight will be 3 sec.Cif u=10√3m/sandθ=60∘, then after 2 sec velocity becomes perpendicular to initial velocity.Dif u = 10 m/s and θ=30∘, then velocity never becomes perpendicular to initial velocity during its flight.
A particle is dropped from a height 12 g metre and 4 s after another particle is projected from the ground towards it with a velocity 4gms−1 The time after which the second particle meets first is
View SolutionA particle is dropped from a height 12 g metre and 4 s after another particle is projected from the ground towards it with a velocity 4gms−1 The time after which the second particle meets first is
View SolutionA particle is projected at angle θ with horizontal from ground. The slop (m) of the trajectory of the particle varies with time (t) as
View SolutionAssertion Particle-1 is dropped from a tower and particle-2 is projected horizontal from the same tower. Then both the particles reach the ground simultaneously.
Reason Both are particles strike the ground with different speeds.
View SolutionA particle is projected from horizontal ground with speed 5ms−1 at 53∘ with horizontal. Find time after which velocity of particle will be 45∘ with horizontal:
View Solution