The position of object moving along an x-axis is given by , where x is in meters and t in seconds. Find the position of the object at the following values of t : (i) 2s, (ii) 4s, (iii) What is the object's displacement between t = 0 s and t = 4 s ? and (iv) What is its average vvelocity for the time interval from t = 2 s to t = 4 ?
To solve the problem step by step, we will follow the instructions given in the question.
Given:
The position of the object is given by the equation:
x(t)=3t−4t2+t3
where x is in meters and t is in seconds.
Step 1: Find the position of the object at t=2 seconds.
Substituting t=2 into the position equation:
x(2)=3(2)−4(22)+(23)
Calculating each term:
=6−4(4)+8
=6−16+8
=−2 meters
Step 2: Find the position of the object at t=4 seconds.
Substituting t=4 into the position equation:
x(4)=3(4)−4(42)+(43)
Calculating each term:
=12−4(16)+64
=12−64+64
=12 meters
Step 3: Calculate the displacement between t=0 seconds and t=4 seconds.
First, find the position at t=0:
x(0)=3(0)−4(02)+(03)=0 meters
Now, the displacement Δx is given by:
Δx=x(4)−x(0)=12−0=12 meters
Step 4: Calculate the average velocity for the time interval from t=2 seconds to t=4 seconds.
The average velocity vavg is given by:
vavg=ΔxΔt
where Δx=x(4)−x(2) and Δt=4−2.
We already found x(4)=12 meters. Now we need x(2)=−2 meters (from Step 1).
Calculating Δx:
Δx=x(4)−x(2)=12−(−2)=12+2=14 meters
Now, substituting into the average velocity formula:
vavg=142=7 meters/second
Summary of Results:
1. Position at t=2 seconds: −2 meters
2. Position at t=4 seconds: 12 meters
3. Displacement from t=0 to t=4 seconds: 12 meters
4. Average velocity from t=2 to t=4 seconds: 7 meters/second
The position of the object is given by the equation:
x(t)=3t−4t2+t3
where x is in meters and t is in seconds.
x(2)=3(2)−4(22)+(23)
Calculating each term:
=6−4(4)+8
=6−16+8
=−2 meters
x(4)=3(4)−4(42)+(43)
Calculating each term:
=12−4(16)+64
=12−64+64
=12 meters
x(0)=3(0)−4(02)+(03)=0 meters
Δx=x(4)−x(0)=12−0=12 meters
vavg=ΔxΔt
where Δx=x(4)−x(2) and Δt=4−2.
Δx=x(4)−x(2)=12−(−2)=12+2=14 meters
vavg=142=7 meters/second
1. Position at t=2 seconds: −2 meters
2. Position at t=4 seconds: 12 meters
3. Displacement from t=0 to t=4 seconds: 12 meters
4. Average velocity from t=2 to t=4 seconds: 7 meters/second
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.1
10 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.2
10 Videos
- MOTIONBOOK - DC PANDEYCHAPTER - MOTIONEXERCISE - Medical entrances gallery
19 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
Similar Questions
The position of the particle moving along x-axis is given by x=2t−3t2+t3 where x is in mt and t is in second.The velocity of the particle at t=2sec is
View SolutionThe position of an object moving along x-axis is given by a+bt2, where a=8.5 m and b=2.5m/s2 and t is measured in seconds. The average velocity (in m/s) of the object between t=2s and t=4s is
View Solution
Knowledge Check
The position of a particle moving on the x-axis is given by x=t3+4t2−2t+5 where x is in meter and t is in seconds
(i) the velocity of the particle at t=4s is 78m/s
(ii) the acceleration of the particle at t=4s is 32m/s2
(iii) the average velocity during the interval t=0 to t=4sis 30m/s
(iv) the average acceleration during the interval t=0 to t=4s is 20m/s2A(i), (ii)B(ii), (iii)C(i), (ii), (iii)DAllThe position of a body moving along x-axis at time t is given by x=(t2−4t+6)m. The distance travelled by body in time interval t = 0 to t = 3 s is
A5 mB7 mC4 mD3 m
In the given x - t graph. Find the position of an object at t = 3 second
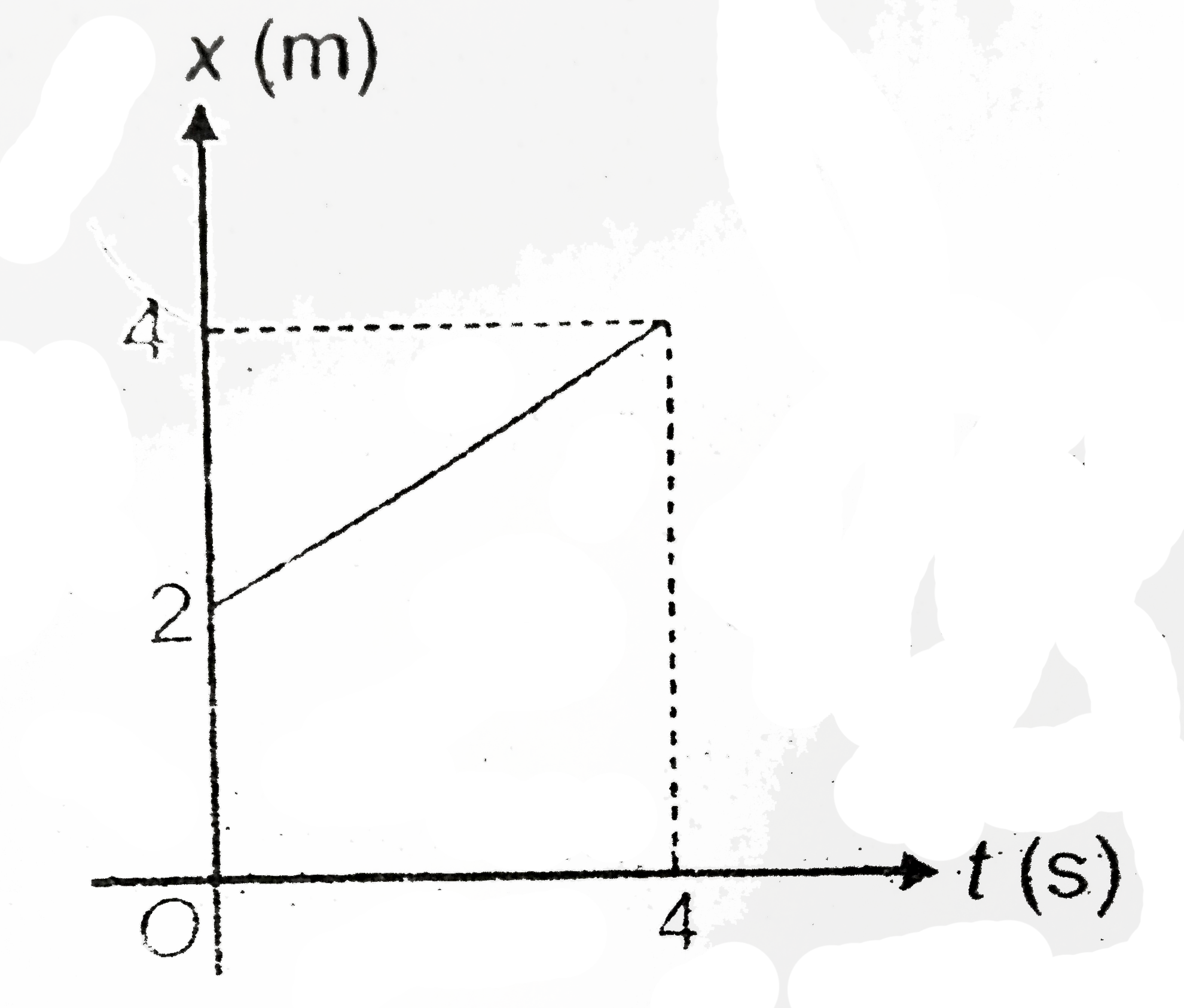
View SolutionThe position (in meters) of a particle moving on the x-axis is given by: x=2+9t+3t2−t3, where t is time in seconds . The distance travelled by the particle between t= 1s and t= 4s is m.
View SolutionThe position of a particle moving along an x axis is given by x=12t2−2t3, where x is in meters and t is in seconds. Determine (a) the position, (b) the velocity, and (c) the acceleration of the particle at t=3.5s. (d) What is the maximum positive coordinate reached by the particle and (e) at what time is it reached ? (f) What is the maximum positive velocity reached by the particle and (g) at what time is it reached ? (h) What is the acceleration of the particle at the instant the particle is not moving ( other than at t=0 ) ? (i) Determine the average velocity of the particle between t=0 and t=3s .
View SolutionA particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionThe displacement of a particle moving along an x axis is given by x=18t+5.0t2, where x is in meters and t is in seconds. Calculate (a) the instantaneous velocity at t=2.0s and (b) the average velocity between t=2.0s and t=3.0s.
View SolutionThe position of an object moving on a straight line is defined by the relation x=t3−2t2−4t, where x is expressed in meter and t in second. Determine
(a) the average velocity during the interval of 1 second to 4 second.
(b) the velocity at t = 1 s and t = 4 s,
(c) the average acceleration during the interval of 1 second to 4 second.
(d) the acceleration at t = 4 s and
View Solution
