Three equal masses 3 kg are connected by massless string of cross sectional area 0.005cm−2 and Young's modulus 2×1011N/m−2. In the absence of friction the longitudes strain in the wire
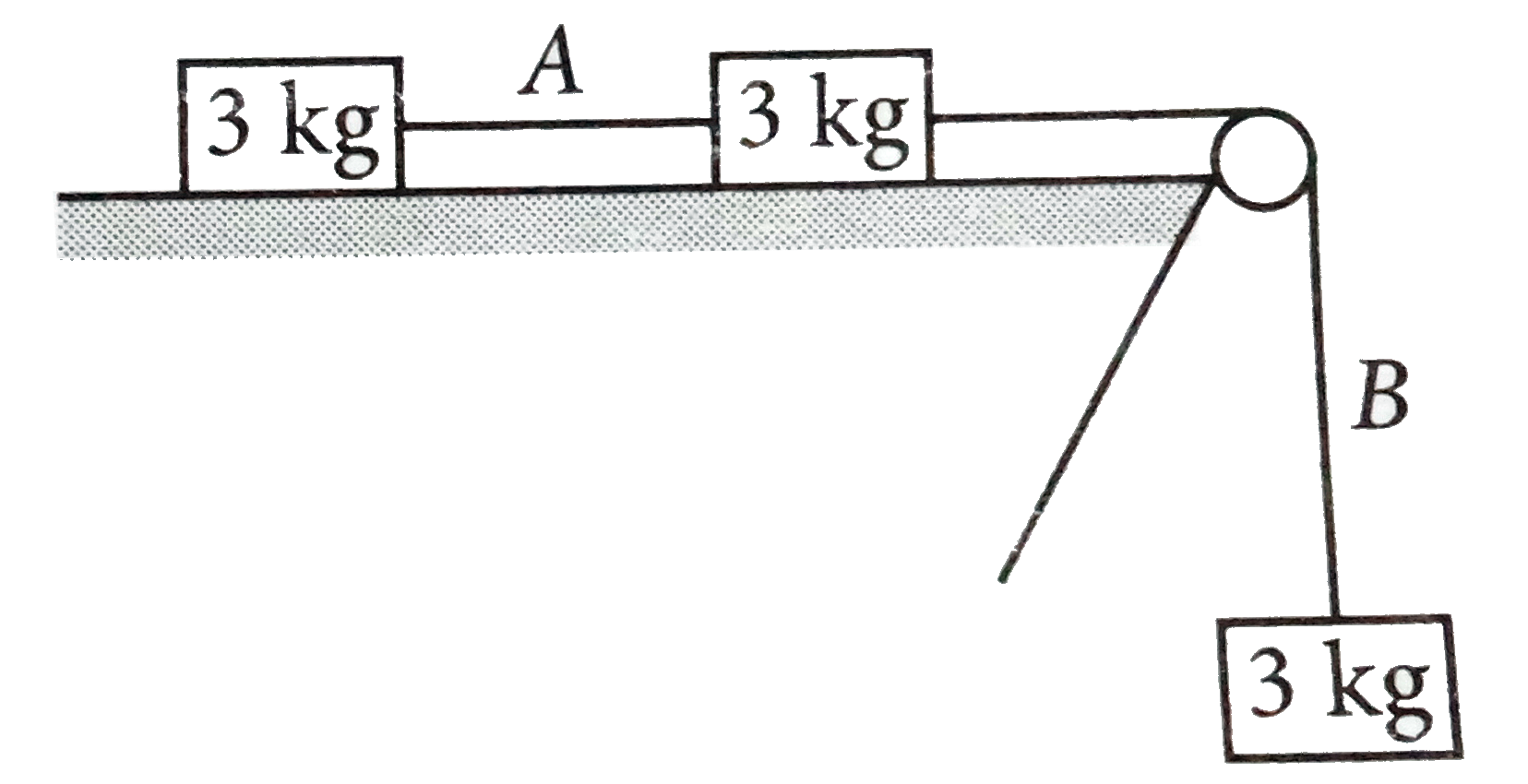
A is 10−4
B is 2×10−4
Both (a) and (b)
None the these
The correct Answer is:C
3g−TB=3a
TA=3a
TB−TA=3a are the equations defining the motion
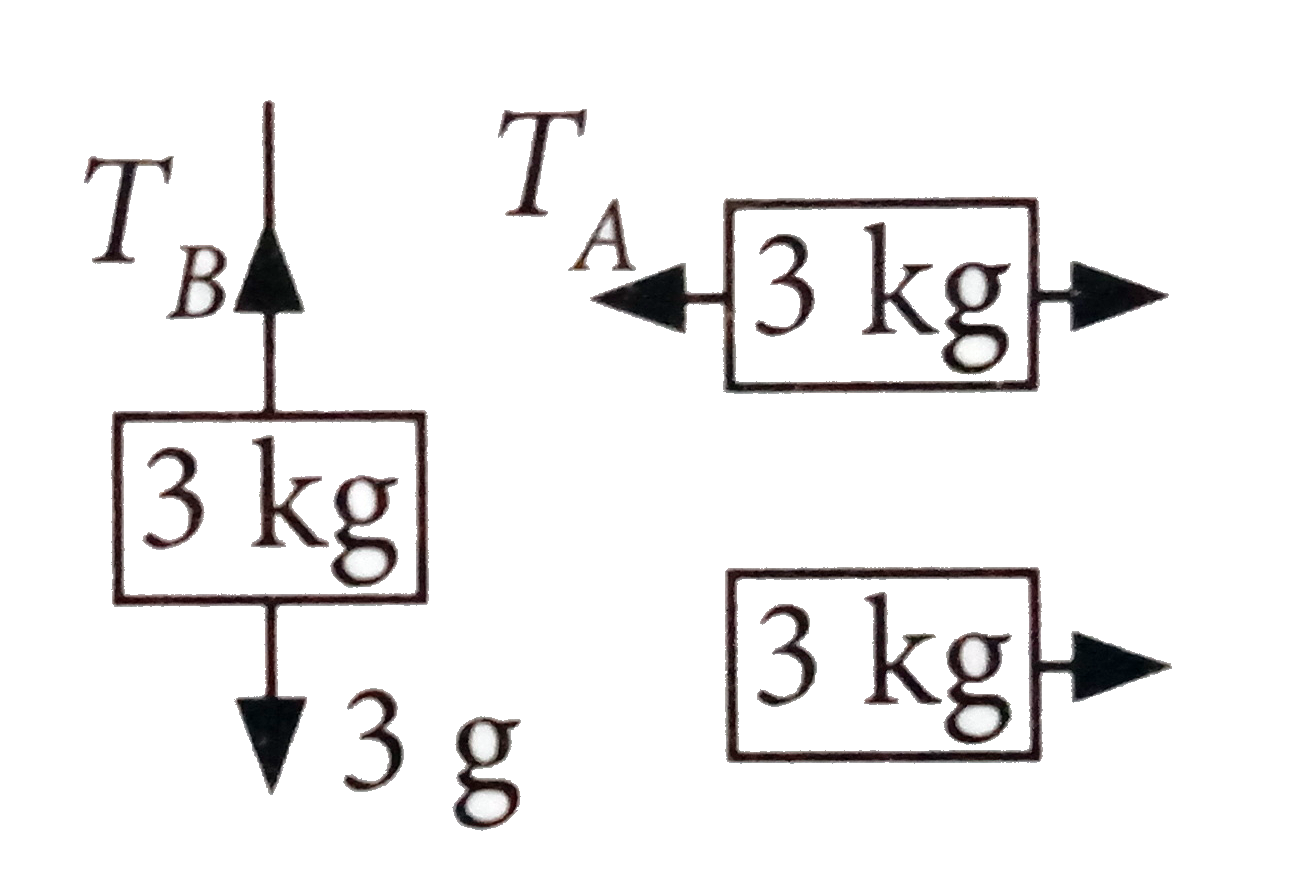
Sloving we get, a=3g9=g3,TA=gandTB=2g
So, Strain in A=TAaY=g0.005×10−4×Y=10−4
Strain in B=TBay=2g0.005×Y×10−4=2×10−4
So, choice (a) and (b) are correct and so choice (c) is correct answer.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTIPSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:NCERT Exemplar
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTIPSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:Assertion And Reason
Explore 15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTIPSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:Applications Of Elastic Behaviour Of Materials
Explore 8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NCERT FINGERTIPSChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:Assertion And Reason
Explore 15 Videos
- MOTION IN A PLANE Book:NCERT FINGERTIPSChapter:MOTION IN A PLANE Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
Each of the three blocks P, Q and R shown in figure has a mass of 3 kg. Easch of the wires A and B has cross sectional area 0.005 cm^2 and Young modulus 2x1011Nm−2. Neglect friction. Find the longitudinal strain developed ineach of the wires. Take g=10ms−2

View SolutionA system consisting of light wires and blocks is released from rest as shown in figure. The coefficient of kinetic friction between the blocks and surfaces is 0.1 and masses of blocks m1,m2 & m3 are 4 kg,4kg and 2 kg respectively. The cross section of the wire between m2 and m3 is 0.003 cm2 and its Young's Modulus is 2×1011N/m2. Calculate
the acceleration of the block m2.
(b) the longitudinal strain developed in the wire m2 and m3.
View Solution
Knowledge Check
Each of three blocks shows in figure has a mass 3 kg. The wire connecting blocks A and B has area of cross-section 0.005cm2 and Young's modulus of elasticity Y=2×1011N/m2 . Neglect friction. Find the elasticity potential energy stored per unit volume in wire connecting blocks A and B in steady state.
(Takeng=10m/s2)
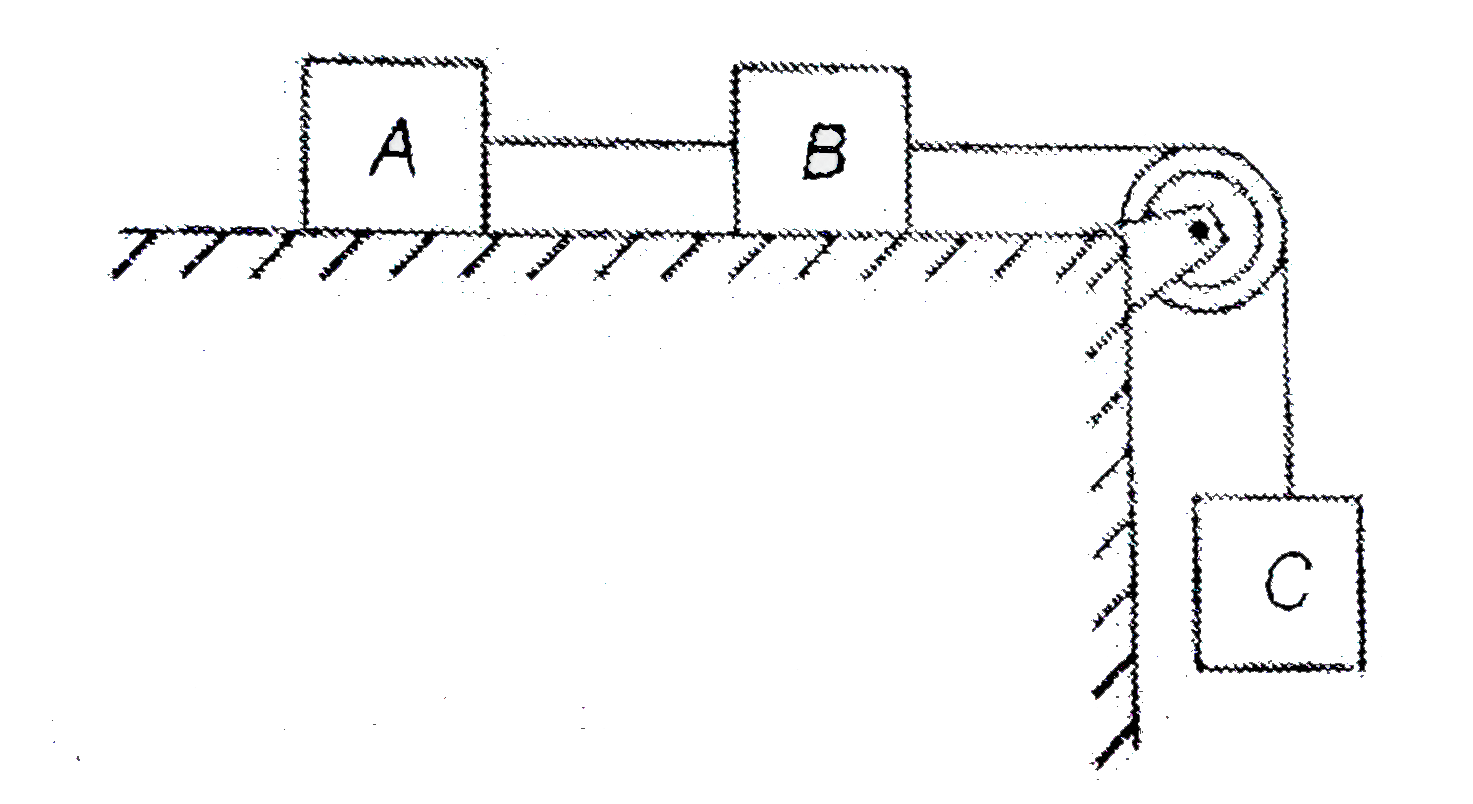 A500J/m3B1000J/m3C2000J/m3D3000J/m3
A500J/m3B1000J/m3C2000J/m3D3000J/m3The area of a cross-section of steel wire is 0.5cm−2 and Young's modulus of steel is 2×1011Nm−2. The force required to stretch by 0.5% of its length is
A1000 NB2000 NC4000 ND5000NThe area of a cross section of steel wire is 0.1cm2 and Young's modulus of steel is 2×1011Nm−2. The force required to strech by 0.1% of its length is
A1000NB2000NC4000ND5000N
A steel wire4.0m in length is stretched through 2.0mm.The cross -sectional area of the wire is 2.0mm2.If young's modulus of steel is 2.0×1011N/m2
(a) the enrgy density of wire,
(b) the elastic potential energy stored in the wire.
View SolutionSpeed of a transverse wave on a straight wire ( mass 6.0 g, length 60 cm and area of cross - section 1.0 mm2 ) is 90ms−1. If the Young's modulus of wire is 16×1011Nm−2 , the extension of wire over its natural length is :
View SolutionA steel wire of mass μ per unit length with a circular cross-section has a radius of 0.1cm. The wire is of length 10m when measured lying horizontal, and hangs from a hook on the wall. A mass fo 25kg is hung from the free end of the wire. Assume the wire to be uniform and laterla strain << logitudinal strain. If density of steel is 7860kgm−3 and Young's modulus is 2×1011N/m2 then the extension in the length fo the wire is
View SolutionA steekl rod of Young's modulus 2×1011N/m2 undergoes an elastic strain of 0.05% .The energy per unit voulme stored in J/m3 is
View SolutionThe length of a rod is 20cm and area of cross-section 2cm2. The Young's modulus of the material of wire is 1.4×1011N/m2. If the rod is compressed by 5 kg-wt along its length, then increase in the energy of the rod in joules will be
View Solution