A rectangle with its sides parallel to the x-axis and y-axis is inscibed in the region bounded by the curves y=x2−4 and 2y=4−x2. The maximum possible area of such a rectangle is closest to the integer
A
10
B
9
C
8
D
7
Video Solution
Text Solution
The correct Answer is:B
y=x2−4&2y=4−x2
P(α,β)⇒2β=4−α2
P(α,4−α22)
Q(−α,4−α22)
PQ=2α
S(α,α2−4),R(−α,−4+α2)
PS=(α2−4−4−α22)=3α2−122
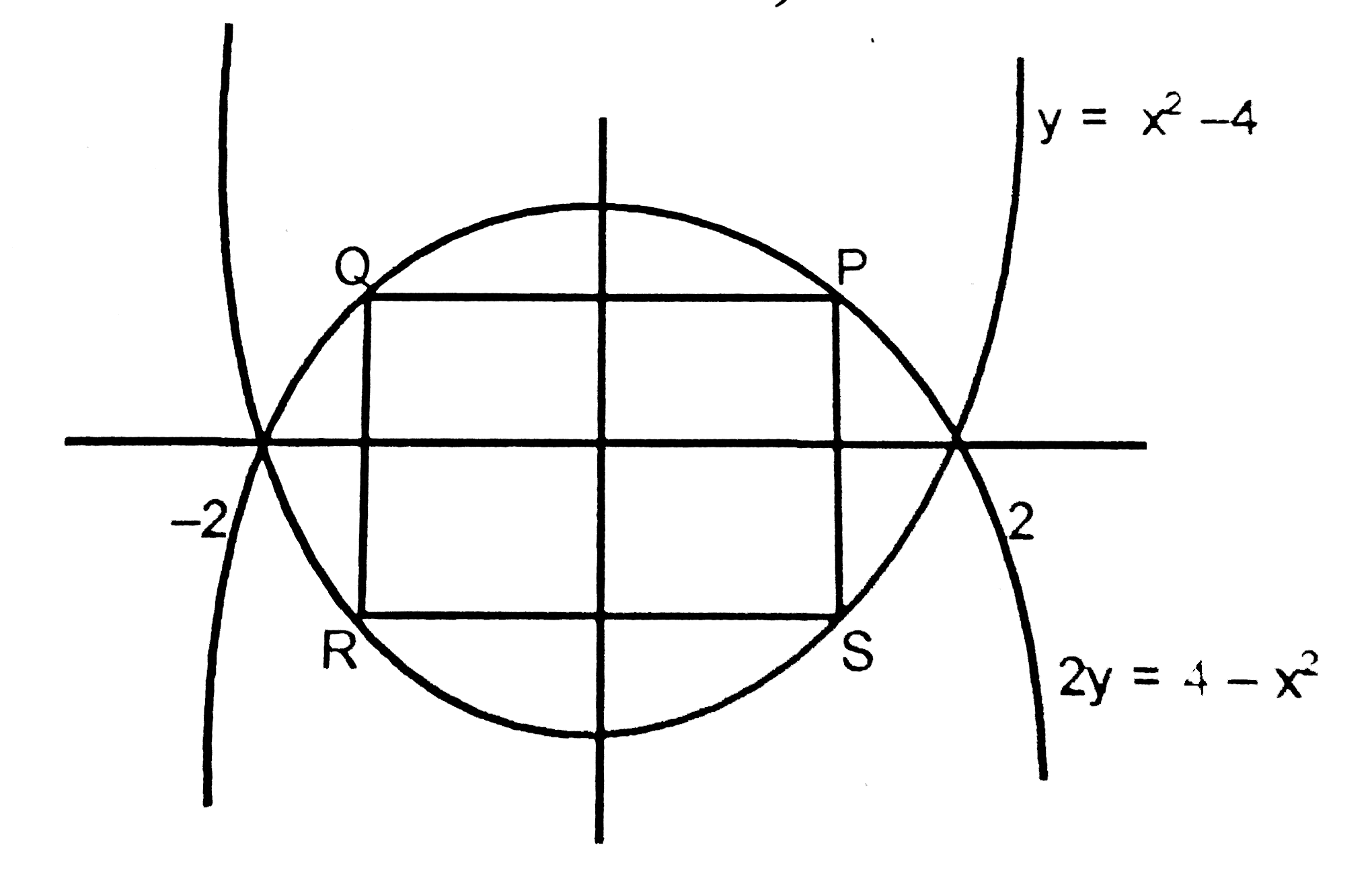
Area=PQPS=2α.3α2−122=3α3−12α=A(α)
A'(α)=9α2−12=0⇒α2129=43
α=±2√3 maximum at α=−2√3
Maix. =A(−2Sqrt(3))=−3.83√3+12.2√3=16√3=16√33=9.22≈9
|
Topper's Solved these Questions
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - PART-2 MATHMATICS
10 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part 2 Mathematics
15 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part B- Mathematics
10 Videos
- KVPY 2021BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART II MATHEMATICS
4 Videos
Similar Questions
Knowledge Check
The area of the region bounded by the curves y=x2 and x=y2 is
A13B12C14D3Area of the region bounded by the curve y=x2−5x+4 and the X-axis is
A32B52C72D92The area of the region bounded by the curves x=y2−2 and x=y is
A94B9C92D97
Find the area of the region bounded by the curve y2=4x and x2=4y.
View SolutionThe area of the region bounded by the curve y=2x−x2and X- axis is
View SolutionThe area bounded by the curve x=2−y−y2 and Y-axis is
View SolutionThe area of the region bounded by the curve y=2x−x2 and x - axis is
View SolutionArea bounded by the curve y=x2+x−6 and the X-axis is
View Solution