A metal box with a square base and vertical sides is to contain 1024 cm3 of water, the material for the top and bottom costs Rs 5 per cm2 and the material for the sides costs Rs 2.50 per cm2. Find the least cost of the box.

Video Solution

Text Solution

Verified by Experts

Since, volume of the box =1024 $c m^{3}$
Let length of the side of square base be x cm and height of the box be y cm.

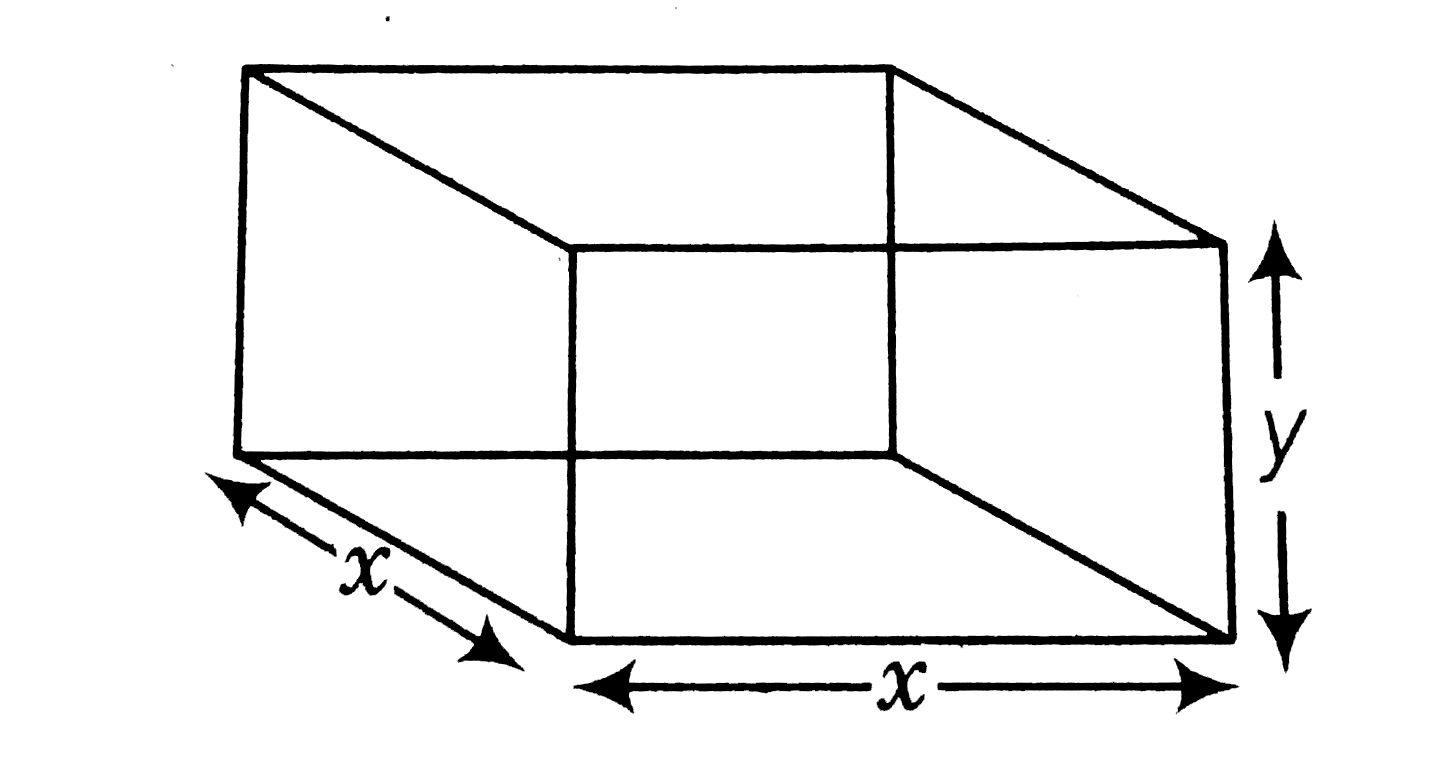
$∴$ Volume of the box(V) $= x^{2} . y = 1024$
Since, $x^{2} y = 1024 \Rightarrow y = \frac{1024}{x^{2}}$
Let C denotes the cost of the box.
$C = 2 x^{2} \times 5 + 4 x y \times 2.50$
$= 10 x^{2} (x + \frac{1024}{x^{2}})$
$= (10 x) (x^{2}) (x^{3} + 1024)$
$\Rightarrow C = 10 x^{2} + \frac{10240}{x}$ ..............(i)
On differentiating both sides w.r.t. x, we get
$\frac{d C}{d x} = 20 x + 10240 {(- x)}^{- 2}$ ................(ii)
Now , $\frac{d C}{d x} = 0$
$\Rightarrow 20 x = \frac{10240}{x^{2}}$
$\Rightarrow 20 x^{3} = 10240$
$\Rightarrow x^{3} = 512 = 8^{3} \Rightarrow x = 8$
Again, differentiating Eq. (ii), w.r.t. x, we get
$\frac{d^{2} C}{{d x}^{2}} = 20 - 10240 (- 2) \frac{.1}{x^{3}}$
$= 20 + \frac{20480}{x^{3}} > 0$
$∴ \frac{d^{2} C}{{({d x}^{2})}_{x = 8}^{2}} = 20 + \frac{20480}{512} = 60 > 0$
For x=8, cost is minimum and the corresponding least cost of the box,
$C (8) = {10.8}^{2} + \frac{10240}{8}$
$= 640 + 1280 = 1920$
$∴$ Least cost =Rs 1920

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLARCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Application Of Integrals
68 Videos

Similar Questions

NCERT EXEMPLAR-APPLICATION OF DERIVATIVES-Application Of Derivatives

Home
Ask Doubt
Profile