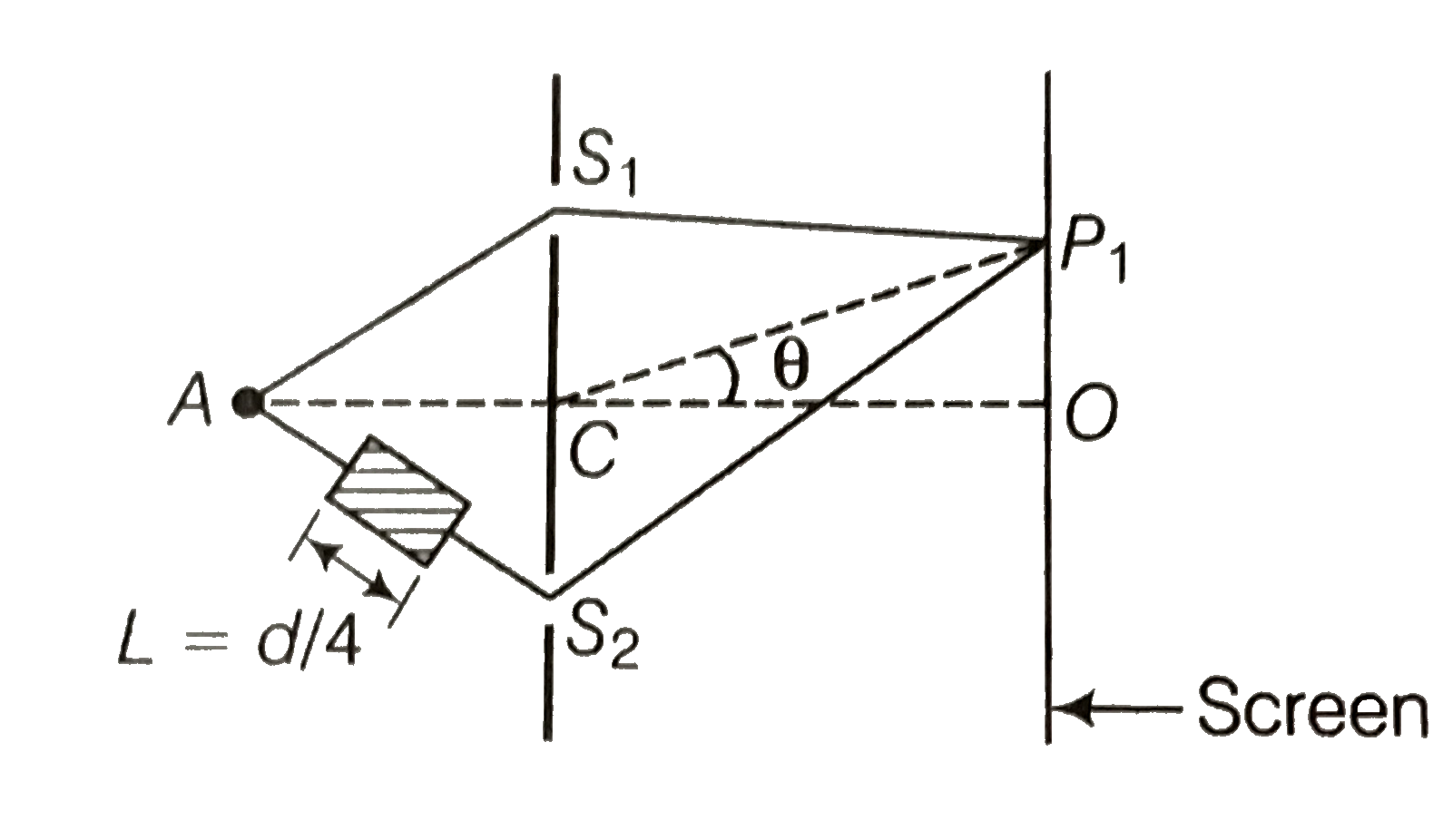
A small transparent slab containing material of is placed along (figure). What will be the distance from O of the principle maxima and of the first minima on either side of the principal maxima obtained in the absence of the glass slab ?
In case of transparent glass slabe of refractive indesx , the path difference will be calculated as
In case of transparent glass slab of refractive index ,
the path difference
For the principal maxima, (path difference is zero )
i.e.,
or,
or
For the first minima, the path difference is
or
[ The diffraction occurs if the wavelength of waves in nearly equal to the side width (d)]
On the positive side
On the negative side
The First principal maxima on the positive side is at distance
above point O
The first principal minima on the negative side is at distance
below point O.
Topper's Solved these Questions
Similar Questions
Light of wavelength 6×10−5 cm falls on screen at a distance of 100 cm from a narrow slit. Find the width of the slit if the first minima lies 1mm on either side of the central maximum
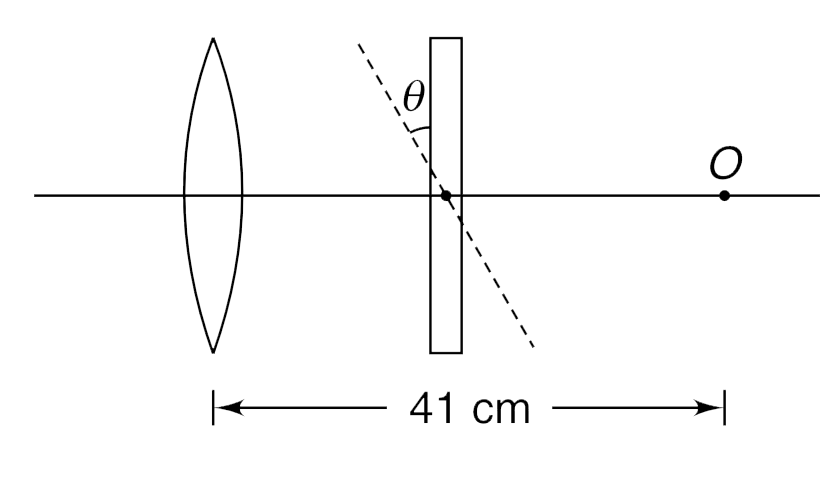
View SolutionA point object O is placed at a distance of 41 cm from a convex lens of focal length f=20 cm on its principal axis. A glass slab of thickness 3 cm and refractive index μ=1.5 is placed between the lens and the object with its faces perpendicular to the principal axis of the lens. Image of the object is formed at point I1. Now the glass slab is tilted by an angle of θ=1° (as shown in the Figure) and the final image is formed at I2. Calculate the distance between points I1 and I2. Consider only paraxial rays for the lens and near normal incidence for the glass slab.
View Solution
Knowledge Check
A small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
The distance of princpal maxima front 'O' isAD4BD16CD8DDA small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
The distance of first minima below the point O isAD√231B3D√231C2D√231D5D√231A small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
The distance of first minima above O isA3D√43BD√247C3D√247D5D√247
Light of wavelengthλ=500nm falls on two narrow slits placed a distance d = 50×10−4 cm
apart, at an angle ϕ=30∘ relative to the slits as shown in figure. On the lower slit a transparent slab of thickness 0.1 mm and refractive index 32 is placed. The interference pattern is observed at a distance D=2m from the slits. Then, calculate
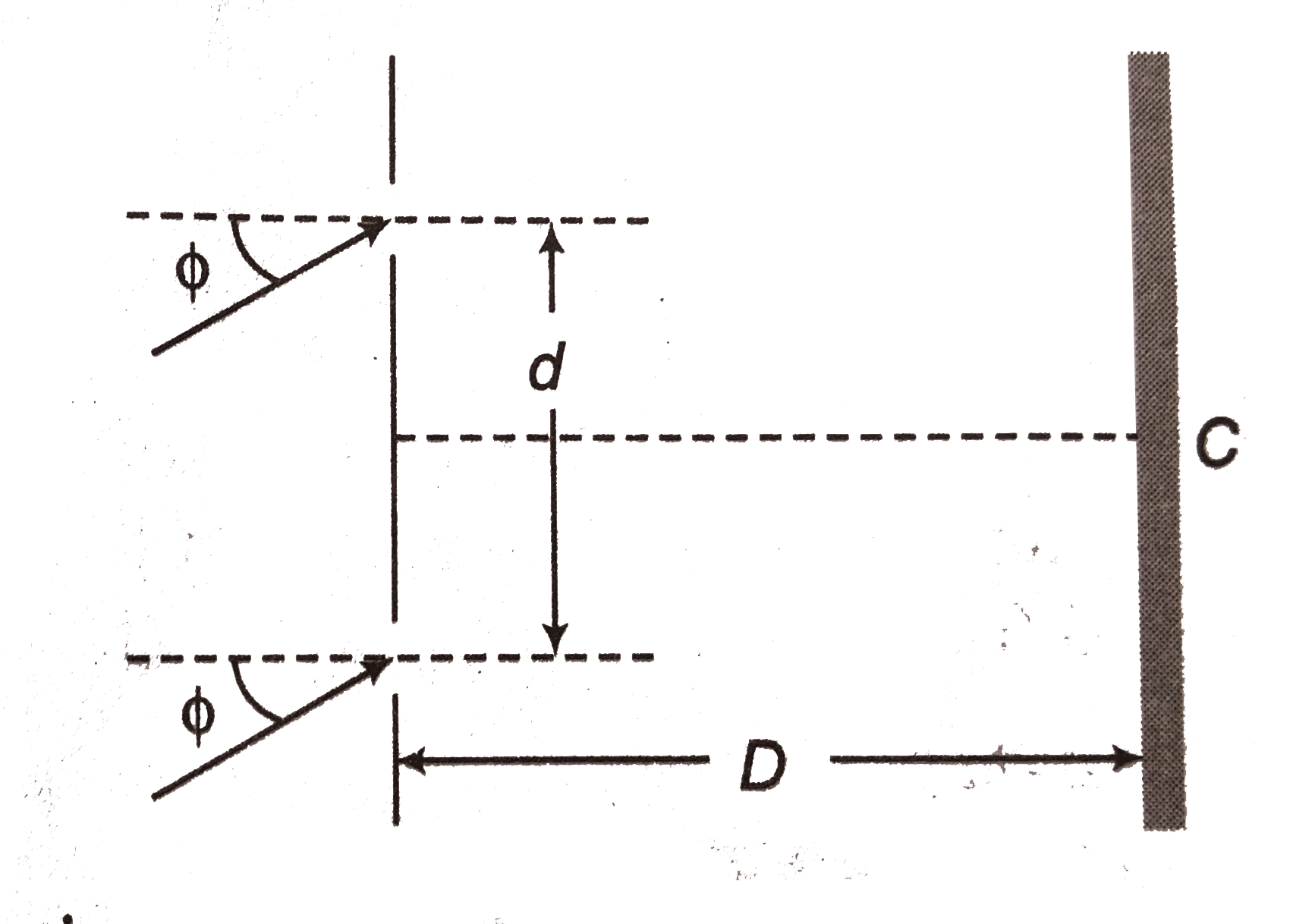
(a) position of the central maxima.
(b) the order of maxima at point C of screen .
(c)how many fringes will pass C, if we remove the transparent slab from the lower slit?
View SolutionA Franhoffer diffraction pattern due to a single slit of width 0.2 mm is being obtained on a screen placed at a distance of 2 m from the slit. The first minima lie at 5mm in either side of the centre maximum on the screen. Find the wavelength of the light used .
View SolutionIn the diffraction from a single slit of width 2.5λ, the total number of minimas and secondary maximum (maxima) on either side of the central maximum are
View SolutionIn the diffraction from a single slit of width 2.5λ, the total number of minimas and secondary maximum (maxima) on either side of the central maximum are
View SolutionIn YDSE arrangement as shown in figure, fringes are seen on screen using monochromatic source S having wavelength 3000 Å (in air). S1and S2 are two slits seperated by d = 1 mm and D = 1m. Left of slits S1 and S2 medium of refractive index n1=2 is present and to the right of S1 and S2 medium of n2=32, is present. A thin slab of thickness 't' is placed in front of S1 . The refractive index of n3 of the slab varies with distance from it's starting face as shown in figure.

In order to get central maxima at the centre of screen, the thickness of slab required is :
View Solution
