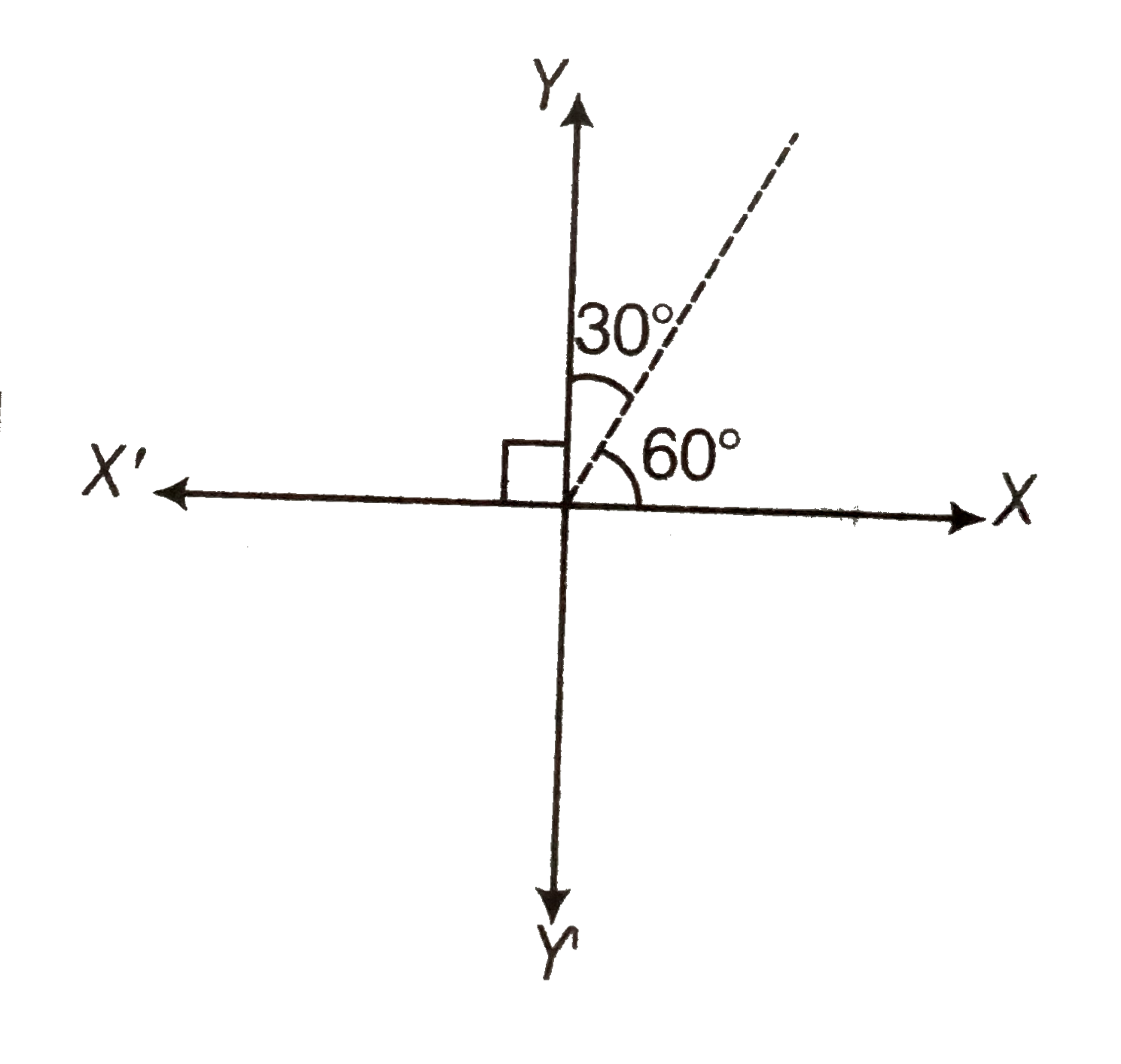
Find the equation of a line passing through $(1, 2)$ and making angle of $30^{0}$ with $y - a ξ s$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:N/a

Given that, angle with Y-axis $= 30^{\circ}$
and angle with X-axis $= 60^{\circ}$
$∴$ Slope of the line, $m = {tan 60}^{\circ} = \sqrt{3}$
So,the equation of a line passing through (1,2)and having slope $\sqrt{3}$ , is
$y - 2 = \sqrt{3} (x - 1)$
$\Rightarrow y - 2 = \sqrt{3} x - \sqrt{3}$
$\Rightarrow y - \sqrt{3} x - 2 + \sqrt{3} = 0$

Updated on:21/07/2023

|

Class 11 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The equations of the lines through $(1.0)$ and making angle of $45^{\circ}$ with $x - 2 y$ =3 are
A $x + 3 y = 3, 3 x - y = 1$
B $x + 3 y = 1, 3 x - y = 3$
C $x - 3 y = 1, x + 3 y = 1$
D $3 x + y = 3, 3 x - y = 3$

NCERT EXEMPLAR-STRAIGHT LINES-MATCHING THE COLUMN

Home
Ask Doubt
Profile