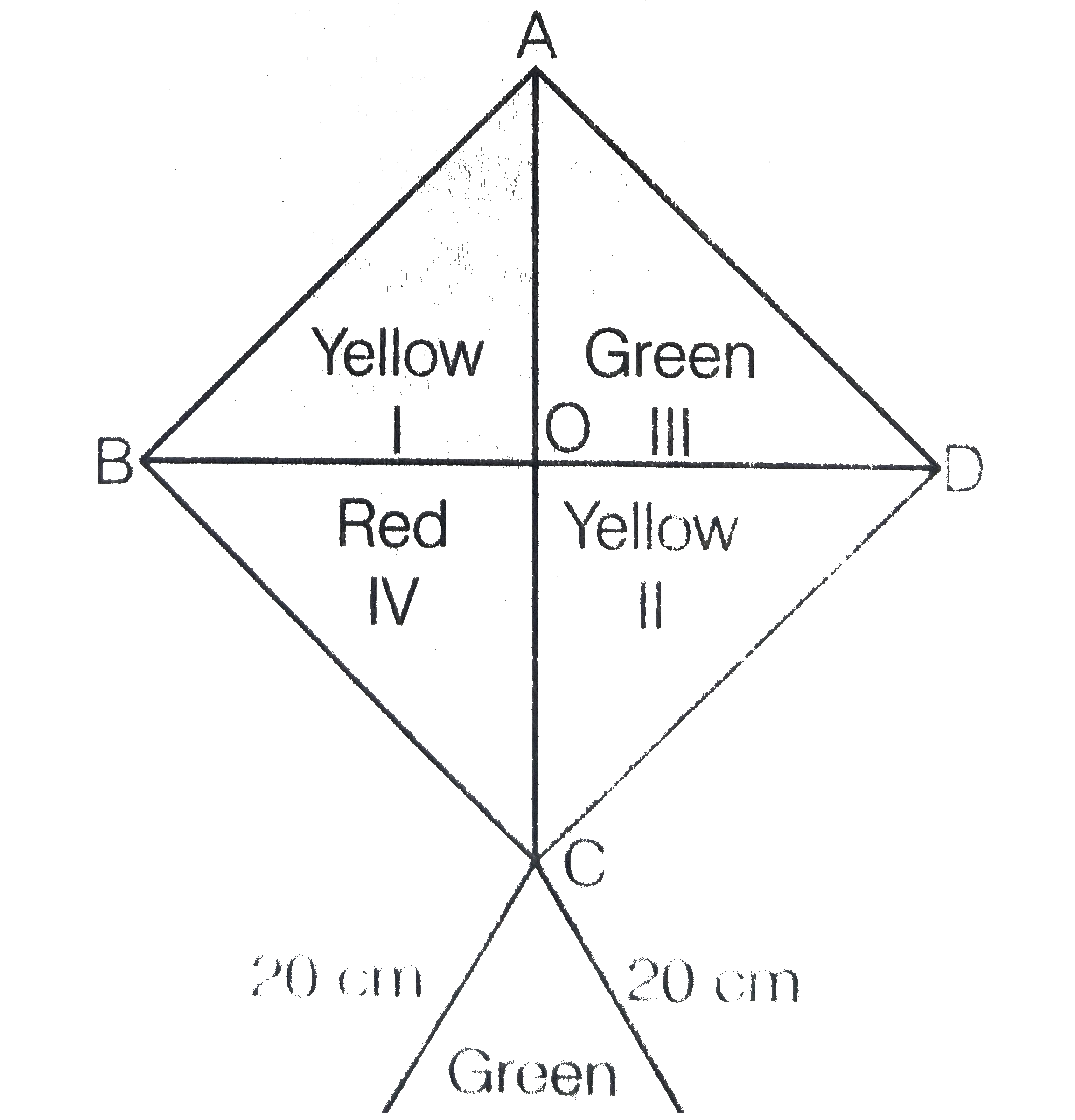
How much paper of each shade is needed to make a kite given in figure, in which ABCD is a square with diagonal 44cm.
Video Solution
Text Solution
We know that, all the sides of a square are always equal.
i.e., AB=BC=CD=DA
in △ACD, AC=44cm,∠D=90∘
Using Pythagoras theorem in △ACD,
AC2=AD2+DC2
⇒ 442=AD2+AD2 [∵DC=AD]
⇒ 2AD2=44×44
⇒r AD2=22×44⇒AD=√22×44
[taking positive square root because length is always positive]
⇒ AD=√2×11×4×11
⇒ AD=22√2cm
So AB=BC=CD=DA=22√2cm
∴ Area of square ABCD=Side×Side=22√2×22√2=968cm2
∴ Area of the red protion=9684=242cm2
[since, area of square is divided into four parts]
Now, area of the green portion=9684=242cm2
In △PCQ, side PC=a=20cm,CQ=b=20cmandPQ=C=14cm
s=a+b+c2=20+20+142=542=27cm
∴ Area of △PCQ=√s(s−a)(s−b)(s−c) [by Heron's formula]
=√27(27−20)(27−20)(27−14)
=√27×7×7×13=√3×3×3×7×7×13
=21√39=21×6.24=131.04cm2
∴ Total area of the green portion=242+131.04)=373.04cm2
Hence, the paper required for each shade to make a kite is red paper 242cm2,yellow paper 484cm2and green paper 373.04cm2.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
How much is the area of the shaded portion in the following figure?
 A11 sq cmB9 sq cmC11 cu cmD9 cu cm
A11 sq cmB9 sq cmC11 cu cmD9 cu cmHow much part of the given square shaped figure is shaded?
 A12B23C35D49
A12B23C35D49Find the area of the shaded region in fig., where ABCD is a square of side 14 cm and four circles are of same radii each.
A128cm2B192cm2C148cm2D168cm2
Which squares should be shaded to make the given figure symmetric?

View SolutionHow many minimum squares must be shaded to make the given figure symmetric?

View SolutionFind the area of the shaded region as shown in figure, where ABCD is square of side 14 cm.
View SolutionFind the area of the shaded region of the given figure, if ABCD is a square of side 14 cm and APD and BPC are semi-circles. ( Takeπ=227)
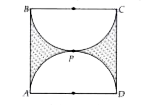
View SolutionFind the area of the shaded region in the given figure, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
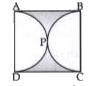
View Solution