|x−1|≤5,|x|≥2
Video Solution
Text Solution
|x−1|≤5
⇒−4≤x−1≤5
⇒−4≤x≤6
⇒x∈[−4,6] ….(i)
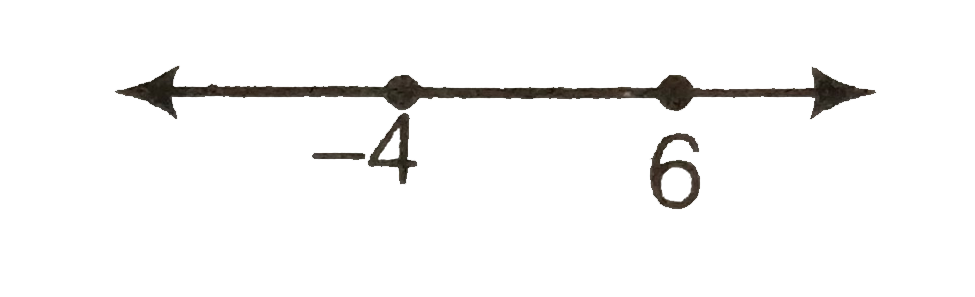
and |x|≥2
and |x|≥2
⇒x≤−2orx≥2
⇒x∈(−∞,−2]∪[2,∞)..(ii)

On combining Eqs. (i) and (ii), we get
x∈(4,−2]∪[2,6]
|
Topper's Solved these Questions
- LINEAR INEQUALITIESBook:NCERT EXEMPLARChapter:LINEAR INEQUALITIESExercise:Long Answer Type Question
Explore 6 Videos
- LINEAR INEQUALITIESBook:NCERT EXEMPLARChapter:LINEAR INEQUALITIESExercise:Objective Type Questions
Explore 14 Videos
- LIMITS AND DERIVATIVES Book:NCERT EXEMPLARChapter:LIMITS AND DERIVATIVES Exercise:FILLERS
Explore 4 Videos
- MATHEMATICAL REASONING Book:NCERT EXEMPLARChapter:MATHEMATICAL REASONING Exercise:OBJECTIVE TYPE QUESTIONS
Explore 21 Videos
Similar Questions
Knowledge Check
The area of the region {(x,y):x2+y2≤5,||x|−|y∣∣≥1 is
A4(π−tan−1(247))−4B5(π−tan−1(247))−4C3(π−tan−1(247))−4D2(π−tan−1(247))−1The set of all values of x satisfying the inequations |x−1|≤5 and |x|≥2, is
A[−4,6]B[−4,−2]C[−4,−2]∪[2,6]D[2,6]Maximize 5x+7y, subject to the constraints 2x+3y≤12,x+y≤5,x≥0andy≥0.
A29B30C28D31
2x+y≥2,x−y≤1,x+2y≤8,x≥0,y≥0.
View Solutiony−2x≤1,x+y≤2,x≥0,y≥0
View Solutionx+y≤5,4x+y≥4,x+5y≥5,x≤4,y≤3.
View Solution3x+4y≤60,x≥2y,x≥1,y≥0
View SolutionBy graphical method, the solutions of linear programming problem maximum Z=3x1+5x2 subject to constraints 3x1+2x2≤18
x1≤4,x2≤6,x1≥0,x2≥0
View Solution