The container shown in figure has two chambers separated by a partition of volumes $V_{1} = 2.0 L$ and $V_{2} = 3.0 L$ . The chambers contain $μ_{1} 4.0$ and $μ_{2} = 5.0$ mole of a gas at pressure $p_{1} = 1.00$ atm and $P_{2} = 2.00$ atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.

Video Solution

Text Solution

Verified by Experts

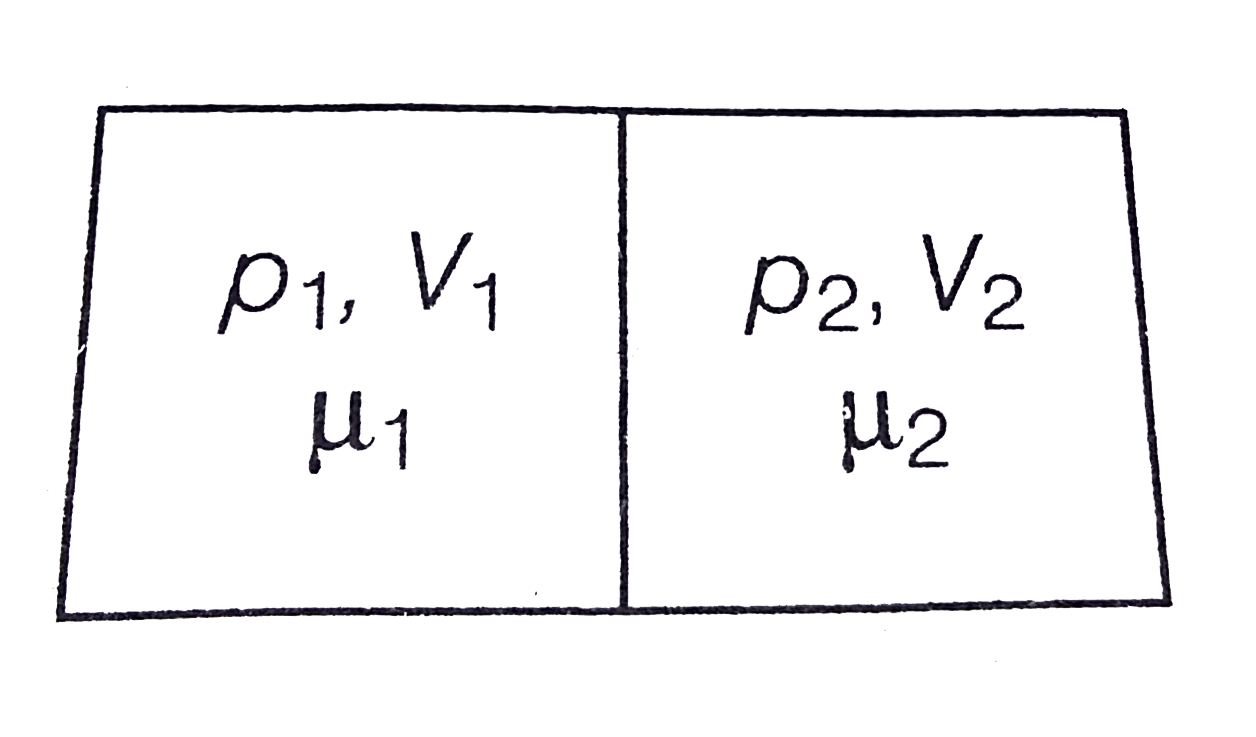
Consider the diagram
$Given V_{1} 2.0 L, V_{2} = 3.0 L$
$μ_{1} 4.0 m o l, μ_{2} = 5.0 m o l$
$p_{1} = 100 a t m p_{2} 2.00 a t m$
For chamber $1, p_{1}, V_{1} = μ_{1} R T_{1}$
For chamber $2, p_{2}, V_{2} = μ_{2} R T_{2}$
When the parition is removed the gasses get mixed wihtout any loss of energy . the mixture now attains a common equilibrium pressure and the total volume of the system is sum of the volume of individual chamber $V_{1}$ and $V_{2}$ .
$So μ = μ_{1} + μ_{2}, V = V_{1} + V_{2}$
From kinetic theory of gasses,
$For l mole p V = \frac{2}{3} μ_{1} E_{1} [\underset{kinetic energy}{E = translational}]$
$For μ_{1} moles p_{1} V_{1} = \frac{2}{3} μ_{2} E_{1}$
$For μ_{2} moles P_{2} V_{2} = \frac{2}{3} μ_{2} E_{2}$
$Total energy is (μ_{1} E_{1} + μ_{2} E_{2}) = \frac{3}{2} (P_{1} V_{1} + P_{2} V_{2})$
$From the above relation p V = \frac{2}{3} E_{Total} = \frac{2}{3} μ E_{per mole}$
$p (V_{1} + V_{2}) = \frac{2}{3} \times \frac{3}{2} (P_{1} V_{1} + p_{2} V_{2})$
$p = \frac{p_{1} V_{1} + p_{2} V_{2}}{V_{1} + V_{2}}$
$= (\frac{1.00 \times 2.0 + 2.00 \times 3.0}{2.0 + 3.0}) atm$
$= \frac{8.0}{5.0} = 1.60 atm$

Updated on:21/07/2023

Class 11 PHYSICS KINETIC THEORY OF GASES

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
An insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume $V_{1}$ and contains ideal gas at pressure $P_{1}$ and temperature $T_{1}$ . The other chambers has volume $V_{2}$ and contains ideal gas at pressure $P_{2}$ and temperature $T_{2}$ . If the partion is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
A $\frac{T_{1} T_{2} (P_{1} V_{1} + P_{2} V_{2})}{P_{1} V_{1} T_{2} + P_{2} V_{2} T_{1}}$
B $\frac{P_{1} V_{1} T_{1} + P_{2} V_{2} T_{2}}{P_{1} V_{1} + P_{2} V_{2}}$
C $\frac{P_{1} V_{1} T_{2} + P_{2} V_{2} T_{1}}{P_{1} V_{1} + P_{2} V_{2}}$
D $\frac{T_{1} T_{2} (P_{1} V_{1} + P_{2} V_{2})}{P_{1} V_{1} T_{1} + P_{2} V_{2} T_{2}}$
Question 2 - Select One
Volume of 0.5 mole of a gas at 1 atm. pressure and $273^{\circ} C$ is
A22.4 litres
B11.2 litres
C44.8 litres
D5.6 litres
Question 3 - Select One
An insulated container of gas has two chambers separated by an insulating partition. One of the chmabers has volume $V_{1}$ and contains ideal gas at pressure $P_{1}$ and temperature $T_{1}$ .The other chamber has volume $V_{2}$ and contains ideal gas at pressure $P_{2}$ and temperature $T_{2}$ . If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
A $(T_{1} T_{2} \frac{P_{1} V_{1} + P_{2} V_{2}}{P_{1} V_{1} T_{2} + P_{2} V_{2} T_{1}}$
B $\frac{P_{1} V_{1} T_{1} + P_{2} V_{2} T_{2}}{P_{1} V_{1} + P_{2} V_{2}}$
C $\frac{P_{1} V_{1} T_{2} + P_{2} V_{2} T_{1}}{P_{1} V_{1} + P_{2} V_{2}}$
D $\frac{T_{1} T_{2} (P_{1} V_{1} + P_{2} V_{2})}{P_{1} V_{1} T_{1} + P_{2} V_{2} T_{2}}$

NCERT EXEMPLAR-KINETIC THEORY-Multiple Choice Questions (MCQs)

Home
Ask Doubt
Profile

Topper's Solved these Questions

Similar Questions

Knowledge Check

Volume of 0.5 mole of a gas at 1 atm. pressure and 273∘C is

NCERT EXEMPLAR-KINETIC THEORY-Multiple Choice Questions (MCQs)

Volume of 0.5 mole of a gas at 1 atm. pressure and $273^{\circ} C$ is